Advances in Applied Mathematics
Vol.05 No.02(2016), Article ID:17554,10
pages
10.12677/AAM.2016.52030
Existence and Multiplicity of Semipositone Discrete Boundary Value Problems
Yunxia Zeng
School of Mathematics and Information, Guangzhou University, Guangzhou Guangdong

Received: Apr. 25th, 2016; accepted: May 10th, 2016; published: May 13th, 2016
Copyright © 2016 by author and Hans Publishers Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



ABSTRACT
By using the Guo-Krasnosel’skii fixed point theorem, a Dirichlet boundary value problem with sign-changing nonlinearity is discussed and some results of existence and multiplicity of positive solutions are established.
Keywords:Positive Solution, Green Function, Fixed Point Theorem, Semipositone Problem

离散半正边值问题正解的存在性及多解性
曾云霞
广州大学数学与信息科学学院,广东 广州

收稿日期:2016年4月25日;录用日期:2016年5月10日;发布日期:2016年5月13日

摘 要
应用Guo-Krasnosel’skii不动点理论,在非线性项为变号函数的情形下,讨论离散Dirichlet问题,建立正解的存在性及多解性结果。
关键词 :正解,Green函数,不动点理论,半正问题

1. 引言
近几十年来,由于差分方程广泛的实际应用背景而日益引起人们的重视,有关差分方程的稳定性、周期解问题及边值问题的研究成果已有大量的文献。差分边值问题正解的存在性作为差分方程领域极其重要的一个研究分支也受到人们的广泛关注。关于这方面的研究,目前比较常用的工具和方法有临界点理论、上下解的方法、不动点方法和分支理论等 [1] - [8] 。在1994年,Anuradha-Shivaji [10] 考虑了下列两点边值问题正解的存在性
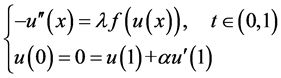
其中,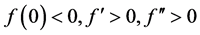 ,并且满足
,并且满足 。Anuradha-Shivaji [13] 把文 [10] 中的研究方法运用到讨论Robin边值问题正解的存在性
。Anuradha-Shivaji [13] 把文 [10] 中的研究方法运用到讨论Robin边值问题正解的存在性

其中, 为参数,
为参数, ,且
,且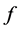 在无穷远处满足超线性增长条件。
在无穷远处满足超线性增长条件。
在1996年,Anuradha在文献 [11] 中讨论了Sturm-Liouville边值问题

正解的存在性。其中,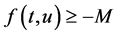 ,即
,即 下有界的,并且在无穷远处满足超线性增长条件,即
下有界的,并且在无穷远处满足超线性增长条件,即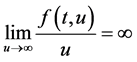 对
对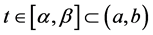 一致成立。
一致成立。
文 [9] 将 [11] 中的研究结果推广到如下离散边值问题

同样要求 有下界,并且在无穷远处满足超线性增长条件。
有下界,并且在无穷远处满足超线性增长条件。
2011年,文 [12] 考虑了如下半正二阶多点边值问题正解的存在性
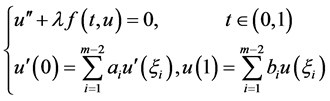
其中, 为正参数,非线性项
为正参数,非线性项 连续且下有界,
连续且下有界, 。此外,还可参见文 [14] - [21] 。
。此外,还可参见文 [14] - [21] 。
最近,文 [22] 讨论了如下Neumann边值问题正解的存在性

文 [22] 将以上文献的条件极大地加以减弱,非线性项 可能最终是非正的甚至是下无界的。
可能最终是非正的甚至是下无界的。
本文,我们研究如下Dirichlet问题
 (1.1)
(1.1)
我们的目的是在非线性项可能下无界的情况下讨论正解的存在性和多解性。为此,我们做如下假设:
(C1): 连续;
连续;
(C2):存在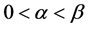 ,使得
,使得 ;
;
(C3):存在函数 ,
, 及
及 ,使得
,使得

本文内容安排如下:在第二部分,我们做一些必要的准备工作,给出一些引理及Guo-Krasnosel’skii不动点定理;在第三部分给出问题(1.1)正解的存在性及多解性结果,并给予证明。
2. 准备工作
引理2.1:若 ,则问题(1.1)没有正解。
,则问题(1.1)没有正解。
证明:假设 ,且问题(1.1)存在正解
,且问题(1.1)存在正解 ,则
,则
 。设
。设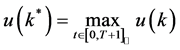 。
。
若 或
或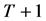 ,则由边界条件
,则由边界条件 知,
知, ,这与
,这与 为正解矛盾。
为正解矛盾。
若 ,则
,则
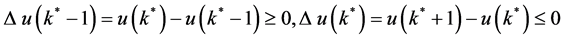
从而, ,这与
,这与 矛盾。
矛盾。
所以,若 ,则问题(1.1)没有正解。
,则问题(1.1)没有正解。
注:由引理2.1知,要研究问题(1.1)正解的存在性,非线性项 不能恒小于0。因此,我们给出了条件(C2)。
不能恒小于0。因此,我们给出了条件(C2)。
记

考虑如下线性Dirichlet边值问题
 (2.1)
(2.1)
引理2.2. 问题(2.1)有唯一解
 (2.2)
(2.2)
其中

 ,且
,且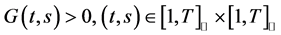 。
。
证明:显然, 对所有
对所有 都成立,则
都成立,则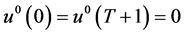 。下面证明
。下面证明 满足方程
满足方程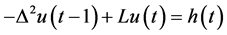 ,即
,即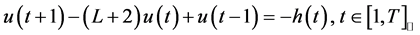 。因为
。因为

我们只需证明 。由于
。由于 为特征方程
为特征方程 的根,对所有
的根,对所有 及
及 ,有
,有

同理,我们可以得到 。考虑
。考虑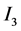 ,再次利用方程
,再次利用方程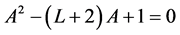 ,有
,有

故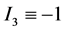 。
。
易知(2.1)对应的齐次方程只有零解,所以方程(2.1)有唯一解 。又由
。又由 知
知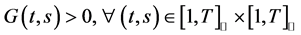 。
。
记

易知 。
。
引理2.3. 。
。
证明: 注意到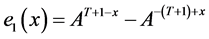 在
在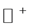 上单调递减,
上单调递减, 在
在 上单调递增。当
上单调递增。当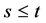 时,有
时,有
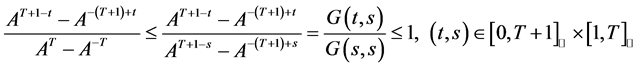 。
。
当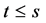 时,有
时,有
 。
。
因此, 。
。
引理2.4: 。这里
。这里
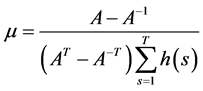
证明:当 时,一方面,
时,一方面,

另一方面,

因此,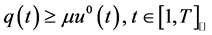 。
。
定义
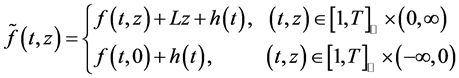
我们考虑边值问题
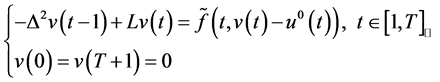 (2.3)
(2.3)
容易得到下列结果:
引理2.5: 是边值问题(1.1)的解当且仅当
是边值问题(1.1)的解当且仅当 是边值条件问题(2.3)的解,且
是边值条件问题(2.3)的解,且 。
。
下面我们给出本文的主要工具:Guo-Krasnosel’skii不动点定理。
引理2.6:设 是实Banach空间,
是实Banach空间, 是
是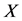 的一个锥,
的一个锥, 是
是 中有界开集,
中有界开集, 全连续算子。如果下列条件之一满足
全连续算子。如果下列条件之一满足
(i) 
(ii) 
则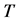 在
在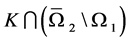 中必有不动点。
中必有不动点。
3. 主要结果
本节我们介绍本文的主要结果及其证明。记

定义
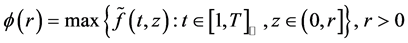

定理3.1:设(C1)~(C3)成立,如果存在 ,使得
,使得
(C4) ,
,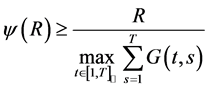 ,
,
则问题(1.1)至少存在一个正解。进一步,若
(C5)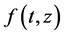 最终非正,即
最终非正,即 ,当
,当 时,对所有
时,对所有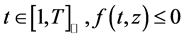 ,
,
(C6) ,
,
则问题(1.1)至少存在两个正解。
证明:假设(C4)成立,我们先利用引理2.6证明方程(2.3)至少存在一个正解 。
。
取空间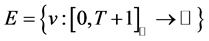 ,其中范数
,其中范数 。定义
。定义
 (3.1)
(3.1)
又由(C1)知, 全连续,定义锥
全连续,定义锥
 (3.2)
(3.2)
由引理2.3, 。接下来,证明
。接下来,证明 在
在 内有不动点,它是(2.3)的一个正解。
内有不动点,它是(2.3)的一个正解。
令
 (3.3)
(3.3)
对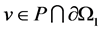 ,我们有
,我们有 。则
。则
 。又
。又 。因此由(C4)得到
。因此由(C4)得到

即 。
。
现在证明 对
对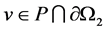 成立。设
成立。设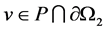 ,由引理2.4,对
,由引理2.4,对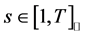 ,
,
 (3.4)
(3.4)
从而 。再通过(C4),对
。再通过(C4),对 ,
,

所以,由引理2.6知,算子 有一个不动点
有一个不动点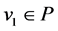 ,满足
,满足 ,也就是说,
,也就是说, 为边值问题 (2.3)的正解。又
为边值问题 (2.3)的正解。又 ,故
,故 是边值问题(1.1)的一个正解。
是边值问题(1.1)的一个正解。
现在,若(C5) (C6)都成立,我们证明问题(1.1)存在另外一个正解 。由(C5)得到
。由(C5)得到

对 一致成立。因此存在
一致成立。因此存在 ,对
,对 成立。
成立。
取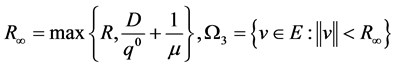 。对任意的
。对任意的 ,类似于(3.4),我们有
,类似于(3.4),我们有
 (3.5)
(3.5)
从而,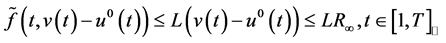 。因此,由条件(C6),对任意的
。因此,由条件(C6),对任意的 ,
,
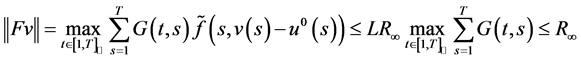
故 ,
, 。因此,由引理2.6知,算子
。因此,由引理2.6知,算子 有一个不动点
有一个不动点 ,且满足
,且满足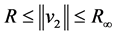 。所以
。所以 是满足边值问题(1.1)的另外一个正解。
是满足边值问题(1.1)的另外一个正解。
推论3.2:设(C1)~(C3)成立,且存在 ,使得(C4)成立。如果
,使得(C4)成立。如果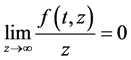 对
对 一致成立,且
一致成立,且 ,则边值问题(1.1)至少存在两个正解。
,则边值问题(1.1)至少存在两个正解。
证明:取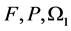 和
和 分别是由(3.1)(3.2)和(3.3)给出的。由定理3.1的证明,
分别是由(3.1)(3.2)和(3.3)给出的。由定理3.1的证明, 有一个不动点
有一个不动点 ,且
,且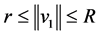 ,故
,故 为边值问题(1.1)的一个正解。现在,我们证明
为边值问题(1.1)的一个正解。现在,我们证明 有另外一个不动点
有另外一个不动点 。
。
取 足够小使得
足够小使得 。从
。从 对
对 一致成立,我们可以找出一个
一致成立,我们可以找出一个 当
当 ,有
,有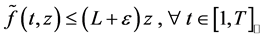 。选取
。选取 和
和 。当
。当 ,容易得出(3.5)成立。从而
,容易得出(3.5)成立。从而
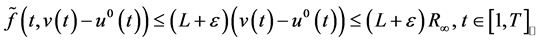 。
。
因此,对 ,
,
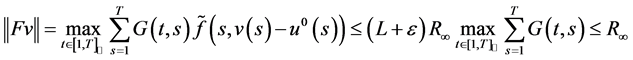
即 ,
,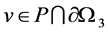 。
。
因此,由引理2.6知,算子 有一个不动点
有一个不动点 ,且满足
,且满足 。所以
。所以 是满足边值问题(1.1)的另外一个正解。
是满足边值问题(1.1)的另外一个正解。
定理3.3:设(C1)-(C3)成立。如果存在 ,使得
,使得
(C4)*: ,
,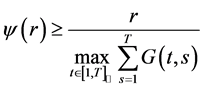 ,
,
则差分方程边值问题(1.1)至少存在一个正解。更进一步,若同时满足
(C5)*: 最终非负,即
最终非负,即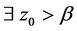 ,当
,当 时,对所有
时,对所有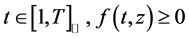 ,
,
(C6)*: ,
,
则差分方程 (1.1)至少存在两个正解。
证明:取 和
和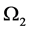 分别是由(3.1)(3.2)和(3.3)给出的。类似于定理3.1的证明,若(C4)*成立,则
分别是由(3.1)(3.2)和(3.3)给出的。类似于定理3.1的证明,若(C4)*成立,则 和
和 。所以
。所以 有一不动点
有一不动点 满足
满足 ,它为问题(2.5)的正解并且满足
,它为问题(2.5)的正解并且满足 。因此,由引理2.5知
。因此,由引理2.5知 为(1.1)的一个正解。
为(1.1)的一个正解。
若条件(C5)*和(C6)*同时成立,对(C5)*,我们知道
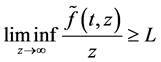
对 一致成立。故存在
一致成立。故存在 ,当
,当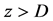 时,有
时,有 。选取
。选取 及
及 。对任意的
。对任意的 ,我们有
,我们有

从而, 。结合条件(C6)*,对满足
。结合条件(C6)*,对满足 ,有
,有

即,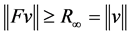 ,
, 。所以
。所以 有另外一个不动点
有另外一个不动点 使得
使得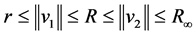 。因此,边值问题(1.1)存在两个不同的正解
。因此,边值问题(1.1)存在两个不同的正解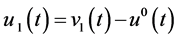 及
及 。
。
下面推论可直接由定理3.3得到。
推论3.4:设条件(C1)-(C3)成立,且存在 ,使得(C4)*成立。如果
,使得(C4)*成立。如果 对
对 一致成立,且
一致成立,且 。则边值问题(1.1)至少存在两个正解。
。则边值问题(1.1)至少存在两个正解。
文章引用
曾云霞. 离散半正边值问题正解的存在性及多解性
Existence and Multiplicity of Semipositone Discrete Boundary Value Problems[J]. 应用数学进展, 2016, 05(02): 232-241. http://dx.doi.org/10.12677/AAM.2016.52030
参考文献 (References)
- 1. Berger, H. (2008) Existence of Nontrivial Solutions of a Two-Point Boundary Value Problem for a 2nth-Order Nonlinear Difference Equation. Advances in Dynamical Systems and Applications, 3, 131-146.
- 2. Anderson, D. (2003) Discrete Third-Order Three-Point Right-Focal Boundary Value Problems. Computers & Mathematics with Applications, 45, 861-871. http://dx.doi.org/10.1016/S0898-1221(03)80157-8
- 3. Anderson, D. and Avery, R. (2001) Multiple Positive Solutions to a Third-Order Discrete Focal Boundary Value Problem. Computers & Mathematics with Applications, 42, 333-340. http://dx.doi.org/10.1016/S0898-1221(01)00158-4
- 4. Aykut, N. (2004) Existence of Positive Solutions for Boundary Value Problems of Second Order Functional Difference Equations. Computers & Ma-thematics with Applications, 48, 517-527. http://dx.doi.org/10.1016/j.camwa.2003.10.007
- 5. Bai, D. (2013) A Global Result for Discrete ϕ-Laplacian Ei-genvalue Problems. Advances in Difference Equations, 264, 10 p.
- 6. Bai, D. and Xu, Y. (2007) Nontrivial Solutions of Boundary Value Problems of Second Order Difference Equations. Journal of Mathematical Analysis and Applications, 326, 297-302. http://dx.doi.org/10.1016/j.jmaa.2006.02.091
- 7. Bai, D. and Xu, X. (2013) Existence and Multiplicity of Difference ϕ-Laplacian Boundary Value Problems. Advances in Difference Equations, 267, 13 p.
- 8. Ji, D. and Ge, W. (2008) Existence of Multiple Positive Solutions for Sturm-Liouville-Like Four-Point Boundary Value Problem with p-Laplacian. Nonlinear Analysis: Theory, Methods & Applications, 68, 2638-2646. http://dx.doi.org/10.1016/j.na.2007.02.010
- 9. Bai, D. and Xu, Y. (2008) Positive Solutions for Semipositone BVPs of Second-Order Difference Equations. Indian Journal of Pure and Applied Mathematics, 39, 59-68.
- 10. Anuradha, V. and Shivaji, R. (1994) A Quadrature Method for Classes of Multi-Parameter Two Point Boundary Value Problems. Applicable Analysis, 54, 263-281. http://dx.doi.org/10.1080/00036819408840282
- 11. Anuradha, A. and Shivaji, R. (1996) Existence Results for Superlinear Semipositone BVP’s. Proceedings of the American Mathematical Society, 124, 757-763. http://dx.doi.org/10.1090/S0002-9939-96-03256-X
- 12. Feng, H. and Bai, D. (2011) Existence of Positive Solu-tions for Semipositone Multi-Point Boundary Value Problem. Mathematical and Computer Modelling, 54, 2287-2292. http://dx.doi.org/10.1016/j.mcm.2011.05.037
- 13. Anuradha, V. and Shivaji, R. (1999) Positive Solutions for a Class of Nonlinear Boundary Value Problems with Neumann-Robin Boundary Conditions. Journal of Mathematical Analysis and Applications, 236, 94-124. http://dx.doi.org/10.1006/jmaa.1999.6439
- 14. Bai, D. and Xu, Y. (2005) Existence of Positive Solutions for Boundary Value Problems of Second-Order Delay Differential Equations. Applied Mathematics Letters, 18, 621-630. http://dx.doi.org/10.1016/j.aml.2004.07.022
- 15. Bai, D. and Xu, Y. (2005) Positive Solutions of Second-Order Two-Delay Differential Systems with Twin-Parameter. Nonlinear Analysis, 63, 601-617. http://dx.doi.org/10.1016/j.na.2005.05.021
- 16. Castro, A. and Shivaji, R. (2000) Evolution of Positive Solution Curves in Semipositone Problems with Concave Nonlinearities. Journal of Mathematical Analysis and Applications, 245, 282-293. http://dx.doi.org/10.1006/jmaa.2000.6787
- 17. Hai, D. and Shivaji, R. (1998) Positive Solutions of Quasilinear Boundary Value Problems. Journal of Mathematical Analysis and Applications, 217, 672-686. http://dx.doi.org/10.1006/jmaa.1997.5762
- 18. Hai, D. and Shivaji, R. (2004) An Existence Result on Positive Solutions for a Class of p-Laplacian Systems. Nonlinear Analysis, 56, 1007-1010. http://dx.doi.org/10.1016/j.na.2003.10.024
- 19. Ma, R. (2003) Multiple Positive Solutions for a Semipositone Fourth-Order Boundary Value Problem. Hiroshima Mathematical Journal, 33, 217-227.
- 20. Maya, C. and Shivaji, R. (1999) Multiple Positive Solutions for a Class of Semilinear Elliptic Boundary Value Problems. Nonlinear Analysis, 38, 497-504. http://dx.doi.org/10.1016/S0362-546X(98)00211-9
- 21. Sun, J. and Wei, J. (2008) Existence of Positive Solutions of Positive Solution for Semi-Positone Second-Order Three- Point Boundary-Value Problems. Electronic Journal of Differential Equations, 41, 1-7.
- 22. Bai, D., Henderson, J. and Zeng, Y. (2015) Positive Solutions of Dis-crete Neumann Boundary Value Problems with Sign-Changing Nonlinearities. Boundary Value Problems, 1, 231. http://dx.doi.org/10.1186/s13661-015-0500-8