Advances in Applied Mathematics
Vol.07 No.08(2018), Article ID:26646,10
pages
10.12677/AAM.2018.78127
Solution of Sequential Fractional Differential Equations with Integral Boundary Value Conditions
Zongfu Zhou, Wei Jiang
School of Mathematical Sciences, Anhui University, Hefei Anhui

Received: Aug. 9th, 2018; accepted: Aug. 22nd, 2018; published: Aug. 29th, 2018

ABSTRACT
In this paper, we investigate a class of nonlocal integral boundary value problems of sequential fractional differential equations of order
with . By means of Banach’s contraction mapping principle, nonlinear contraction and Leray-Schauder degree theory, some existence result of solutions are obtained. An illustrative example is given to show the applicability of our results.
. By means of Banach’s contraction mapping principle, nonlinear contraction and Leray-Schauder degree theory, some existence result of solutions are obtained. An illustrative example is given to show the applicability of our results.
Keywords:Fixed Point Theorems, Solution, Integral Boundary Condition, Sequential Fractional Differential Equation

一类具积分边值条件的有序分数阶微分方程解
周宗福,蒋伟
安徽大学数学科学学院,安徽 合肥

收稿日期:2018年8月9日;录用日期:2018年8月22日;发布日期:2018年8月29日

摘 要
本文研究了一类带有非局部积分边值条件的 阶有序分数阶微分方程,其中 。借助于Banach压缩映像原理,非线性压缩和Leray-Schauder度理论,得到该问题解的存在性若干结果,并给出一个例子来说明所得结果的应用性。
关键词 :不动点定理,解,积分边值条件,有序的分数阶微分方程

Copyright © 2018 by authors and Hans Publishers Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/


1. 引言
近年来,数学工作者对分数阶微分方程初值问题和边值问题进行了广泛的研究。一些学者研究了带有多点的,积分的,反周期的,非局部的等类型的边值条件的分数阶微分方程,得到了一些很好的结果 [1] - [13] 。分数阶微分方程边值问题方面还有其他很多研究成果,参见文献 [14] - [20] 。
本文研究带有积分边界条件的有序分数阶微分方程:
(1.1)
(1.2)
其中
是Caputo分数阶导数, ,D是正常导数,
连续,
为正的实常数,
。
,D是正常导数,
连续,
为正的实常数,
。
本文主要目标是讨论边值问题(1.1)~(1.2)解的存在性。
在文献 [21] 中,研究了如下的有序分数阶微分方程的边值问题:
其中
,
为正实数, 为实数。
为实数。
在文献 [22] 中,研究了如下具边值条件的分数阶微分方程:
其中 , 连续, 为常实数。
受文献 [21] 和 [22] 的启发,本文研究边值问题(1.1)~(1.2),运用Banach压缩映像原理,非线性压缩,以及Leray-Schauder度理论,得到边值问题(1.1)~(1.2)解存在的若干结果。
本文的内容安排如下:第二部分给出分数阶微积分的一些定义及有关引理。第三部分给出三个主要结论:第一个结论是根据Banach压缩映像原理得出的,第二个结论是根据非线性压缩得出的,第三个结论是根据Leray-Schauder度理论得出的。第四部分给出实例,说明我们主要结果的应用性。
2. 预备知识
在这部分,我们介绍一些分数阶微积分定义和引理,为后面我们建立主要结果做准备。
定义2.1 函数 的q阶分数阶积分定义为
其中右边是逐点定义在 上。
定义2.2 函数 的q阶Riemann-Liouville分数阶导数定义为
定义2.3 函数 的q阶Caputo分数阶导数定义为
注2.4 (i) 若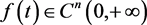 ,则
,则
(ii) 常数的Caputo分数阶导数为零。
引理2.5 [1] 对于 ,分数阶微分方程 的通解为
其中 , , , 表示实数q的整数部分。
引理2.6 [1] 对任意 ,有下式成立:
其中 , , 。
在本文的以下部分,我们假设:
其中 , , , 。
对 ,引入线性方程:
(2.1)
考虑带有边界条件(1.2)的有序分数阶微分方程(2.1),即考虑边值问题(2.1)~(1.2)。
引理2.7 边值问题(2.1)~(1.2)的唯一解为
(2.2)
证 对方程(2.1)两端作用 ,我们得到
也可以写成
上式两端从0到t积分,结合(1.2)中的 ,可得
(2.3)
再由边值条件(1.2)可得
将 , 代入到(2.3),即得到(2.2)。证毕。
3. 主要结果
令 ,定义其中的范数为 ,则E为一个Banach空间。
由引理2.7,我们可将边值问题(1.1)~(1.2)转换成 定义为:
(3.1)
显然,u是边值问题(1.1)~(1.2)的解当且仅当u为算子T的不动点。
首先,通过Banach压缩映像原理来研究边值问题(1.1)~(1.2)解的存在唯一性。
定理3.1 设f连续且满足:
,
其中Lipschitz常数 。若 ,则边值问题(1.1)~(1.2)存在唯一解,其中
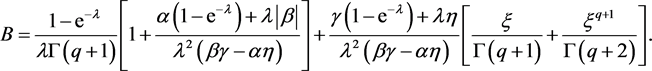
证 第一步. T如(3.1)定义,令
又令 。对 , ,有
于是,得到 。因此 。
第二步,对于
, ,有
,有
即有
。由于
,因此T在 上是压缩的。由压缩映像原理可知,定理的结论成立。证毕。
上是压缩的。由压缩映像原理可知,定理的结论成立。证毕。
接下来我们利用非线性压缩的一个著名定理来分析(1.1)~(1.2)解的存在问题。
定义3.2 令F为一个Banach空间, 为一个映射。如果存在一个单调不减的连续函数 ,使得 ,对任意 ,有 ,且满足:
则称 是非线性压缩算子。
引理3.3 [23] 设F为一个Banach空间, 是非线性压缩算子,则 在F上存在唯一的不动点。
定理3.4 设 连续,且设
其中 是连续的,且正常数
由下式给出:
是连续的,且正常数
由下式给出:
则边值问题(1.1)~(1.2)存在唯一解。
证 令 为(3.1)定义的算子,定义一个单调不减的连续函数 ,
注意到函数 满足 ,且对任意 ,有 。
对任意 ,且任意 ,我们有
故有 ,因此T是非线性压缩算子,从而由引理3.3知,算子T存在唯一不动点,
即边值问题(1.1)~(1.2)存在唯一解。证毕。
现在利用Leray-Schauder度理论,讨论边值问题(1.1)~(1.2)的解的存在性。
定理3.5 设 连续,且存在常数 (这里的B即为定理3.1中的B), ,使得
则边值问题(1.1)~(1.2)至少存在一个解。
证 考虑算子方程 ,下面证明此方程至少存在一个解。
取 ,R将在后面给出。我们将证明 满足条件: 。由Arzela-Ascoli定理易知,T在 上是全连续的。定义一个连续映射 :
通过拓扑度的同伦不变性,可得出:
,其中I是恒等算子。
再由Leray-Schauder度的非零性质可知,至少存在一个 使 ,可见u即为T的不动点。
假设 , ,使得 ,那么对 ,则
从而 ,即有 。
取 ,则 ,有 。因此边值问题(1.1)~(1.2)至少存在一个解。证毕。
4. 举例
例4.1 考虑如下边值问题
(4.1)
其中 。
直接计算易知
取
则
因此定理3.5的条件都满足,所以由定理3.5知边值问题(4.1)至少存在一个解。
致谢
本文得到国家自然科学基金项目(编号:11371027)和安徽省自然科学基金项目(编号:1608085MA12)资助。
文章引用
周宗福,蒋伟. 一类具积分边值条件的有序分数阶微分方程解
Solution of Sequential Fractional Differential Equations with Integral Boundary Value Conditions[J]. 应用数学进展, 2018, 07(08): 1095-1104. https://doi.org/10.12677/AAM.2018.78127
参考文献
- 1. Oldham, K. and Spanier, J. (1974) Fractional Calculus. Academic Press, New York.
- 2. Miller, K. and Ross, B. (1993) An Intro-duction to the Fractional Calculus and Fractional Differential Equations. Wiley, New York.
- 3. Su, X. (2011) Solutions to Boundary Fractional Boundary Value Problem of Fractional Order on Unbounded Domains in a Banach Space. Nonlinear Analysis, 74, 2844-2852. https://doi.org/10.1016/j.na.2011.01.006
- 4. Bai, Z.B. and Sun, W. (2012) Existence and Multiplicity of Positive Solutions for Singular Fractional Boundary Value Problems. Computers & Mathematics with Applications, 63, 1369-1381. https://doi.org/10.1016/j.camwa.2011.12.078
- 5. Agarwal, R.P., O’Regan, D. and Stanek, S. (2012) Positive Solutions for Mixed Problems of Singular Fractional Differential Equations. Mathematische Nachrichten, 285, 27-41. https://doi.org/10.1002/mana.201000043
- 6. Cabada, A. and Wang, G. (2012) Positive Solutions of Nonlinear Fractional Dif-ferential Equations with Integral Boundary Value Conditions. Journal of Mathematical Analysis and Applications, 389, 403-411. https://doi.org/10.1016/j.jmaa.2011.11.065
- 7. Ahmad, B. and Ntouyas, S.K. (2013) A Study of Nonlinear Fractional Differen-tial Equations of Arbitrary Order with Riemann-Liouville Type Multistrip Boundary Conditions. Mathematical Problems in Engi-neering.
- 8. Zhang, L., Wang, G., Ahmad, B. and Agarwal, R.P. (2013) Nonlinear Fractional Integro-Differcntial Equations on Unbounded Domains in a Banach Space. Journal of Computational and Applied Mathematics, 249, 51-56. https://doi.org/10.1016/j.cam.2013.02.010
- 9. Ahmad, B. and Ntouyas, S.K. (2013) Existence Results for Higher Order Frac-tional Differential Inclusions with Multistrip Fractional Integral Boundary Conditions. Electronic Journal of Qualitative Theory of Differential Equations, 20, 19.
- 10. O’Regan, D. and Stanek, S. (2013) Fractional Boundary Value Problems with Singularities in Space Variables. Nonlinear Dynamics, 71, 641-652. https://doi.org/10.1007/s11071-012-0443-x
- 11. Graef, J.R., Kong, L. and Wang, M. (2014) Existence and Uniqueness of Solutions for a Fractional Boundary Value Problem on a Graph. Fractional Calculus and Applied Analysis, 17, 499-510. https://doi.org/10.2478/s13540-014-0182-4
- 12. Wang, G., Liu, S. and Zhang, L. (2014) Eigenvalue Problem for Nonlinear Fractional Differential Equations with Integral Boundary Conditions. Abstract and Applied Analysis. https://doi.org/10.1155/2014/142391
- 13. Ahmad, B. and Agarwal, R.P. (2014) Some New Versions of Fractional Boundary Value Problems with Slit-Strips Conditions. Boundary Value Problems, 175. https://doi.org/10.1186/s13661-014-0175-6
- 14. Podlubny, I. (1999) Fractional Differential Equations. Academic Press, San Diego.
- 15. Kilbas, A.A., Srivastava, H.M. and Trujillo, J.J. (2006) Theory and Applications of Fractional Differential Equations. North-Holland Mathematics Studies, Vol. 204, Elsevier Science B. V, Amsterdam.
- 16. Ahmad, B. and Nieto, J.J. (2009) Existence of Solutions for Nonlocal Boundary Value Problems of Higher Order Nonlinear Fractional Differential Equations. Abstract and Applied Analysis, 2009, Article ID: 494720. https://doi.org/10.1155/2009/494720
- 17. Ahmad, B. and Nieto, J.J. (2009) Existence Results for a Coupled System of Nonlinear Fractional Differential Equations with Three-Point Boundary Conditions. Computers & Mathematics with Applications, 58, 1838-1843. https://doi.org/10.1016/j.camwa.2009.07.091
- 18. Nieto, J.J. (2010) Maximum Principles for Fractional Differential Equations Derived from Mittag-Leffler Functions. Applied Mathematics Letters, 23, 1248-1251. https://doi.org/10.1016/j.aml.2010.06.007
- 19. Baleanu, D., Mustafa, O.G. and Agarwal, R.P. (2010) An Existence Result for a Superlinear Fractional Differential Equation. Applied Mathematics Letters, 23, 1129-1132. https://doi.org/10.1016/j.aml.2010.04.049
- 20. Zhang, S. (2010) Positive Solutions to Singular Boundary Value Problem for Nonlinear Fractional Differential Equation. Computers & Mathematics with Applications, 59, 1300-1309. https://doi.org/10.1016/j.camwa.2009.06.034
- 21. Ahmad, B. and Nieto, J.J. (2012) Sequential Fractional Differential Equations with Three-Point Boundary Conditions. Computers and Mathematics with Applications, 64, 3046-3052. https://doi.org/10.1016/j.camwa.2012.02.036
- 22. Ahmad, B. and Ntouyas, S.K. (2016) Some Fractional-Order One-Dimensional Semi-Linear Problems under Nonlocal Integral Boundary Conditions. Racsam, 110, 159-172. https://doi.org/10.1007/s13398-015-0228-4
- 23. Boy, D.W. and Wong, J.S.W. (1969) On Nonlinear Contractions. Proceedings of the American Mathematical Society, 20, 458-464. https://doi.org/10.1090/S0002-9939-1969-0239559-9