Advances in Condensed Matter Physics
Vol.07 No.01(2018), Article ID:23871,5
pages
10.12677/CMP.2018.71006
Topological Flat Band and Quantum Hall Effect in the Triangular Lattice
Le Gong, Hao Wei*, Xiangru Tao
School of Physics Science and Technology, Xinjiang University, Urumqi Xinjiang

Received: Feb. 2nd, 2018; accepted: Feb. 18th, 2018; published: Feb. 27th, 2018

ABSTRACT
We use the tight-bonding model to study the topological flat band in a triangular lattice. Under a staggered magnetic field with zero total flux through the unit cell, the topological nearly flat band with a large band gap and nonzero Chern number can be obtained by manipulating the next nearest neighbor hopping and staggered flux. This topological flat band carries a high Chern number C = 2, which can yield an integer quantum Hall effect.
Keywords:Topological Flat Band, Staggered Flux, Quantum Hall Effect
三角晶格中的拓扑平带和量子霍尔效应
龚乐,魏浩*,陶相如
新疆大学物理科学与技术学院,新疆 乌鲁木齐

收稿日期:2018年2月2日;录用日期:2018年2月18日;发布日期:2018年2月27日

摘 要
本文采用紧束缚模型研究三角晶格中的拓扑平带。在模型中引入净磁场为零的交错磁场,通过改变格点之间的次近邻跃迁强度和交错磁通的大小能够得到具有大带隙且拓扑非平庸的近平带结构。此拓扑平带的高陈数C = 2,进而可以产生稳定的整数量子霍尔效应。
关键词 :拓扑平带,交错磁通,量子霍尔效应

Copyright © 2018 by authors and Hans Publishers Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/


1. 引言
量子霍尔效应自发现以来引起了人们广泛的关注 [1] [2] 。之后,人们开始对无磁场格点模型的整数和分数量子霍尔效应做了大量的研究工作。Haldane模型 [3] 就是其中最著名的一个例子,它是建立在半满填充的蜂窝状晶格上的,由于模型中引入了净磁场为零的非均匀磁场,整数量子霍尔效应可以稳定存在,而且霍尔电导等于一个拓扑不变的陈数。从拓扑学的观点看,Haldane模型可以视为广义的整数量子霍尔效应,但是这些体系的单粒子能带都是色散的,它们不支持分数激发,故在这些体系中不能实现分数量子霍尔效应。然而,如何在格点模型上实现分数量子态,现在还不清楚。要在格点模型上实现分数拓扑态的关键在于实现拓扑非平庸的近平带结构。在实际材料中,严格的平带(带宽为零)是非物理的,因此我们可以放宽限制,只要求带宽远小于带隙宽度即可。
最近,研究者提出了一系列的拓扑平带格点模型 [4] - [14] ,有望克服上述困难,从而实现分数量子霍尔效应。在这些格点模型中,通过调节短程跃迁参数、自旋轨道耦合强度或者交错磁通,可以使能带宽度变小甚至接近于平带。根据接近于平的能带与朗道能级之间的相似性可以猜测 [4] :在这些平带模型中,考虑到排斥相互作用,分数量子霍尔效应(或者分数拓扑绝缘体)可以稳定存在。
本文中我们将详细地研究不考虑相互作用的二维三角晶格模型,通过调节次近邻跃迁强度和交错磁通找到拓扑非平庸的平带结构,从而实现整数量子霍尔效应。在本模型中,由于引入了净磁场为零的非均匀磁场,整数量子霍尔效应可以稳定存在,而且霍尔电导等于一个拓扑不变的陈数。另外,在通常的磁场作用下的连续模型中,朗道能级的陈数一般为1,而在本系统中,还可以实现高陈数,即C = 2的拓扑平带。
2. 模型和方法
二维三角晶格模型如图1所示,其紧束缚模型哈密顿量为 [15] :
(1)
其中 和 分别表示最近邻和次近邻格点对, 表示第 个格点上的消灭和产生算符,最近邻和次近邻格点上的磁通所产生的相位沿着黑色和红色箭头方向分别为 和 , 和 分别表示沿着实线(虚线)方向的最近邻和次近邻跃迁强度,如图1所示。可以通过久保公式计算霍尔电导 [16] :
(2)
其中 是费米能, 是系统的面积, 和 是本征态 和 所对应的本征能量, 为
速度算符。当费米能级 处于能隙之间时,霍尔电导表示为 ,其中 是第 个

Figure 1. The three-band triangular-lattice model, which contains three independent sites (A, B, C)
图1. 三角晶格示意图,每个原胞内含有3个原子,分别用A,B,C标记
填充的子能级陈数 [16] 。
3. 结果与讨论
周期性边界条件下,对哈密顿量(1)进行傅里叶变换后可以得到动量空间的系统哈密顿量,再对动量空间中的哈密顿量进行数值对角化,就可以得到在不同参数下的本征矢量和本征值谱,进而计算出各能带的陈数。
计算结果表明,在不同参数条件下三角晶格的能带中都含有三个能带。分别调节交错磁通和近邻跃迁强度,即调节交错磁通 从0增加到 ,同时使次近邻跃迁强度 从 增加到 ,可以得到 和 的相图,如图2(a)和图2(b)所示。从图中容易得出,当交错磁通 时,带隙与能带的带宽比值出现最大。如图2(c)和图2(d)所示,得到了带隙与带宽的比值随次近邻跃迁强度的变化曲线。从图中可以发现,当交错磁通和次近邻跃迁取 和 时,带隙与最低能带的带宽比值最大, ;而当 和 时,带隙与中间能带的带宽比值最大, ,其中 、 、 、 分别表示中间能带和最低能带间的带隙、最高能带和中间能带间的带隙、最低能带的带宽、中间能带的带宽。此时,能带结构如图3(a)和图3(b)所示,可以看出,最低能带带宽较小,最高能带的带宽虽相对较大,一定程度上仍可认为是属于近平带结构。
由此可以得到,交错磁通 时,能带中出现了平带结构。通过计算可知此时的能带陈数不等于零,分别为 , ,因此能带是拓扑非平庸的,这表明通过调控交错磁通可以得到具有陈数不等于零的拓扑非平庸能带。因此,在接下来的计算过程中选取交错磁通为 ,然后通过调节次近邻跃迁强度,去寻找拓扑非平庸的近平带结构。考虑到粒子的激发是从低能级向高能级跃迁,所以期望较低能级会具有拓扑平带结构。
如图3(a)和图3(b)所示,从能带和陈数的数值计算结果可知,当 和 以及 和 时,带隙与带宽比值最大,而此时各能级的陈数分别为 , , ,这正是我们所寻找的拓扑近平带结构。如图3(c)所示,通计算系统的霍尔电导发现,带隙间有量子化的霍尔电导,电导的平台值分别为 ,和 ,这对应着整数量子霍尔态。因此可以在拓扑平带结构中实现零磁场的整数量子霍尔效应。由于该拓扑平带和二维朗道能级的相似性,在此系统中加上相互作用会有可能实现无磁场的分数激发,从而实现分数量子霍尔态。
4. 结论
本文采用紧束缚模型研究了二维三角晶格在次近邻跃迁强度和交错磁通两个参数调控下的能带结构。

Figure 2. (a) and (b) The flatness ratio of the band gap to the bandwidth versus magnetic flux and next nearest neighbor hopping; (c) and (d) The flatness ratio of the band gap to the bandwidth versus next nearest neighbor hopping at a fixed magnetic flux
图2. (a)和(b)带隙与带宽的比值随次交错磁通和近邻跃迁强度变化关系;(c)和(d)在给定交错磁通下,带隙与带宽的比值随次近邻跃迁强度变化曲线
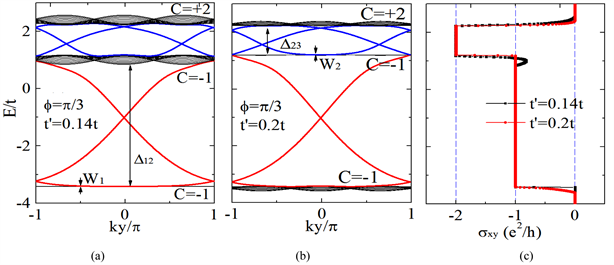
Figure 3. (a) and (b) Energy dispersion of nanoribbon in triangular lattice; (c) The Hall conductance
图3. (a)和(b) 三角晶格纳米带的能带结构;(c) 霍尔电导
通过调节这两个参数,我们得到了拓扑非平庸的近平带结构,此时能带具有高陈数C = 2,系统中可以存在稳定的整数量子霍尔效应,对应的霍尔电导台阶为 。然而,当进一步考虑到粒子间相互作用时,系统中有望实现分数激发,进而出现分数量子霍尔效应。
基金项目
本项目得到了新疆大学大学生创新训练计划项目(201710755074)的支持。
文章引用
龚乐,魏浩,陶相如. 三角晶格中的拓扑平带和量子霍尔效应
Topological Flat Band and Quantum Hall Effect in the Triangular Lattice[J]. 凝聚态物理学进展, 2018, 07(01): 43-47. http://dx.doi.org/10.12677/CMP.2018.71006
参考文献 (References)
- 1. Klitzing, K.V., Dorda, G. and Popper, M. (1980) New Method for High-Accuracy Determination of the Fine-Structure Constant Based on Quantized Hall Resistance. Physical Review Letters, 45, 494-497. https://doi.org/10.1103/PhysRevLett.45.494
- 2. Tsui, D.C., Stormer, H.L. and Gossard, A.C. (1982) Two-Dimensional Magnetotransport in the Extreme Quantum Limit. Physical Review Letters, 48, 1559-1562. https://doi.org/10.1103/PhysRevLett.48.1559
- 3. Haldane, F.D.M. (1988) Model for a Quantum Hall Effect without Landau Levels: Condensed-Matter Realization of the “Parity Anomaly”. Physical Review Letters, 61, 2015. https://doi.org/10.1103/PhysRevLett.61.2015
- 4. Sheng, D.N., Gu, Z.C., Sun, K., et al. (2011) Fractional Quantum Hall Effect in the Absence of Landau Levels. Nature Communications, 2, Article No. 389. https://doi.org/10.1038/ncomms1380
- 5. Tang, E., Mei, J.W. and Wen, X.G. (2011) High-Temperature Fractional Quantum Hall States. Physical Review Letters, 106, Article ID: 236802. https://doi.org/10.1103/PhysRevLett.106.236802
- 6. Sun, K., Gu, Z.C., Katsura, H., et al. (2011) Nearly Flatbands with Nontrivial Topology. Physical Review Letters, 106, Article ID: 236803. https://doi.org/10.1103/PhysRevLett.106.236803
- 7. Neupert, T., Santos, L., Chamon, C., et al. (2011) Fractional Quantum Hall States at Zero Magnetic Field. Physical Review Letters, 106, Article ID: 236804. https://doi.org/10.1103/PhysRevLett.106.236804
- 8. Wang, Y.F., Gu, Z.C., Gong, C.D., et al. (2011) Fractional Quantum Hall Effect of Hard-Core Bosons in Topological Flat Bands. Physical Review Letters, 107, Article ID: 146803. https://doi.org/10.1103/PhysRevLett.107.146803
- 9. Qi, X.L. (2011) Generic Wavefunction Description of Fractional Quan-tum Anomalous Hall States and Fractional Topological Insulators. Physical Review Letters, 107, Article ID: 126803. https://doi.org/10.1103/PhysRevLett.107.126803
- 10. Liu, X.P., Chen, W.C., Wang, Y.F., et al. (2013) Topological Quantum Phase Transitions on the Kagome and Square- Octagon Lattices. Journal of Physics: Condensed Matter, 25, Article ID: 305602. https://doi.org/10.1088/0953-8984/25/30/305602
- 11. He, Y.-C., Sheng, D. N. and Chen, Y. (2014) Chiral Spin Liquid in a Frustrated Anisotropic Kagome Heisenberg Model. Physical Review Letters, 112, Article ID: 137202. https://doi.org/10.1103/PhysRevLett.112.137202
- 12. Simon, S.H., Harper, F. and Read, N. (2015) Fractional Chern Insulators in Bands with Zero Berry Curvature. Physical Review B, 92, Article ID: 195104. https://doi.org/10.1103/PhysRevB.92.195104
- 13. Zeng, T.S., Zhu, W. and Sheng, D.N. (2016) Bosonic Integer Quantum Hall States in Topological Bands with Chern Number Two. Physical Review B, 93, Article ID: 195121. https://doi.org/10.1103/PhysRevB.93.195121
- 14. Du, L., Zhou, X.T. and Fiete, G.A. (2017) Quadratic Band Touching Points and Flat Bands in Two-Dimensional Topological Floquet Systems. Physical Review B, 95, Article ID: 035136. https://doi.org/10.1103/PhysRevB.95.035136
- 15. Wang, Y.F., Yao, H., Gu, Z.C., et al. (2012) Fractional Quantum Hall Effect in Topological Flat Bands with Chern Number Two. Physical Review B, 86, Article ID: 201101(R). https://doi.org/10.1103/PhysRevB.86.201101
- 16. Thouless, D.J., Kohmoto, M., Nightingale, M.P., et al. (1982) Quantized Hall Conductance in a Two-Dimensional Periodic Potential. Physical Review Letters, 49, 405-408. https://doi.org/10.1103/PhysRevLett.49.405