Pure Mathematics
Vol.4 No.05(2014), Article ID:14031,9 pages
DOI:10.12677/PM.2014.45023
 -Order of Solutions of Linear Differential Equations
in the Unit Disc
-Order of Solutions of Linear Differential Equations
in the Unit Disc
Institute of Mathematics and Information Science, Jiangxi Normal University, Nanchang
Email: gongpan12@163.com
Copyright © 2014 by authors and Hans Publishers Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received: Jul. 10th, 2014; revised: Aug. 6th, 2014; accepted: Aug. 15th, 2014
ABSTRACT
In this paper, we investigate the
 -order of solution of second-order linear differential
equation
-order of solution of second-order linear differential
equation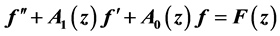 , where
, where ,
,
 and
and
 are analytic functions in the unit disc. We obtain several theorems about the growth
and oscillation of solutions of differential equations.
are analytic functions in the unit disc. We obtain several theorems about the growth
and oscillation of solutions of differential equations.
Keywords:Differential Equations,
 -Order, Unit Disc
-Order, Unit Disc

单位圆内线性微分方程解的 级
级
龚 攀,肖丽鹏
江西师范大学数学与信息科学学院,南昌
Email: gongpan12@163.com
收稿日期:2014年7月10日;修回日期:2014年8月6日;录用日期:2014年8月15日

摘 要
主要研究单位圆内二阶线性微分方程 解的
解的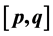 级,其中
级,其中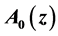 ,
, 和
和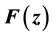 是单位圆内解析函数。我们将得到一些微分方程解的复振荡结论。
是单位圆内解析函数。我们将得到一些微分方程解的复振荡结论。
关键词
微分方程,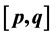 级,单位圆
级,单位圆

1. 引言
假定读者熟悉亚纯函数的Nevanlinna理论在复平面和单位圆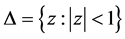 (见文[1] -[5] )中的应用,并用
(见文[1] -[5] )中的应用,并用 表示单位圆上函数
表示单位圆上函数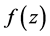 的增长级。最近,很多学者用Nevanlinna理论研究了单位圆内线性微分方程解的增长性,并且得到了很多结论(见文[6]
-[12] )。在文献[13] [14] 中,Juneja,Kapoor和Bajpai研究了整函数
的增长级。最近,很多学者用Nevanlinna理论研究了单位圆内线性微分方程解的增长性,并且得到了很多结论(见文[6]
-[12] )。在文献[13] [14] 中,Juneja,Kapoor和Bajpai研究了整函数 级的性质并且得到了相关的结论,应用
级的性质并且得到了相关的结论,应用 级概念来研究微分方程解的性质见参考文献[15] [16] 等。
级概念来研究微分方程解的性质见参考文献[15] [16] 等。
我们给出一些关于单位圆内解析函数和亚纯函数的迭代级和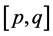 级的相关定义。对于
级的相关定义。对于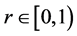 ,定义
,定义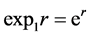 和
和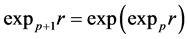 ,
,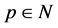 。同理,定义
。同理,定义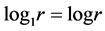 和
和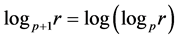 ,
,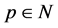 。进一步,我们记
。进一步,我们记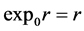 ,
,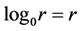 ,
,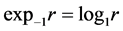 ,
,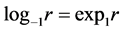 。
。
定义A[8] :定义单位圆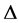 内亚纯函数
内亚纯函数 的迭代
的迭代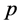 级为
级为
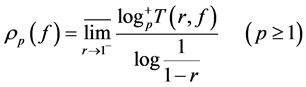 其中
其中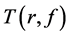 为
为 的特征函数。
的特征函数。
对于单位圆内 内解析函数
内解析函数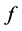 ,我们也定义为
,我们也定义为
 其中
其中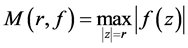 。
。
注1.1:根据M. Tsuji文献[5] ,如果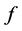 是单位圆内
是单位圆内 内解析函数,则
内解析函数,则
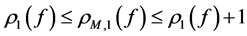 .
.
根据文献[3] 命题2.2.2,我们有
 .
.
定义B[9] [17] :定义单位圆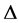 内亚纯函数
内亚纯函数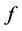 的迭代
的迭代 级零点收敛指数为
级零点收敛指数为
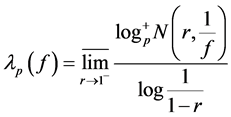 其中
其中 是亚纯函数
是亚纯函数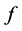 在
在 的零点个数。类似的,定义
的零点个数。类似的,定义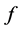 不同迭代
不同迭代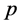 级零点收敛指数为
级零点收敛指数为
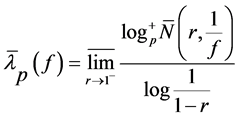 其中
其中 是亚纯函数
是亚纯函数 在
在 不同零点个数。
不同零点个数。
定义C[16] :假设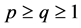 是整数,
是整数, 是单位圆
是单位圆 内亚纯函数,定义
内亚纯函数,定义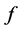 的
的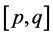 级为
级为
 .
.
对于单位圆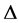 内解析函数
内解析函数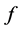 ,我们也定义
,我们也定义
 .
.
注1.2[16] :对于任意的 ,我们有
,我们有
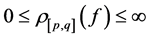 。根据定义C,我们有
。根据定义C,我们有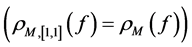
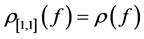 和
和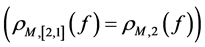
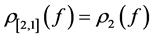 。
。
关于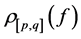 和
和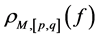 之间的关系,我们有以下结论:
之间的关系,我们有以下结论:
命题1.1[16] :假设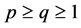 是整数,
是整数, 是单位圆
是单位圆 内具有
内具有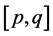 级的解析函数
级的解析函数
(1) 如果 ,则
,则
 .
.
(2) 如果 ,则
,则
 .
.
定义D[16] :假设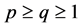 是整数,
是整数,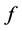 是单位圆
是单位圆 内亚纯函数,定义
内亚纯函数,定义 的
的 级零点收敛指数为
级零点收敛指数为
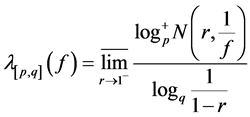 .
.
类似的,定义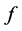 的
的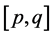 级不同零点收敛指数为
级不同零点收敛指数为
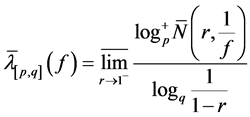 .
.
关于二阶微分方程
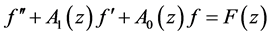 (1.1)
(1.1)
B. Belaïdi和Latreuch在文献[18] [19] 中研究了方程解的微分多项式的增长性和迭代级,为了陈述他们的结果,我们需要以下记号。
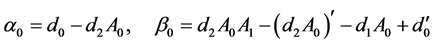 , (1.2)
, (1.2)
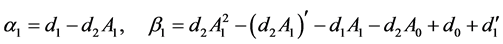 , (1.3)
, (1.3)
 , (1.4)
, (1.4)
其中 ,
, ,
, 是
是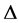 内解析的函数。
内解析的函数。
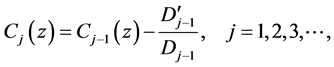 (1.5)
(1.5)
 (1.6)
(1.6)
和
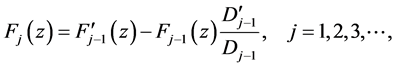 (1.7)
(1.7)
其中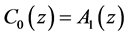 ,
,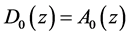 和
和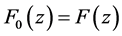 。
。
定理A[18] :假设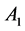 ,
,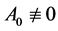 ,
, 是
是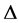 内增长级有限的解析函数,
内增长级有限的解析函数,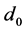 ,
,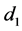 ,
, 也是
也是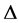 内增长级有限的解析函数,其中
内增长级有限的解析函数,其中 ,
,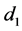 ,
,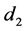 至少有一个不等于零,而且有
至少有一个不等于零,而且有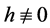 ,
,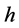 的定义见(1.4)。如果
的定义见(1.4)。如果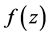 是方程(1.1)的有穷级解并且满足
是方程(1.1)的有穷级解并且满足
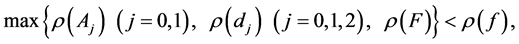
则微分多项式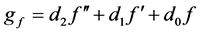 满足
满足
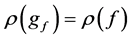 .
.
定理B[19] :假设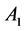 ,
,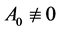 ,
, 是
是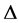 内迭代
内迭代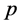 级有限的亚纯函数,并且
级有限的亚纯函数,并且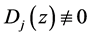 和
和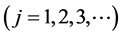
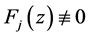 。如果
。如果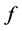 是方程(1.1)在
是方程(1.1)在 内的亚纯解满足
内的亚纯解满足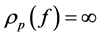 和
和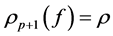 ,则
,则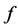 满足
满足
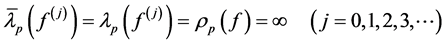
和

本文将考虑二阶非齐次线性微分方程(1.1)解的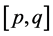 级问题,其中
级问题,其中 ,
, ,
,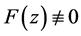 是单位圆
是单位圆 内的解析函数,我们得到以下结果。
内的解析函数,我们得到以下结果。
定理1.1:假设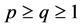 是整数,
是整数,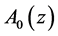 ,
, ,
,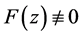 是
是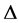 内解析函数。
内解析函数。 ,
, ,
, 也是
也是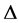 内解析函数其中
内解析函数其中 ,
, ,
, 至少有一个不等于零,而且有
至少有一个不等于零,而且有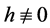 ,
, 的定义见(1.4)。如果
的定义见(1.4)。如果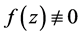 是方程(1.1)的解并且满足
是方程(1.1)的解并且满足
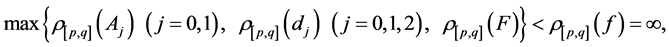 (1.8)
(1.8)
和
 .
.
则微分多项式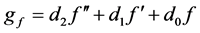 满足
满足
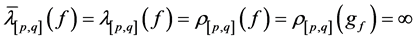
和

定理1.2:假设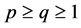 是整数,
是整数, ,
,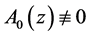 ,
, 是
是 内
内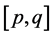 级有限的亚纯函数并且
级有限的亚纯函数并且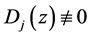 和
和
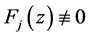 。
。 ,
,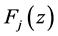 的定义见(1.6),(1.7)。如果
的定义见(1.6),(1.7)。如果 是方程(1.1)在
是方程(1.1)在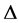 内的亚纯解并且满足
内的亚纯解并且满足 ,
, 。则有
。则有
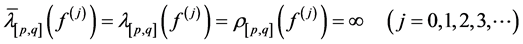
和
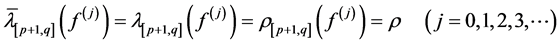
2. 主要引理
引理2.1[15] :设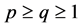 是整数,
是整数,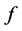 和
和 是
是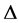 内具有
内具有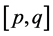 级的亚纯函数。则有
级的亚纯函数。则有
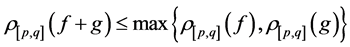
和

如果 ,则有
,则有
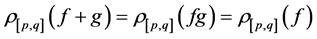
引理2.2[15] :设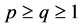 是整数,
是整数,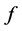 是
是 内具有
内具有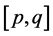 级的亚纯函数。则有
级的亚纯函数。则有
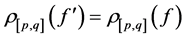 .
.
引理2.3[16] :设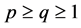 是整数,
是整数,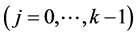
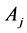 ,
, 是
是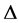 内解析函数,
内解析函数, 是微分方程(2.1)的解
是微分方程(2.1)的解
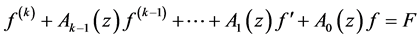 (2.1)
(2.1)
满足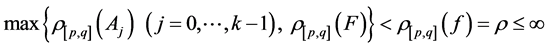 。则有
。则有
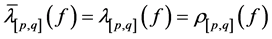
和

引理2.4[20] :设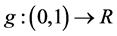 ,
,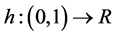 是单调递增的函数并且
是单调递增的函数并且 成立,除去一个例外集
成立,除去一个例外集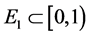 满足
满足 。则存在一个常数
。则存在一个常数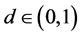 ,如果
,如果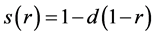 ,则有
,则有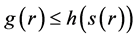 对所有的
对所有的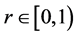 成立。
成立。
引理2.5[21] :设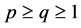 是整数,
是整数,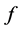 是
是 内的亚纯函数并且有
内的亚纯函数并且有 。设
。设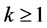 是整数。则对任意的
是整数。则对任意的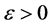 有
有
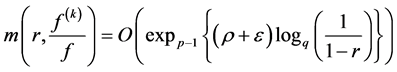 成立,除去一个例外集
成立,除去一个例外集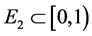 满足
满足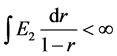 。
。
引理2.6:设 是整数,
是整数,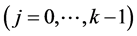
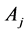 ,
,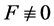 是
是 内
内 级有限的亚纯函数,
级有限的亚纯函数,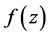 是微分方程(2.1)亚纯解,满足
是微分方程(2.1)亚纯解,满足 ,
, ,则有
,则有
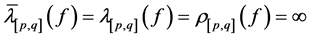
和
 .
.
证明:根据方程(2.1)得
 (2.2)
(2.2)
容易看出如果 是
是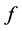 的
的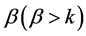 阶零点,假设
阶零点,假设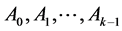 在
在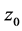 解析,则
解析,则 是
是 的
的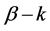 阶零点。因此有
阶零点。因此有
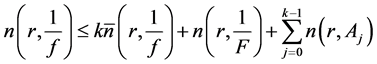 , (2.3)
, (2.3)
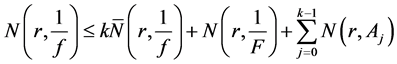 . (2.4)
. (2.4)
根据引理2.5和(2.2)得
 (2.5)
(2.5)
除去一个例外集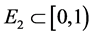 满足
满足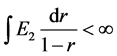 。根据(2.4),(2.5)则有
。根据(2.4),(2.5)则有
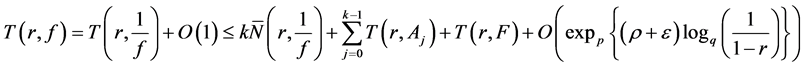 (2.6)
(2.6)
对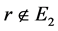 成立。
成立。
设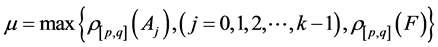 ,则当
,则当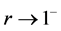 时,有
时,有
 . (2.7)
. (2.7)
根据(2.6),(2.7)我们有,当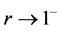 时,
时,
 (2.8)
(2.8)
其中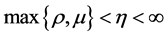 ,对
,对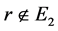 成立。
成立。
因为 ,
, ,根据引理2.4和(2.8)我们有
,根据引理2.4和(2.8)我们有
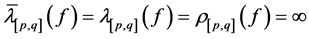
和
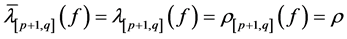
3. 定理的证明
3.1. 定理1.1的证明
假设 是方程(1.1)的解满足
是方程(1.1)的解满足
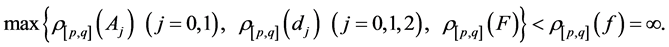
将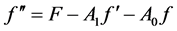 代入
代入 ,得到
,得到
 . (3.1)
. (3.1)
微分(3.1),再用 代替
代替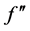 得
得
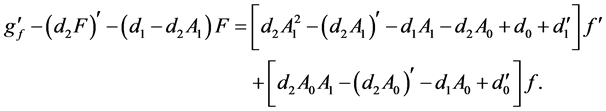 (3.2)
(3.2)
利用(1.2),(1.3),(3.1),(3.2)可改写为
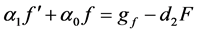 , (3.3)
, (3.3)
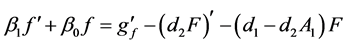 . (3.4)
. (3.4)
令
 (3.5)
(3.5)
因为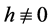 ,由(3.3)~(3.5),可得
,由(3.3)~(3.5),可得
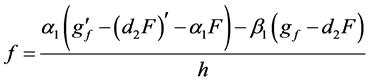 . (3.6)
. (3.6)
由(1.8),(3.1),引理2.1和引理2.2我们有 。如果
。如果
 根据(1.3),(1.8),(3.5),(3.6),引理1.1,引理1.2,得到
根据(1.3),(1.8),(3.5),(3.6),引理1.1,引理1.2,得到
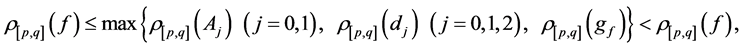
这是一个矛盾。因此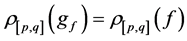 。
。
现在证明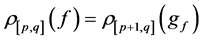 。据(3.1),引理2.1,引理2.2,有
。据(3.1),引理2.1,引理2.2,有
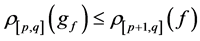 又因为
又因为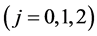
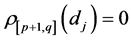 ,
,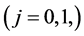
 ,
,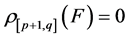 ,根据(1.3),(3.5),(3.6),引理1.1,引理1.2,得到
,根据(1.3),(3.5),(3.6),引理1.1,引理1.2,得到
 所以
所以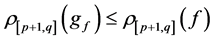 。
。
再由引理2.3有
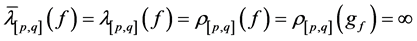
和

综上所述定理1.1证明完毕。
3.2. 定理1.2的证明
因为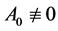 ,
, 是
是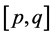 级有限的亚纯函数,应用引理2.6可得
级有限的亚纯函数,应用引理2.6可得
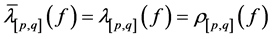
和

方程(1.1)两边除以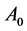 得,
得,
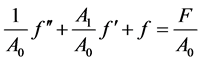 (3.7)
(3.7)
方程(3.7)两边微分得
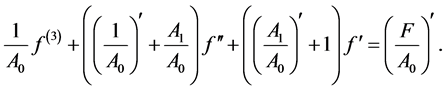 (3.8)
(3.8)
方程(3.8)两边乘以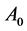 得
得
 , (3.9)
, (3.9)
其中
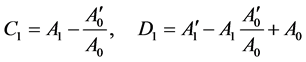
和
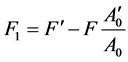 .
.
因为 ,
, ,和
,和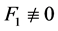 是单位圆内
是单位圆内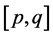 级有限的亚纯函数,对(3.9)式利用引理2.6得
级有限的亚纯函数,对(3.9)式利用引理2.6得

和
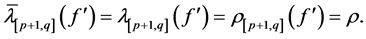
现将方程(3.9)两边除以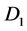 得
得
 . (3.10)
. (3.10)
方程(3.10)两边微分再乘以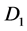 得
得
 , (3.11)
, (3.11)
由于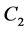 ,
,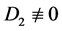 ,
,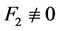 是单位圆内
是单位圆内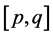 级有限的亚纯函数,定义见(1.5)~(1.7)。方程(3.11)结合引理2.6得
级有限的亚纯函数,定义见(1.5)~(1.7)。方程(3.11)结合引理2.6得
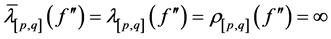
和
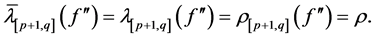
假设
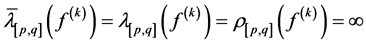 (3.12)
(3.12)
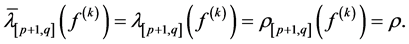 (3.13)
(3.13)
对于所有的 成立,现在证明(3.12)~(3.13)对
成立,现在证明(3.12)~(3.13)对 也成立。同前面的证明我们有
也成立。同前面的证明我们有
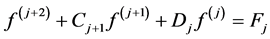 其中
其中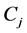 ,
,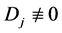 ,
, 是单位圆内
是单位圆内 级有限的亚纯函数,定义见(1.5)~(1.7)。利用引理2.6得
级有限的亚纯函数,定义见(1.5)~(1.7)。利用引理2.6得
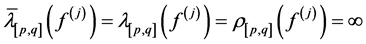
和

综上所述定理1.2证明完毕。
致 谢
感谢审稿老师对文章提出宝贵的意见。
基金项目
国家自然科学基金(11301232,11171119),江西省自然科学基金(20132BAB211009),江西省教育厅青年科学基金(GJJ12207)资助项目。
参考文献 (References)
- [1] Hayman, W.K. (1964) Meromorphic functions. Clarendon Press, Oxford.
- [2] Heittokangas, J. (2000) On complex differential equations in the unit disc. Annales Academiæ Scientiarum Fennicæ Mathematica Dissertationes, 122, 1- 54.
- [3] Laine, I. (1993) Nevanlinna Theory and complex differential equations, de Gruyter studies in mathematics, 15. Walter de Gruyter & Co., Berlin/New York.
- [4] Laine, I. (2008) Complex differential equations, handbook of differential equations: Ordinary differential equations. Handb. Differ. Equ., Elsevier/North-Holland, Amsterdam, Vol. IV, 269-363.
- [5] Tsuji, M. (1975) Potential theory in modern function theory. Chelsea, New York, Reprint of the 1959 Edition.
- [6] Belaïdi, B. (2010) Oscillation of fast growing solutions of linear differential equations in the unit disc. Acta Universitatis Sapientiae, Mathematica, 2, 25-38.
- [7] Belaïdi, B. (2011) Growth of solutions of linear differential equations in the unit disc. Bulletin of Mathematical Analysis and Applications, 3, 14-26.
- [8] Cao, T.B. and Yi, H.Y. (2006) The growth of solutions of linear differential equations with coefficients of iterated order in the unit disc. Journal of Mathematical Analysis and Applications, 319, 278-294.
- [9] Cao, T.B. (2009) The growth, oscillation and fixed points of solutions of complex linear differential equations in the unit disc. Journal of Mathematical Analysis and Applications, 352, 739-748.
- [10] Chen, Z.X. and Shon, K.H. (2004) The growth of solutions of differential equations with coefficients of small growth in the disc. Journal of Mathematical Analysis and Applications, 297, 285-304.
- [11] Chyzhykov, I.E., Gundersen, G.G. and Heittokangas, J. (2003) Linear differential equations and logarithmic derivative estimates. Proceedings of the London Mathematical Society, 86, 735-754.
- [12] Cao, T.B., Zhu, C.X. and Liu, K. (2011) On the complex oscillation of meromorphic solutions of second order linear differential equations in the unit disc. Journal of Mathematical Analysis and Applications, 374, 272-281.
- [13] Juneja, O.P., Kapoor, G.P. and Bajpai, S.K. (1976) On the
 -order and lower
-order and lower
 -order of an entire function. Journal Fur Die Reine Und Angewandte
Mathematik, 282, 53-67.
-order of an entire function. Journal Fur Die Reine Und Angewandte
Mathematik, 282, 53-67. - [14] Juneja, O.P., Kapoor, G.P. and Bajpai, S.K. (1977) On the
 -order and lower
-order and lower
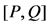 -type of an entire function. Journal Fur Die Reine Und Angewandte
Mathematik, 280, 180-190.
-type of an entire function. Journal Fur Die Reine Und Angewandte
Mathematik, 280, 180-190. - [15] Belaïdi, B. (2012) Growth and oscillation theory of
 -order analytic solutions of linear differential equations
in the unit disc. Journal of Mathematical Analysis, 3, 1-11.
-order analytic solutions of linear differential equations
in the unit disc. Journal of Mathematical Analysis, 3, 1-11. - [16] Latreuch, Z. and Belaïdi, B. (2013) Linear differential equations
with analytic coefficients of
 -order in the unit disc. Sarajevo Journal of Mathematics,
9, 71-84.
-order in the unit disc. Sarajevo Journal of Mathematics,
9, 71-84. - [17] Cao, T.B. and Deng, Z.S. (2010) Solutions of non-homogeneous linear differential equations in the unit disc. Annales Polonici Mathematici, 97, 51-61.
- [18] Latreuch, Z. and Belaïdi, B. (2013) Complex oscillation of differential polynomials in the unit disc. Periodica Mathematica Hungarica, 66, 45-60.
- [19] Latreuch, Z. and Belaïdi, B. (2013) Complex oscillation of solutions and their derivatives of non-homogenous linear differetial equations in the unit disc. International Journal of Analysis and Applications, 2, 111-123.
- [20] Bank, S. (1972) General theorem concerning the growth of solutions of first-order algebraic differential equations. Compositio Mathematica, 25, 61-70.
- [21] Belaïdi, B. (2011) Growth of solutions to linear differential
equations with analytic coefficients of
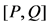 -order in the unit disc. Electron. Journal of Differential
Equations, 2011, 1-11.
-order in the unit disc. Electron. Journal of Differential
Equations, 2011, 1-11.