Advances in Applied Mathematics
Vol.04 No.03(2015), Article ID:15796,7
pages
10.12677/AAM.2015.43027
Four Types of Functions Solutions of the Novel Auxiliary Equation and Its Application on the Perturbed Nonlinear Schrödinger Equation
Xue Liu1,2, Huaitang Chen1,2
1School of Science, Linyi University, Linyi Shandong
2School of Mathematics Science, Shandong Normal University, Jinan Shandong
Email: chenhuaitang@163.com
Received: Jul. 10th, 2015; accepted: Jul. 27th, 2015; published: Aug. 3rd, 2015
Copyright © 2015 by authors and Hans Publishers Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



ABSTRACT
Four types of functions solutions of this novel auxiliary equation are gained. We obtain interaction solutions of nonlinear Schrödinger equation with perturbed terms successfully.
Keywords:Four Types of Functions Solution, Novel Auxiliary Equation Method, Interaction Solution
新辅助方程的四类函数解对带扰动项非线Schrödinger方程的应用
刘 学1,2,陈怀堂1,2
1临沂大学,山东 临沂
2山东师范大学,山东 济南
Email: chenhuaitang@163.com
收稿日期:2015年7月10日;录用日期:2015年7月27日;发布日期:2015年8月3日

摘 要
本文通过构造法求解新辅助方程的四类函数解,并将新辅助方程方法带扰动项非线性Schrödinger方程中,成功获得方程的相互作用解。
关键词 :四类函数解,新辅助方程,相互作用解

1. 引言
自发现孤立波以来,求解非线性偏微分方程的方法层出不穷。传统的方法有:Bäcklund变换[1] 、Hirota双线性变换法[2] 、Darboux变换法[3] 、Painlevé展开法[4] 等。随着计算机技术的发展,结合非线性科学和机械化数学的深入研究,人们又发现了很多求解非线性发展方程的新方法:Jacobi椭圆方程展开法[5] 、齐次平衡法[6] 、Riccati方程展开法[7] 、辅助方程法[8] 、Wronsk形式展开法[9] 、 -展开法[10] 等。前面提到的求解非线性偏微分方程的方法中:Jacobi椭圆函数展开法、齐次平衡法、Riccati函数法、
-展开法[10] 等。前面提到的求解非线性偏微分方程的方法中:Jacobi椭圆函数展开法、齐次平衡法、Riccati函数法、 -展开法都可以归类为辅助方程法[11] [12] 。辅助方程法是一种非常直接也有效的求解方程的方法。求解非线性偏微分方程,目前没有统一的求解方法,而且获得的精确解通常是单孤子解、双周期解、多孤子解,很少获得同时包含有理函数、双曲函数、三角函数、指数函数、雅克比椭圆函数的相互作用解。
-展开法都可以归类为辅助方程法[11] [12] 。辅助方程法是一种非常直接也有效的求解方程的方法。求解非线性偏微分方程,目前没有统一的求解方法,而且获得的精确解通常是单孤子解、双周期解、多孤子解,很少获得同时包含有理函数、双曲函数、三角函数、指数函数、雅克比椭圆函数的相互作用解。
本文中我们对新辅助方程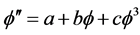 进行四类函数的求解,成功获得了三角函数、双曲函数、指数函数、Jacobi椭圆函数,并将其成功应用于带有扰动项的非线性Schrödinger方程中。
进行四类函数的求解,成功获得了三角函数、双曲函数、指数函数、Jacobi椭圆函数,并将其成功应用于带有扰动项的非线性Schrödinger方程中。
2. 新辅助方程四类函数解
新辅助方程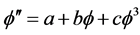 进行解的新的构造:
进行解的新的构造:
情况1: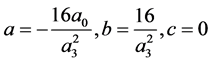
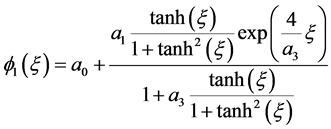
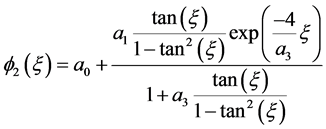 (1)
(1)
情况2: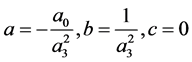

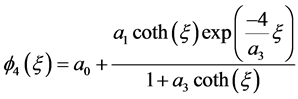
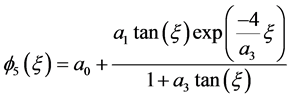 (2)
(2)
情况3: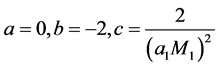
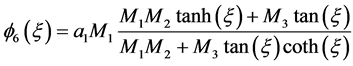
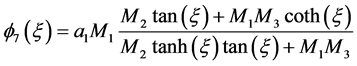
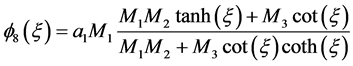

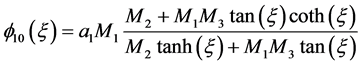
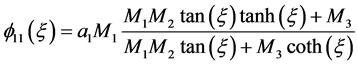
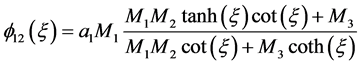
 (3)
(3)
情况4: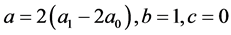
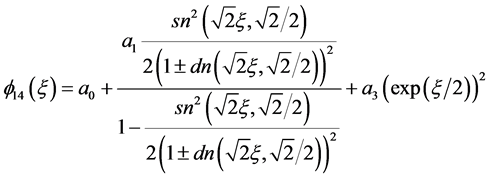
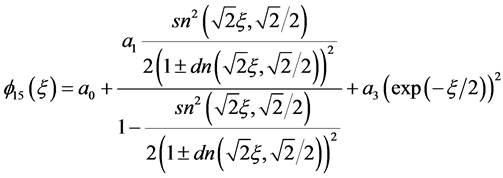 (4)
(4)
其中 为任意常数。
为任意常数。
新辅助方程方法步骤如下:
第一步:非线性偏微分方程的一般形式:
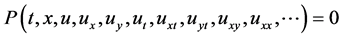 (5)
(5)
第二步:作行波变换:
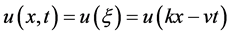 (6)
(6)
其中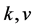 是任意常数。
是任意常数。
第三步:把(6)代入方程(5),得到一个常微分方程:
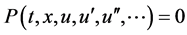 (7)
(7)
第四步:假设方程(5)的精确解有如下形式:
 (8)
(8)
其中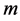 是正整数,可由齐次平衡方程(5)中的最高次项和非线性项确定其值。
是正整数,可由齐次平衡方程(5)中的最高次项和非线性项确定其值。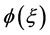 满足新辅助方程。将方程(8)代入方程(7),得到关于
满足新辅助方程。将方程(8)代入方程(7),得到关于 的多项式,合并同类型进行化简使
的多项式,合并同类型进行化简使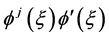 的每项系数为零,得到一系列关于
的每项系数为零,得到一系列关于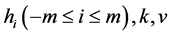 的超定方程组,借助科学工具Maple求解。
的超定方程组,借助科学工具Maple求解。
3. 扰动项的非线性 Schrödinger方程的四类函数解
带有扰动项的非线性Schrödinger方程[13] :
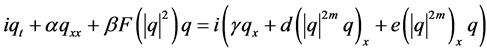 (9)
(9)
该方程作为光脉冲的传播的一个数学模型,可以广泛应用于非线性光学领域中。其中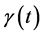 为模型内部的色散系数,
为模型内部的色散系数,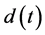 表示短脉冲的自陡峭系数,
表示短脉冲的自陡峭系数,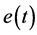 为高阶的色散系数。本文主要考虑带有扰动项方程非线性特征的Kerr律:
为高阶的色散系数。本文主要考虑带有扰动项方程非线性特征的Kerr律:
 (10)
(10)
根据Kerr律方程(9),不失一般性当 :
:
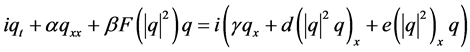 (11)
(11)
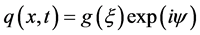 (12)
(12)
其中 ,代入方程(11)使其虚数部分为零,我们得到一个关系式:
,代入方程(11)使其虚数部分为零,我们得到一个关系式:

方程(11)简化成:
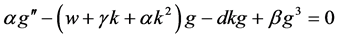 (13)
(13)
应用新辅助方程,求解方程:
1) 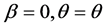
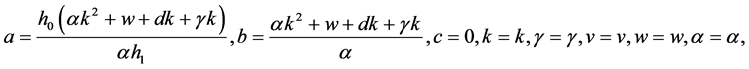
方程(11)的相互作用解:
情况1: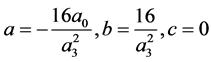
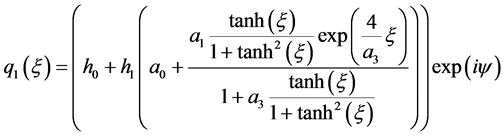
 (14)
(14)
情况2: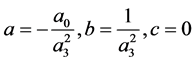
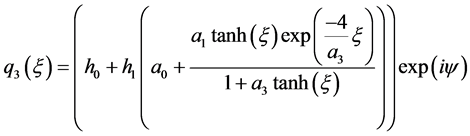
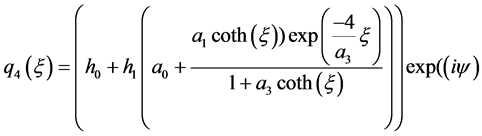
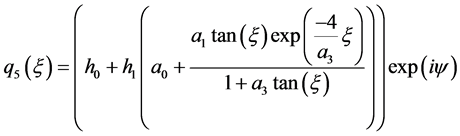 (15)
(15)
情况4: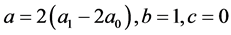
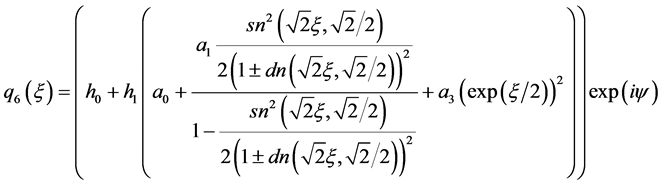
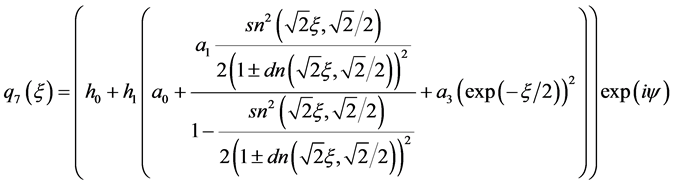 (16)
(16)
2) 当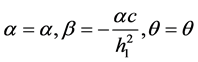
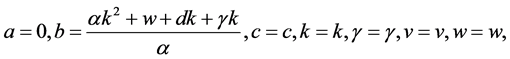
方程(11)的相互作用解:
情况3: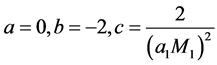
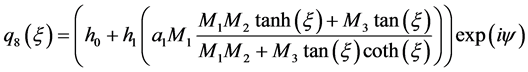
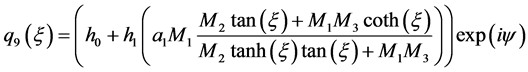


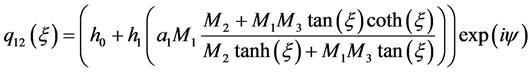

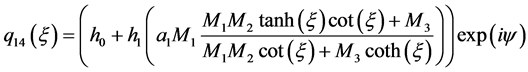
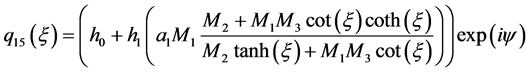 (17)
(17)
其中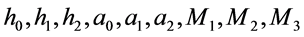 为任意常数。
为任意常数。
4. 主要结论
本文中我们对新辅助方程 进行四类函数的求解,成功获得了三角函数、双曲函数、指数函数、Jacobi椭圆函数,将其应用于带有扰动项的非线性Schrödinger方程中,获得非线性偏微分方程的相互作用解。研究非线性偏微分方程的相互作用解具有非常重要的现实依据,例如地震波、飓风波、海啸波同时作用时,我们可以通过研究其作用形式可以减弱其破坏程度。获得非线性偏微分方程相互作用解可以使我们更好的描述复杂的非线性物理现象。
进行四类函数的求解,成功获得了三角函数、双曲函数、指数函数、Jacobi椭圆函数,将其应用于带有扰动项的非线性Schrödinger方程中,获得非线性偏微分方程的相互作用解。研究非线性偏微分方程的相互作用解具有非常重要的现实依据,例如地震波、飓风波、海啸波同时作用时,我们可以通过研究其作用形式可以减弱其破坏程度。获得非线性偏微分方程相互作用解可以使我们更好的描述复杂的非线性物理现象。
基金项目
山东省自然科学基金(ZR2010AM014);临沂大学应用数学提高项目。
文章引用
刘学,陈怀堂. 新辅助方程的四类函数解对带扰动项非线Schro¨dinger方程的应用
Four Types of Functions Solutions of the Novel Auxiliary Equation and Its Application on the Perturbed Nonlinear Schro¨dinger Equation[J]. 应用数学进展, 2015, 04(03): 217-223. http://dx.doi.org/10.12677/AAM.2015.43027
参考文献 (References)
- 1. Hu, X.B. and Ma, W.X. (2002) Application of Hirota’s bilinear formalism to the Toeplitz lattice—some special soliton- like solutions. Physics Letters A, 293, 161-165. http://dx.doi.org/10.1016/S0375-9601(01)00850-7
- 2. 谷超豪 (1999) 孤立子理论中的Darboux 变换及其几何应用. 上海科技出版社, 上海.
- 3. Geng, X.G. and He, G.L. (2010) Some new integrable nonlinear evolution equations and Darboux transformation. Journal of Mathematical Physics, 51, Article ID: 033514. http://dx.doi.org/10.1063/1.3355192
- 4. Zhao, Y.L., Liu, Y.P. and Li, Z.B. (2010) A connection between the (G'/G)-expansion method and the truncated Painlevé expansion method and its application to the mKdV equation. Chinese Physics B, 19, Article ID: 030306.
- 5. Chen, H.T. and Yin, H.C. (2007) Double elliptic equation method and new exact solutions of the (n+1)-dimensional sinh-Gordon equation. Journal of Mathematical Physics, 48, Article ID: 013504. http://dx.doi.org/10.1063/1.2424550
- 6. El-Wakil, S.A., Abulwafa, E.M., Elhanbaly, A. and Abdou, M.A. (2007) The extended homogeneous balance method and its applications for a class of nonlinear evolution equations. Chaos, Solitons & Fractals, 33, 1512-1522. http://dx.doi.org/10.1016/j.chaos.2006.03.010
- 7. Chen, Y. and Li, B. (2004) General projective Riccati equation method and exact solutions for generalized KdV-type and KdV-Burgers-type equations with nonlinear terms of any order. Chaos, Solitons & Fractals, 19, 977-984. http://dx.doi.org/10.1016/S0960-0779(03)00250-9
- 8. Chen, H.T., Yang, S.H. and Ma, W.X. (2013) Double sub-equation method for complexiton solutions of nonlinear partial differential equations. Applied Mathematics and Computation, 219, 4775-4781. http://dx.doi.org/10.1016/j.amc.2012.10.094
- 9. Tang, Y.N., Ma, W.X., Xu, W. and Gao, L. (2011) Wronskian determinant solutions of the (3+1)-dimensional Jimbo- Miwa equation. Applied Mathematics and Computation, 217, 8722-8730. http://dx.doi.org/10.1016/j.amc.2011.03.120
- 10. Wang, M.L., Li, X.Z. and Zhang, J.L. (2008) The (G'/G)-expansion method and travelling wave solutions of nonlinear evolution equations in mathematical physics. Physics Letters A, 372, 417-423. http://dx.doi.org/10.1016/j.physleta.2007.07.051
- 11. 高美茹, 陈怀堂 (2012) (2+1)维sine-Gordon方程的三种函数混合的相互作用解. 物理学报, 22, 138-141.
- 12. 徐兰兰, 陈怀堂 (2013) 变系数(2+1)维Nizhnik-Novikov-Vesselov方程的三孤子新解. 物理学报, 9, 090204.
- 13. Abdel Latif, M.S. (2014) Bright and dark soliton solutions for the perturbed nonlinear Schrödinger’s equation with Kerr law and non-Kerr law nonlinearity. Applied Mathematics and Computation, 247, 501-510. http://dx.doi.org/10.1016/j.amc.2014.08.098