Pure Mathematics
Vol.
10
No.
10
(
2020
), Article ID:
38448
,
5
pages
10.12677/PM.2020.1010118
Gorenstein (m, n)-投射模
杨强
西北师范大学,数学与统计学院,甘肃 兰州

收稿日期:2020年10月8日;录用日期:2020年10月23日;发布日期:2020年10月30日

摘要
本文引入了Gorenstein (m, n)-投射模的概念。在强左(m, n)-凝聚环上研究了这类模的一些性质,并给出了Gorenstein (m, n)-投射模的一些等价刻画。
关键词
(m, n)-内射模,Gorenstein (m, n)-投射模,强(m, n)-凝聚环
Gorenstein (m, n)-Projective Modules
Qiang Yang
College of Mathematics and Statistics, Northwest Normal University, Lanzhou Gansu
Received: Oct. 8th, 2020; accepted: Oct. 23rd, 2020; published: Oct. 30th, 2020

ABSTRACT
In this article, the notion of Gorenstein (m, n)-projective modules is introduced. Some properties of such modules are investigated over strongly left (m, n)-coherent rings, and some equivalent characterizations of Gorenstein (m, n)-projective modules are given.
Keywords:(m, n)-Injective Module, Gorenstein (m, n)-Projective Module, Strongly (m, n)-Coherent Ring

Copyright © 2020 by author(s) and Hans Publishers Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY 4.0).
http://creativecommons.org/licenses/by/4.0/


1. 引言
作为有限生成投射模的一种推广,Auslander和Bridger在文献 [1] 中研究了双边Noether环上Gorenstein维数为0的有限生成模。1995年,在文献 [2] 中Enochs和Jenda在一般环上引入Gorenstein投射模和Gorenstein内射模的概念。自此,以Gorenstein投射(内射)模为研究对象的Gorenstein同调代数受到了学者们的广泛关注 [1] - [6]。
2001年,作为n-内射模,P-内射模,FP-内射模的统一推广,Chen,Ding,Li和Zhou在文献 [7] 中引入了(m, n)-内射模的概念。2005年,Zhang等人在文献 [8] 中证明了在(m, n)-凝聚环上,(m, n)-内射左R-模的示性模是(m, n)-平坦的。同年,在文献 [9] 中,Mao和Ding在凝聚环上研究了模的FP-投射维数以及环的FP-投射整体维数。2006年,Mao和Ding在文献 [10] 中证明了 是完备的余挠理论,其中 是(m, n)-投射模类, 是(m, n)-内射模类。2014年,曾月迪在文献 [11] 中引入强(m, n)-凝聚环的概念,并证明了在强(m, n)-凝聚环上, 是完备遗传余挠对。2020年,杨强和赵仁育在文献 [12] 中研究了Gorenstein (m, n)-内射模,并在强(m, n)-凝聚环上利用Gorenstein (m, n)-内射模给出了左(m, n)-内射环的一些等价刻画。
受上述研究的启发,本文引入Gorenstein (m, n)-投射模的概念。证明了Gorenstein (m, n)-投射模关于直和封闭;在强左(m, n)-凝聚环和任意环上,给出了Gorenstein (m, n)-投射模的等价刻画。
2. 预备知识
设m和n是任取的两个正整数,R是具有单位元的结合环,所涉及的模均为左R-模。用 表示模M的内射维数,用 表示所有内射维数有限的左R-模类,对于左R-模M,用 表示M的示性模。
定义2.1 称左R-模M是(m, n)-表示的,如果存在左R-模的短正合序列 ,其中K是n-生成的。
定义2.2 称左R-模M是(m, n)-内射的,如果对任意的(m, n)-表示左R-模P, 。
定义2.3 称左R-模N是(m, n)-投射的,如果对任意的(m, n)-内射左R-模M, 。
注记2.4 (1) (m, n)-表示模是(m, n)-投射模;
(2) (m, n)-投射模关于直和,直和项和扩张封闭。
定义2.5 称环R是左(m, n)-凝聚环,如果左R-模 的每一个n-生成子模是有限表示的。
定义2.6 称环R是强左(m, n)-凝聚环,如果 的每一个n-生成子模是(m, n)-表示的。
3. Gorenstein (m, n)-投射模及其性质
定义3.1 称左R-模M是Gorenstein (m, n)-投射模,如果存在(m, n)-投射左R-模的正合序列
,
使得 ,并对任意的 , 正合。
Gorenstein (m, n)-投射左R-模的类记为 。
注记3.2 (1) (m, n)-投射左R-模是Gorenstein (m, n)-投射左R-模;
(2) 若M是Gorenstein (m, n)-投射左R-模,则对任意 , ;
(3) 若 是(m, n)-投射左R-模的正合序列,并对任意的 , 正合,则每个箭头的像,核,余核都是Gorenstein (m, n)-投射左R-模;
(4) Gorenstein (m, n)-投射左R-模关于直积封闭。
命题3.3 Gorenstein (m, n)-投射左R-模关于直和封闭。
证明 设 是一簇Gorenstein (m, n)-投射左R-模,令 。对任意的 ,因为 是Gorenstein (m, n)-投射左R-模,所以存在(m, n)-投射左R-模的正合序列
,
使得 ,并对任意的 , 正合。于是有正合序列
,
使得 ,并对任意的 , 正合。因此M是Gorenstein (m, n)-投射左R-模。
命题3.4 设R是强左(m, n)-凝聚环,M是左R-模,则以下成立:
(1) 若M是Gorenstein (m, n)-投射左R-模,则对任意的 ,任意的 , ;
(2) 对任意左R-模的正合序列 , 是Gorenstein (m, n)-投射模,则对任意的 ,以及任意的 ,。
证明 (1) 设M是Gorenstein (m, n)-投射左R-模,E是(m, n)-内射左R-模,且 ,考虑下列正合序列
,
其中 是(m, n)-投射左R-模,因为R是强左(m, n)-凝聚环,由 [11] 定理2.2知 是完备遗传余挠对,所以 。故对任意的 ,。又因为 ,所以对任意的 ,。
(2) 的证明由(1)可得。
定理3.5 设R是强左(m, n)-凝聚环,M是左R-模,则M是Gorenstein (m, n)-投射左R-模当且仅当存在(m, n)-投射左R-模的正合序列
,
使得 。
证明 Þ)显然。
• )设N是(m, n)-内射左R-模,且 。下证 正合,对k进行归纳总结。当 时,结论显然成立。设 ,考虑短正合序列
,
其中E是内射模, 。则有短正合序列
,
因为R是强左(m, n)-凝聚环,由 [8] 定理5.7知, 和 是(m, n)-平坦模,故 是(m, n)-平坦模,再由 [8] 定理5.7知,L是(m, n)-内射模。由归纳假设知 正合。于是存在复形的短正合序列
,
其中 和 正合,从而由 [13] 定理6.3知, 正合。因此M是Gorenstein (m, n)-投射的。
推论3.6 设R是强左(m, n)-凝聚环,M是左R-模,则以下等价:
(1) M是Gorenstein (m, n)-投射模;
(2) 存在左R-模的正合序列 ,其中每个 是(m, n)-投射模;
(3) 存在左R-模的短正合序列 ,其中P是(m, n)-投射模,L是Gorenstein (m, n)-投射模。
证明 (1) Þ (3) Þ (2)显然。
(2) Þ (1)设 是(m, n)-投射分解。由(2)知,存在左R-模的正合序列
,
其中 是(m, n)-投射左R-模,于是有左R-模的正合序列
,
使得 。故由定理3.5知M是Gorenstein (m, n)-投射模。
命题3.7 设R是强左(m, n)-凝聚环, 是左R-模的短正合序列。则以下成立:
(1) 若 是Gorenstein (m, n)-投射模, 是(m, n)-投射模,则 是Gorenstein (m, n)-投射模;
(2) 若 是Gorenstein (m, n)-投射模, 是(m, n)-投射模,则 是Gorenstein (m, n)-投射模。
证明 (1) 因为 是Gorenstein (m, n)-投射模,所以存在左R-模的短正合序列 ,其中P是(m, n)-投射模,则N是Gorenstein (m, n)-投射模。考虑下列推出图1:

Figure 1. Pushout diagram
图1. 推出图
在短正合序列
中,P和
是(m, n)-投射模,由 [9] 注记2.8知,Q是(m, n)-投射模。在短正合序列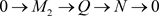 中,因为N是Gorenstein (m, n)-投射模,所以由推论3.6知,
中,因为N是Gorenstein (m, n)-投射模,所以由推论3.6知,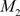 是Gorenstein (m, n)-投射模。
是Gorenstein (m, n)-投射模。
(2) 类似地,由推论3.6可得。
定理 3.8 设R环,则以下等价:
(1) 每个左R-模是Gorenstein (m, n)-投射的;
(2) 环R满足以下两个条件:
(i) 每个内射左R-模是(m, n)-投射的;
(ii) 每个内射维数有限的(m, n)-内射左R-模是内射的。
证明 (1) Þ (2) 设M是内射左R-模。则有(1)知M是Gorenstein (m, n)-投射模。于是存在短正合序列
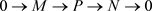 ,
,
其中P是(m, n)-投射模,由于M是内射模,所以该正合序列可裂。因此M是P的直和项,由注记2.4 (2)知,M是(m, n)-投射模。故(i)成立。设E是内射维数有限的(m, n)-内射左R-模,N是一个左R-模。由(1)知,N是Gorenstein (m, n)-投射模。于是由注记3.2 (2)知, ,所以E是内射模,故(ii)成立。
,所以E是内射模,故(ii)成立。
(2) Þ (1) 设M是左R-模。 是M的一个(m, n)-投射分解,
是M的一个(m, n)-投射分解,
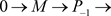 是M的一个内射分解,由(i)知存在(m, n)-投射左R-模的正合序列
是M的一个内射分解,由(i)知存在(m, n)-投射左R-模的正合序列
 ,
,
使得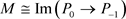 。设E内射维数有限的(m, n)-内射左R-模,由(ii)知,E是内射模,所以
。设E内射维数有限的(m, n)-内射左R-模,由(ii)知,E是内射模,所以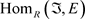 正合。因此M是Gorenstein (m, n)-投射模。
正合。因此M是Gorenstein (m, n)-投射模。
基金项目
国家自然科学基金资助项目(11861055)。
文章引用
杨 强. Gorenstein (m, n)-投射模
Gorenstein (m, n)-Projective Modules[J]. 理论数学, 2020, 10(10): 1002-1006. https://doi.org/10.12677/PM.2020.1010118
参考文献
- 1. Auslander, M. and Bridger, M. (1969) Stable Module Theory. In: Memoirs of the American Mathematical Society, 94. https://doi.org/10.1090/memo/0094
- 2. Enoch, E.E. and Jenda, O.M.G. (1995) Gorenstein Injective and Projective Modules. Mathematische Zeitschrift 220, 611-633. https://doi.org/10.1007/BF02572634
- 3. Christensen, L.W. (2000) Gorenstein Dimension. In: Lecture Notes in Math, Springer-Verlag, Berlin. https://doi.org/10.1007/BFb0103984
- 4. Enoch, E.E. and Jenda, O.M.G. (2000) Relative Homological Algerbra. Walter de Gruyter, Berlin. https://doi.org/10.1515/9783110803662
- 5. Holm, H. (2004) Gorenstein Homological Dimensions. Journal of Pure and Applied Algebra, 189, 167-193. https://doi.org/10.1016/j.jpaa.2003.11.007
- 6. Holm, H. (2004) Gorenstein Derived Functors. Proceedings of the American Mathematical Society, 132, 1913-1923. https://doi.org/10.1090/S0002-9939-04-07317-4
- 7. Chen, J.L., Ding, N.Q., Li, Y.L. and Zhou, Y.Q. (2001) On (m, n)-Injectivity of Modules. Communications in Algebra, 29, 5589-5603. https://doi.org/10.1081/AGB-100107948
- 8. Zhang, X.X., Chen, J.L. and Zhang, J. (2005) On (m, n)-Injective Modules and (m, n)-Coherent Rings. Algebra Colloquium, 12, 149-160. https://doi.org/10.1142/S1005386705000143
- 9. Mao, L.X. and Ding, N.Q. (2005) Fp-Projective Dimensions. Communications in Algebra, 33, 1153-1170. https://doi.org/10.1081/AGB-200053832
- 10. Mao, L.X. and Ding, N.Q. (2006) On Relative Injective Modules and Relative Coherent Rings. Communications in Algebra, 34, 2531-2545. https://doi.org/10.1080/00927870600651208
- 11. 曾月迪. 强(m, n)-凝聚环[J]. 江南大学学报(自然科学版), 2014, 13(5): 611-615.
- 12. 杨强, 赵仁育. Gorenstein (m, n)-内射模[J]. 山东大学学报(理学版), 2020, 55(12): 18-22.
- 13. Rotman, J.J. (1979) An Introduction to Homological Algebra. Academic Press, New York.