高离化态Mn离子的Kα谱线性质的理论研究 Theoretical Research on Kα X-Rays of Highly Ionized Manganese Ion

doi:10.12677/APP.2013.37026

设为首页加入收藏期刊导航网站地图

期刊菜单

文章导航

Applied Physics 应用物理, 2013, 3, 134-140

http://dx.doi.org/10.12677/app.2013.37026 Published Online September 2013 (http://www.hanspub.org/journal/app.html)

Theoretical Research on K



X-Rays of Highly

Ionized Manganese Ion*

Huili Li, Guohua Jin, li Zhou

School of Computer, Jiangxi University of Traditional Chinese Medicine, Nanchang

Email: huili_scu@126.com

Received: Aug. 2nd, 2013; revised: Aug. 16th, 2013; accepted: Aug. 21st, 2013

stricted use, distribution, and reproduction in any medium, provided the original work is properly cited.

Abstract: By using relativistic configuration interaction (RCI) and multi-configuration Dirac-Fock (MCDF) method,

we have calculated the transition energies, transition probabilities, absorption oscillator strengths and line strengths Kα

X-ray from MnXVII through XXIV. Furthermore, configuration interaction includes Berit interaction and quantum elec-

trodynamics (QED) corrections. Our calculated results are compared with the other available data on He-like and

Li-like manganese and are in accord with them. The present wide range data are useful for studying manganese plasma.

Keywords: Highly Ionized; Mn Ion; Spectrum Properties

高离化态 Mn 离子的 K



谱线性质的理论研究*

李会丽，金国华，周丽

江西中医药大学，计算机学院，南昌

Email: huili_scu@126.com

收稿日期：2013 年8月2日；修回日期：2013年8月16 日；录用日期：2013年8月21日

摘要：基于相对论组态相互作用和多组态Dirac-Fock (MCDF)方法，我们系统地研究和讨论了K



谱线类氦到

类铍的跃迁能级，跃迁几率，吸收振子强度和线强度的性质。组态相互作用计算包括Breit 相互作用，量子电动

力学修正。我们的理论数据与可得到的实验数据(类氦和类锂)符合得很好，这些数据为等离子体锰离子的研究提

供了有价值的信息。

关键词：高离化；锰离子；谱线性质

1. 引言

原子的核外电子大量剥离形成高离化态原子，其

光谱特征与电离后剩下的核外电子的行为有关，是近

代原子物理学研究的热点之一，我们知道 K



射线的

能级和跃迁几率在天体物理的分光镜应用，溶解等离

子体和 x射线激光方面起着很重要的作用[1-5]。K壳发

射的光谱可以比较可靠的用来诊断发生在宇宙空间

的电离区，并且识别比较年轻的超星系残迹。锰元素

不仅是比较丰富的元素而且是恒星和气态星云演变

扮演重要的角色，所以锰元素是研究 Kx-射线重要的

元素。到目前为止，尚无对高离化锰的 K



射线光谱

的系统完整的研究，特别是从类 He锰到类 F锰。所

以详细研究这些射线的性质是很有必要的。

2. 理论方法

*基金项目：国家自然基金项目(11147156, 11264019)；江西省教育

厅科学技术研究项目(12532)。多组态 Dirac-Fock 方法扩展的优化版本(EOL)是

134

高离化态 Mn 离子的 K谱线性质的理论研究

用来计算跃迁的，该方法的理论基础很多研究者[6-11]

已经讨论过，在这我们简单介绍一下：

在Dirac-Fock方法中，一定J和宇称的组态函数





是Dirac 轨道的行列式的线性组合而成的。这

些组态函数(CSF)的线性组合是用来构建相同的 J和

宇称的原子态函数(AS)

 

csf









 

 (1)

式子中是态的混合系数，是包括在原子态函

数(ASF)的估计的组态函数(CSF)的数目。构建的原子

态函数(ASF) 是用来求解 Dirac-Fock 方程，Dira-

Coulomb 自旋哈密顿量可以表示成：

Cicsf



111

ˆˆ ˆˆ

NNN

iiji

Hi rr







 (2)

式子中第一项是jj耦合，是一体的贡献来自电子动能

和与核的相互作用的。第二项是两体Coulomb相互作

用是电子之间产生的这些贡献主要来自 Breit 相互作

用，真空极化，自洽场能量，并且作为一级微扰附加

项。对于 Breit 修正和高阶修正项是与已有的文献中

的方法一样[10,12]。

相对论的跃迁几率可以通过 Coulomb 规范和

Babushkin 规范来计算，一般认为非相对论速度方向

上的测量和长度方向上的测量。用这两种类型规范计

算的跃迁比率与多组态模型略有不同，但是这两个规

范都与全组态相互作用波函数一致。

基于多组态的 Dirac-Fock 方法的组态相互作用的

扩展，本文系统计算了高离化态Mn 的K



和K



线的

一些性质。有限制的激发空间方法用来构建组态函数

(CSF)。这种限制激发空间的方法的思想是只考虑激

发空间的电子从占有的轨道激发到空轨道。为了检测

计算的收敛轨道也相应地增加，激发组通常随着轨道

层数增加而扩大，由于具有相同主量子数n通常有相

似的能量。例如轨道层时的一组

3n



1,2,2 ,3,3 ,3

spspd

1,2 ,2,,4 ,4,4{

和轨道层的 4n

优化轨道是用于组态相互作用的计算。为了产生

基本轨道的初步预算，Thomas-Fermi势能也将用在计

算中，层层扩大的优化用于下面的 CI计算。具体的

步骤为：首先根据组态波函数来优化的轨道，固

定

3n



的轨道来优化 4n



层的轨道。用同样的方法

来优化 567n



，，的情况。优化以属于 LS项的所有精

细结构的(2J + 1)为权重并且促使自洽标准以10−8收

敛。在次方法中，用哈密顿矩阵的块结构，可以同时

得到不同角量子J和宇称的态。由于J和宇称都是好

量子数并且基函数是本征态，以便于基函数阶的重排

促使具有相同J和宇称的态分成特别的块。得到基函

数以后，通过对角化哈密顿矩阵，包括 Breit 相互作

用，真空极化和自洽场能量修正，基于相对论组态相

对论组态相互作用的计算来确定 CSF 扩展系数。在

RCI 的计算中，反复的 Davidson方法和矩阵表示将用

于比较大的扩展。

通过相对论组态相互作用和多组态 Dirac-Fock

(MCDF)方法，我们系统地计算了了K谱线类氦到类

氟的跃迁能级，跃迁几率，吸收振子强度和线强度的

性质。

3. 计算结果与讨论

为了检测我们理论计算的可靠性，在表 1中，我

们列出了随着轨道层不断增加的两组跃迁的MCD F

和RCI 的长度和速度规范线强度的比较计算。从表中

可以看出：这两种长度和速度规范形式相互符合得很

好，而且随着轨道层数的增加符合度也在不断改善。

长度值相对比较稳定，这是由于长度随着激发空间的

扩展变化比较小，所以在我们的计算中，我们采用的

是长度规范。

为了更进一步地检验我们理论计算的可信度，在

表2中，我们的理论计算值与相应的实验数据作了比

较，我们可以看到类 He锰和类 Li 锰的K射线的能

级和波长与相对应的实验值符合得较好，并且类He

锰能级与相应的实验值的不同度小于0.2%，类 Li锰

能级与相应的实验值的不同度小于0.6%。这些理论与

实验的比较可以证明我们理论计算的方法是可行的。

}

sp spd f。比较大的轨道组会导致

比较大的计算量，所以适当的限制也是有必要的。基

于上面的计算方法，激发空间是由主量子数 n = 1~7

的所有轨道组成并且只是l从s到f。由于双激发相比

单激发比较精确，在我们的计算中我们只考虑双激

发。

基于 MCDF方法和 RCI 计算，运用扩展的优化

版本(EOL)，我们系统计算了高离化锰类 He 到类 F



射线的跃迁能级，跃 (

的迁几率 l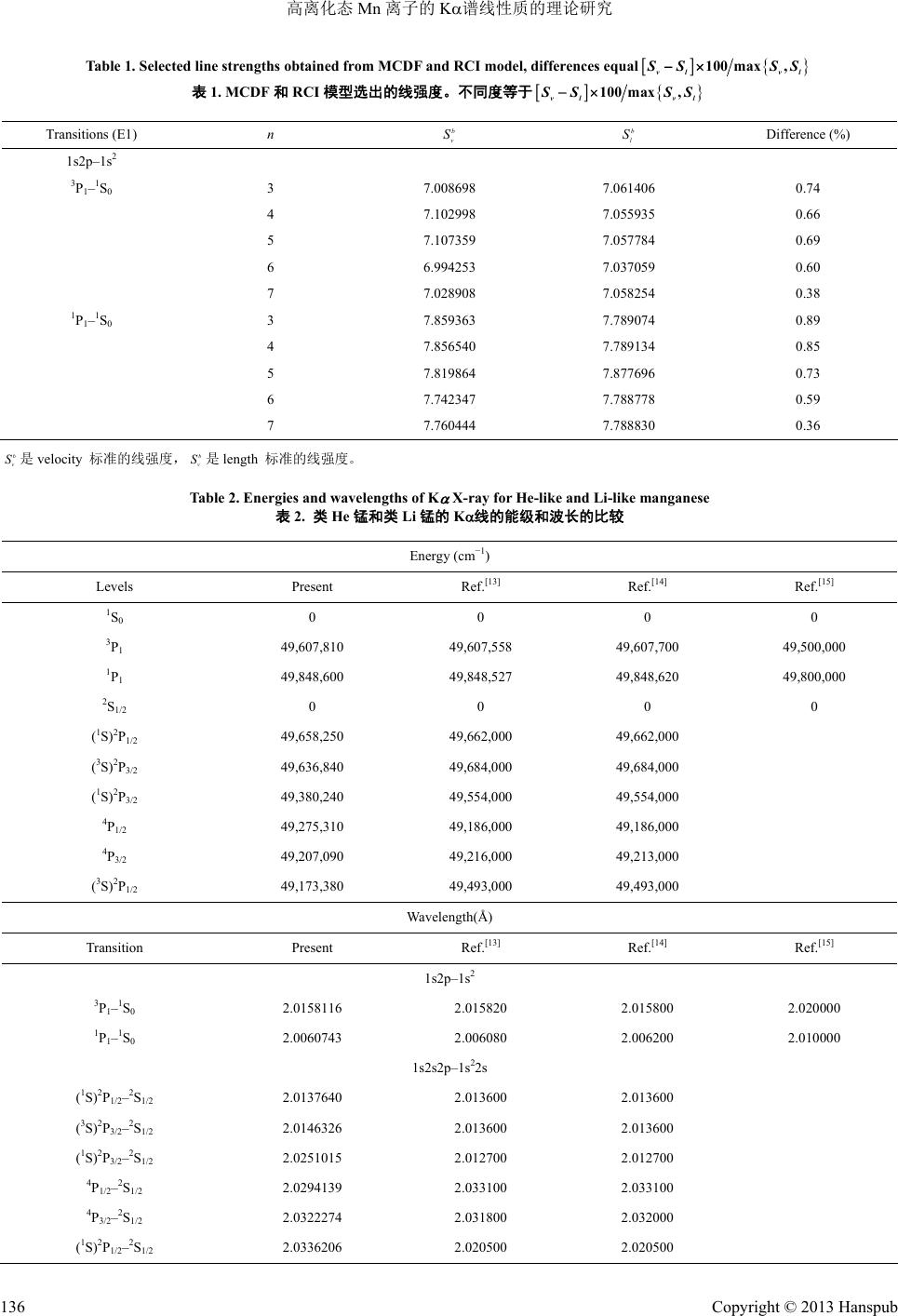

)，吸收震荡能

高离化态 Mn 离子的 K谱线性质的理论研究

136

Table 1. Selected line strengths obtained from MCDF and RCI model, differences equal









100max,

vl vl

SS S

表1. MCDF和RCI模型选出的线强度。不同度等于









100max,

vl vl

SS S

Transitions (E1) n b

S b

S Difference (%)

1s2p–1s2

3P1–1S0 3 7.008698 7.061406 0.74

4 7.102998 7.055935 0.66

5 7.107359 7.057784 0.69

6 6.994253 7.037059 0.60

7 7.028908 7.058254 0.38

1P1–1S0 3 7.859363 7.789074 0.89

4 7.856540 7.789134 0.85

5 7.819864 7.877696 0.73

6 7.742347 7.788778 0.59

7 7.760444 7.788830 0.36

S是velocity 标准的线强度，是 length 标准的线强度。

Table 2. Energies and wavelengths of K



X-ray for He-like and Li-like manganese

表2. 类He 锰和类 Li锰的K线的能级和波长的比较

Energy (cm−1)

Levels Present Ref.[13] Ref.[14] Ref.[15]

1S0 0 0 0 0

3P1 49,607,810 49,607,558 49,607,700 49,500,000

1P1 49,848,600 49,848,527 49,848,620 49,800,000

2S1/2 0 0 0 0

(1S)2P1/2 49,658,250 49,662,000 49,662,000

(3S)2P3/2 49,636,840 49,684,000 49,684,000

(1S)2P3/2 49,380,240 49,554,000 49,554,000

4P1/2 49,275,310 49,186,000 49,186,000

4P3/2 49,207,090 49,216,000 49,213,000

(3S)2P1/2 49,173,380 49,493,000 49,493,000

Wavelength(Å)

Tr a nsi t io n Present Ref.[13] Ref.[14] Ref.[15]

1s2p–1s2

3P1–1S0 2.0158116 2.015820 2.015800 2.020000

1P1–1S0 2.0060743 2.006080 2.006200 2.010000

1s2s2p–1 s22s

(1S)2P1/2–2S1/2 2.0137640 2.013600 2.013600

(3S)2P3/2–2S1/2 2.0146326 2.013600 2.013600

(1S)2P3/2–2S1/2 2.0251015 2.012700 2.012700

4P1/2–2S1/2 2.0294139 2.033100 2.033100

4P3/2–2S1/2 2.0322274 2.031800 2.032000

(1S)2P1/2–2S1/2 2.0336206 2.020500 2.020500

高离化态 Mn 离子的 K谱线性质的理论研究

Table 3. Present results of transit i on energies i n au, transition probabilities () in



, absorption oscillator strengths () in length gauge,

the line strengths (S) and the rations of length to velocity rates (

AA) for K



X-ray from He-like to F-like manga nese

表3. 高离化态类 He Mn到类F Mn的K线的跃迁能级(au)，跃迁几率 l以A1



为单位，长度与速度的比率(lv

AA)，吸收振子强度 (长

度规范)和线强度 S(au)

Transition Energy

A l

1s2p–1s2

1P1–1S0 227.1300 1.1825(15) 1.0019 7.1345(−1) 4.7118(−3)

3P1–1S0 226.0329 9.8063(13) 1.0044 5.9739(−2) 3.9645(−4)

1s2s2p–1 s22s

(1S)2P1/2–2S1/2 226.2627 1.8933(14) 1.0040 2.3021(−1) 1.5262(−3)

(3S)2P3/2–2S1/2 226.1651 2.4720(14) 1.0039 3.0084(−1) 1.9953(−3)

(1S)2P3/2–2S1/2 224.9960 5.3832(14) 1.0070 6.6195(−1) 4.4131(−3)

4P1/22–2S1/2 224.5179 2.1183(14) 1.0076 2.6159(−1) 1.7477(−3)

4P3/2–2S1/2 224.1711 3.9245(13) 1.0073 4.8614(−2) 3.2529(−4)

(3S)2P1/2–2S1/2 224.0534 1.1148(13) 1.0077 1.3823(−2) 9.2548(−5)

1s2s22p–1s22s2

1P1–1S0 224.5275 1.1079(15) 1.1123 6.8404(−1) 4.5699(−3)

3P1–1S0 223.4706 8.7084(13) 1.1104 5.4275(−2) 3.6431(−4)

1s2s22p2–1s22s22p

2S1/2–2P1/2 224.0145 4.6916(12) 1.0489 5.8196(−3) 3.8969(−5)

2D3/2–2P1/2 223.5850 1.6037(13) 1.1509 1.9970(−2) 1.3397(−4)

2S1/2–2P3/2 223.5501 1.0464(14) 1.1144 2.6069(−1) 1.7492(−3)

2D3/2–2P3/2 223.1206 4.7744(14) 1.1316 1.1940 (0) 8.0272(−3)

2p1/2–2P1/2 223.0392 4.2616(14) 1.1305 5.3326(−1) 3.5863(−3)

4p3/2–2P1/2 223.0121 4.9102(14) 1.1348 6.1457(−1) 4.1337(−3)

2D5/2–2P3/2 222.6955 2.5645(14) 1.1245 6.4379(−1) 4.3364(−3)

2P1/2–2P3/2 222.5748 5.4973(13) 1.1382 1.3815(−1) 9.3106(−4)

4P3/2–2P3/2 222.5476 2.7204(13) 1.1381 6.8384(−2) 4.6092(−4)

2P3/2–2P1/2 222.0115 4.7456(10) 1.2201 5.9856(−5) 1.0416(−7)

4P1/2–2P1/2 221.8981 1.7330(13) 1.1194 2.1909(−2) 1.4810(−4)

4P5/2–2P3/2 221.8780 3.0134(13) 1.1220 7.8482(−2) 5.3058(−4)

2P3/2–2P3/2 221.6912 6.7355(12) 1.1284 1.7062(−2) 1.1544(−4)

4P1/2–2P3/2 221.4337 3.2325(11) 1.0525 8.2077(−4) 5.5600(−6)

1s2s22p3–1s22s22p2

1P1–3P0 223.0440 1.5111(09) 0.9128 9.4540(−7) 6.3580(−9)

1P1–3P1 222.7801 1.7235(12) 1.1577 3.2425(−3) 2.1832(−5)

1P1–3P2 222.5996 2.7913(11) 1.2979 8.7666(−4) 5.9075(−6)

3P2–3P1 222.3494 6.7020(12) 1.1589 1.2657(−2) 8.5392(−5)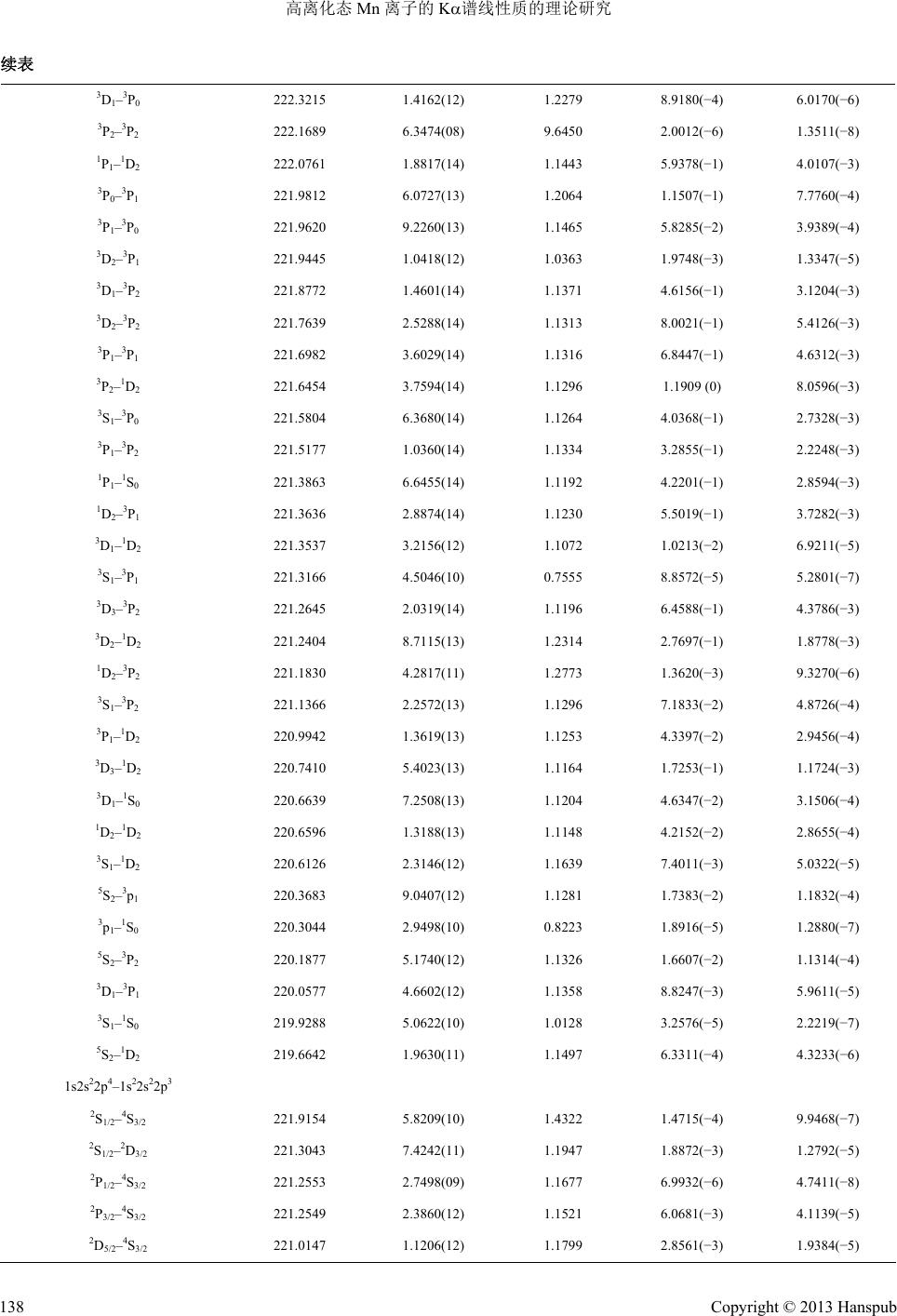

高离化态 Mn 离子的 K谱线性质的理论研究

续表

3D1–3P0 222.3215 1.4162(12) 1.2279 8.9180(−4) 6.0170(−6)

3P2–3P2 222.1689 6.3474(08) 9.6450 2.0012(−6) 1.3511(−8)

1P1–1D2 222.0761 1.8817(14) 1.1443 5.9378(−1) 4.0107(−3)

3P0–3P1 221.9812 6.0727(13) 1.2064 1.1507(−1) 7.7760(−4)

3P1–3P0 221.9620 9.2260(13) 1.1465 5.8285(−2) 3.9389(−4)

3D2–3P1 221.9445 1.0418(12) 1.0363 1.9748(−3) 1.3347(−5)

3D1–3P2 221.8772 1.4601(14) 1.1371 4.6156(−1) 3.1204(−3)

3D2–3P2 221.7639 2.5288(14) 1.1313 8.0021(−1) 5.4126(−3)

3P1–3P1 221.6982 3.6029(14) 1.1316 6.8447(−1) 4.6312(−3)

3P2–1D2 221.6454 3.7594(14) 1.1296 1.1909 (0) 8.0596(−3)

3S1–3P0 221.5804 6.3680(14) 1.1264 4.0368(−1) 2.7328(−3)

3P1–3P2 221.5177 1.0360(14) 1.1334 3.2855(−1) 2.2248(−3)

1P1–1S0 221.3863 6.6455(14) 1.1192 4.2201(−1) 2.8594(−3)

1D2–3P1 221.3636 2.8874(14) 1.1230 5.5019(−1) 3.7282(−3)

3D1–1D2 221.3537 3.2156(12) 1.1072 1.0213(−2) 6.9211(−5)

3S1–3P1 221.3166 4.5046(10) 0.7555 8.8572(−5) 5.2801(−7)

3D3–3P2 221.2645 2.0319(14) 1.1196 6.4588(−1) 4.3786(−3)

3D2–1D2 221.2404 8.7115(13) 1.2314 2.7697(−1) 1.8778(−3)

1D2–3P2 221.1830 4.2817(11) 1.2773 1.3620(−3) 9.3270(−6)

3S1–3P2 221.1366 2.2572(13) 1.1296 7.1833(−2) 4.8726(−4)

3P1–1D2 220.9942 1.3619(13) 1.1253 4.3397(−2) 2.9456(−4)

3D3–1D2 220.7410 5.4023(13) 1.1164 1.7253(−1) 1.1724(−3)

3D1–1S0 220.6639 7.2508(13) 1.1204 4.6347(−2) 3.1506(−4)

1D2–1D2 220.6596 1.3188(13) 1.1148 4.2152(−2) 2.8655(−4)

3S1–1D2 220.6126 2.3146(12) 1.1639 7.4011(−3) 5.0322(−5)

5S2–3p1 220.3683 9.0407(12) 1.1281 1.7383(−2) 1.1832(−4)

3p1–1S0 220.3044 2.9498(10) 0.8223 1.8916(−5) 1.2880(−7)

5S2–3P2 220.1877 5.1740(12) 1.1326 1.6607(−2) 1.1314(−4)

3D1–3P1 220.0577 4.6602(12) 1.1358 8.8247(−3) 5.9611(−5)

3S1–1S0 219.9288 5.0622(10) 1.0128 3.2576(−5) 2.2219(−7)

5S2–1D2 219.6642 1.9630(11) 1.1497 6.3311(−4) 4.3233(−6)

1s2s22p4–1s22s22p3

2S1/2–4S3/2 221.9154 5.8209(10) 1.4322 1.4715(−4) 9.9468(−7)

2S1/2–2D3/2 221.3043 7.4242(11) 1.1947 1.8872(−3) 1.2792(−5)

2P1/2–4S3/2 221.2553 2.7498(09) 1.1677 6.9932(−6) 4.7411(−8)

2P3/2–4S3/2 221.2549 2.3860(12) 1.1521 6.0681(−3) 4.1139(−5)

2D5/2–4S3/2 221.0147 1.1206(12) 1.1799 2.8561(−3) 1.9384(−5)

138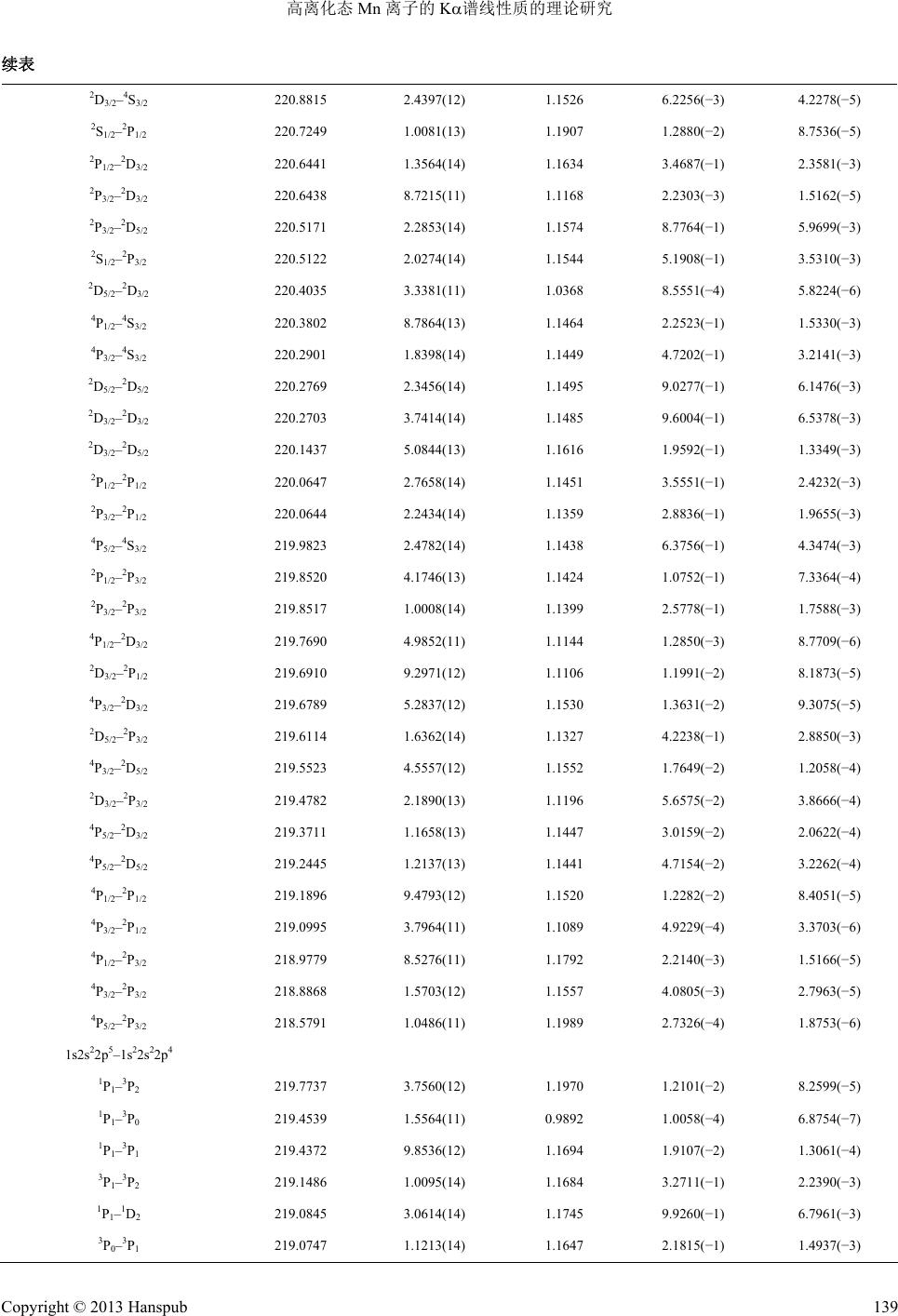

高离化态 Mn 离子的 K谱线性质的理论研究

续表

2D3/2–4S3/2 220.8815 2.4397(12) 1.1526 6.2256(−3) 4.2278(−5)

2S1/2–2P1/2 220.7249 1.0081(13) 1.1907 1.2880(−2) 8.7536(−5)

2P1/2–2D3/2 220.6441 1.3564(14) 1.1634 3.4687(−1) 2.3581(−3)

2P3/2–2D3/2 220.6438 8.7215(11) 1.1168 2.2303(−3) 1.5162(−5)

2P3/2–2D5/2 220.5171 2.2853(14) 1.1574 8.7764(−1) 5.9699(−3)

2S1/2–2P3/2 220.5122 2.0274(14) 1.1544 5.1908(−1) 3.5310(−3)

2D5/2–2D3/2 220.4035 3.3381(11) 1.0368 8.5551(−4) 5.8224(−6)

4P1/2–4S3/2 220.3802 8.7864(13) 1.1464 2.2523(−1) 1.5330(−3)

4P3/2–4S3/2 220.2901 1.8398(14) 1.1449 4.7202(−1) 3.2141(−3)

2D5/2–2D5/2 220.2769 2.3456(14) 1.1495 9.0277(−1) 6.1476(−3)

2D3/2–2D3/2 220.2703 3.7414(14) 1.1485 9.6004(−1) 6.5378(−3)

2D3/2–2D5/2 220.1437 5.0844(13) 1.1616 1.9592(−1) 1.3349(−3)

2P1/2–2P1/2 220.0647 2.7658(14) 1.1451 3.5551(−1) 2.4232(−3)

2P3/2–2P1/2 220.0644 2.2434(14) 1.1359 2.8836(−1) 1.9655(−3)

4P5/2–4S3/2 219.9823 2.4782(14) 1.1438 6.3756(−1) 4.3474(−3)

2P1/2–2P3/2 219.8520 4.1746(13) 1.1424 1.0752(−1) 7.3364(−4)

2P3/2–2P3/2 219.8517 1.0008(14) 1.1399 2.5778(−1) 1.7588(−3)

4P1/2–2D3/2 219.7690 4.9852(11) 1.1144 1.2850(−3) 8.7709(−6)

2D3/2–2P1/2 219.6910 9.2971(12) 1.1106 1.1991(−2) 8.1873(−5)

4P3/2–2D3/2 219.6789 5.2837(12) 1.1530 1.3631(−2) 9.3075(−5)

2D5/2–2P3/2 219.6114 1.6362(14) 1.1327 4.2238(−1) 2.8850(−3)

4P3/2–2D5/2 219.5523 4.5557(12) 1.1552 1.7649(−2) 1.2058(−4)

2D3/2–2P3/2 219.4782 2.1890(13) 1.1196 5.6575(−2) 3.8666(−4)

4P5/2–2D3/2 219.3711 1.1658(13) 1.1447 3.0159(−2) 2.0622(−4)

4P5/2–2D5/2 219.2445 1.2137(13) 1.1441 4.7154(−2) 3.2262(−4)

4P1/2–2P1/2 219.1896 9.4793(12) 1.1520 1.2282(−2) 8.4051(−5)

4P3/2–2P1/2 219.0995 3.7964(11) 1.1089 4.9229(−4) 3.3703(−6)

4P1/2–2P3/2 218.9779 8.5276(11) 1.1792 2.2140(−3) 1.5166(−5)

4P3/2–2P3/2 218.8868 1.5703(12) 1.1557 4.0805(−3) 2.7963(−5)

4P5/2–2P3/2 218.5791 1.0486(11) 1.1989 2.7326(−4) 1.8753(−6)

1s2s22p5–1s22s22p4

1P1–3P2 219.7737 3.7560(12) 1.1970 1.2101(−2) 8.2599(−5)

1P1–3P0 219.4539 1.5564(11) 0.9892 1.0058(−4) 6.8754(−7)

1P1–3P1 219.4372 9.8536(12) 1.1694 1.9107(−2) 1.3061(−4)

3P1–3P2 219.1486 1.0095(14) 1.1684 3.2711(−1) 2.2390(−3)

1P1–1D2 219.0845 3.0614(14) 1.1745 9.9260(−1) 6.7961(−3)

3P0–3P1 219.0747 1.1213(14) 1.1647 2.1815(−1) 1.4937(−3)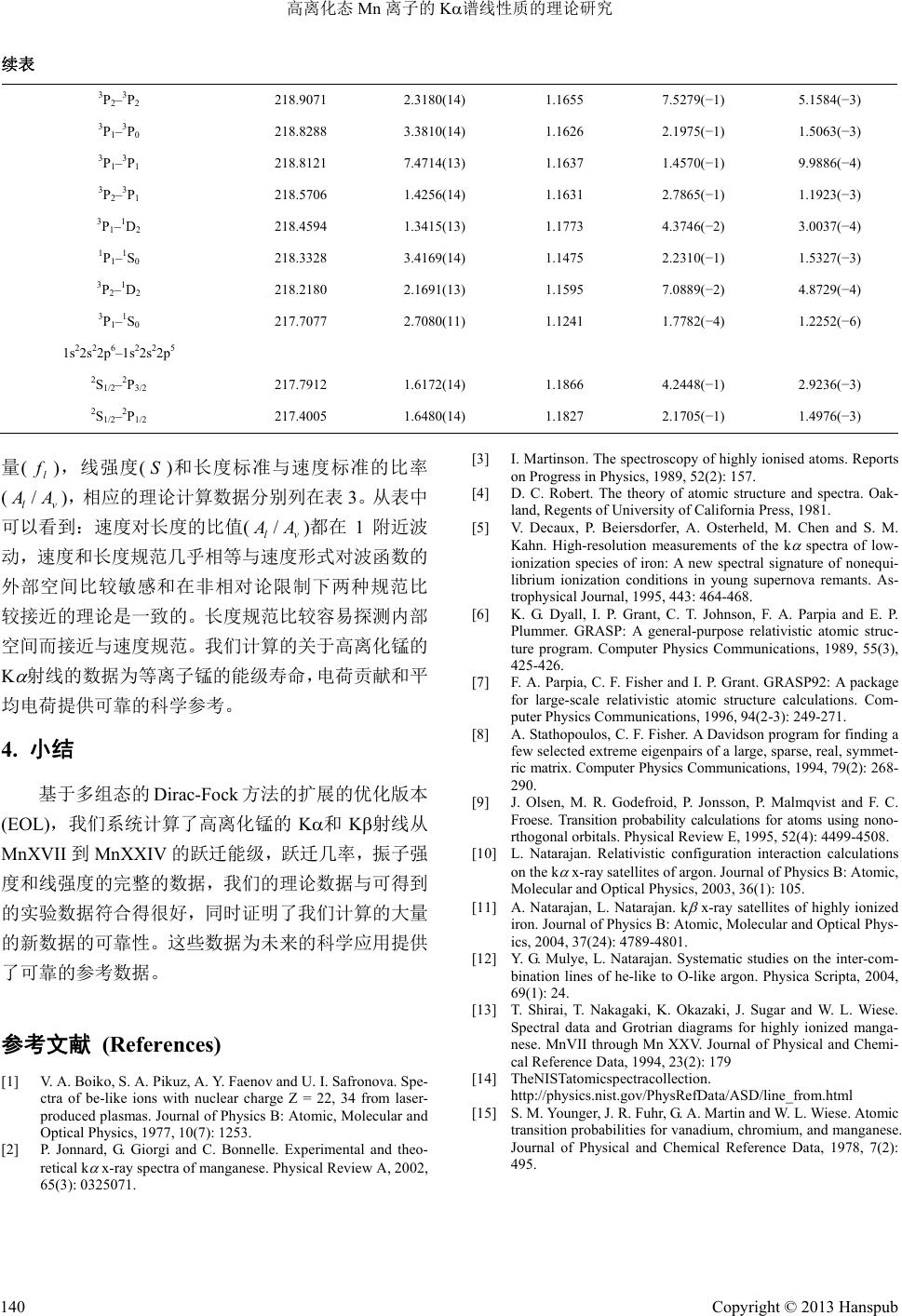

高离化态 Mn 离子的 K谱线性质的理论研究

140

续表

3P2–3P2 218.9071 2.3180(14) 1.1655 7.5279(−1) 5.1584(−3)

3P1–3P0 218.8288 3.3810(14) 1.1626 2.1975(−1) 1.5063(−3)

3P1–3P1 218.8121 7.4714(13) 1.1637 1.4570(−1) 9.9886(−4)

3P2–3P1 218.5706 1.4256(14) 1.1631 2.7865(−1) 1.1923(−3)

3P1–1D2 218.4594 1.3415(13) 1.1773 4.3746(−2) 3.0037(−4)

1P1–1S0 218.3328 3.4169(14) 1.1475 2.2310(−1) 1.5327(−3)

3P2–1D2 218.2180 2.1691(13) 1.1595 7.0889(−2) 4.8729(−4)

3P1–1S0 217.7077 2.7080(11) 1.1241 1.7782(−4) 1.2252(−6)

1s22s22p6–1s22s22p5

2S1/2–2P3/2 217.7912 1.6172(14) 1.1866 4.2448(−1) 2.9236(−3)

2S1/2–2P1/2 217.4005 1.6480(14) 1.1827 2.1705(−1) 1.4976(−3)

[3] I. Martinson. The spectroscopy of highly ionised atoms. Reports

on Progress in Physics, 1989, 52(2): 157.

量(l

)，线强度( )和长度标准与速度标准的比率

(

l[4] D. C. Robert. The theory of atomic structure and spectra. Oak-

land, Regents of University of California Press, 1981.

A)，相应的理论计算数据分别列在表3。从表中

可以看到：速度对长度的比值(/

A)都在 1附近波

动，速度和长度规范几乎相等与速度形式对波函数的

外部空间比较敏感和在非相对论限制下两种规范比

较接近的理论是一致的。长度规范比较容易探测内部

空间而接近与速度规范。我们计算的关于高离化锰的



射线的数据为等离子锰的能级寿命，电荷贡献和平

均电荷提供可靠的科学参考。

[5] V. Decaux, P. Beiersdorfer, A. Osterheld, M. Chen and S. M.

Kahn. High-resolution measurements of the k



spectra of low-

ionization species of iron: A new spectral signature of nonequi-

librium ionization conditions in young supernova remants. As-

trophysical Journal, 1995, 443: 464-468.

[6] K. G. Dyall, I. P. Grant, C. T. Johnson, F. A. Parpia and E. P.

Plummer. GRASP: A general-purpose relativistic atomic struc-

ture program. Computer Physics Communications, 1989, 55(3),

425-426.

[7] F. A. Parpia, C. F. Fisher and I. P. Grant. GRASP92: A package

for large-scale relativistic atomic structure calculations. Com-

puter Physics Communications, 1996, 94(2-3): 249-271.

[8] A. Stathopoulos, C. F. Fisher. A Davidson program for finding a

few selected extreme eigenpairs of a large, sparse, real, symmet-

ric matrix. Computer Physics Communications, 1994, 79(2): 268-

290.

4. 小结

基于多组态的 Dirac-Fock 方法的扩展的优化版本

(EOL)，我们系统计算了高离化锰的 K和K射线从

MnXVII 到MnXXIV 的跃迁能级，跃迁几率，振子强

度和线强度的完整的数据，我们的理论数据与可得到

的实验数据符合得很好，同时证明了我们计算的大量

的新数据的可靠性。这些数据为未来的科学应用提供

了可靠的参考数据。

[9] J. Olsen, M. R. Godefroid, P. Jonsson, P. Malmqvist and F. C.

Froese. Transition probability calculations for atoms using nono-

rthogonal orbitals. Physical Review E, 1995, 52(4): 4499-4508.

[10] L. Natarajan. Relativistic configuration interaction calculations

on the k



x-ray satellites of argon. Journal of Physics B: Atomic,

Molecular and Optical Physics, 2003, 36(1): 105.

[11] A. Natarajan, L. Natarajan. k



x-ray satellites of highly ionized

iron. Journal of Physics B: Atomic, Molecular and Optical Phys-

ics, 2004, 37(24): 4789-4801.

[12] Y. G. Mulye, L. Natarajan. Systematic studies on the inter-com-

bination lines of he-like to O-like argon. Physica Scripta, 2004,

69(1): 24.

[13] T. Shirai, T. Nakagaki, K. Okazaki, J. Sugar and W. L. Wiese.

Spectral data and Grotrian diagrams for highly ionized manga-

nese. MnVII through Mn XXV. Journal of Physical and Chemi-

cal Reference Data, 1994, 23(2): 179

参考文献 (References)

[14] TheNISTatomicspectracollection.

http://physics.nist.gov/PhysRefData/ASD/line_from.html

[1] V. A. Boiko, S. A. Pikuz, A. Y. Faenov and U. I. Safronova. Spe-

ctra of be-like ions with nuclear charge Z = 22, 34 from laser-

produced plasmas. Journal of Physics B: Atomic, Molecular and

Optical Physics, 1977, 10(7): 1253.

[15] S. M. Younger, J. R. Fuhr, G. A. Martin and W. L. Wiese. Atomic

transition probabilities for vanadium, chromium, and manganese.

Journal of Physical and Chemical Reference Data, 1978, 7(2):

495.

[2] P. Jonnard, G. Giorgi and C. Bonnelle. Experimental and theo-

retical k



x-ray spectra of manganese. Physical Review A, 2002,

65(3): 0325071.