Pure Mathematics
Vol.
11
No.
11
(
2021
), Article ID:
46265
,
11
pages
10.12677/PM.2021.1111200
线性多智能体系统的自适应动态事件触发一致性
田昌源,刘开恩,崔丹丹
青岛大学数学与统计学院,山东 青岛
收稿日期:2021年9月24日;录用日期:2021年10月27日;发布日期:2021年11月3日

摘要
本文研究了一般线性多智能体系统的自适应动态事件触发一致性问题。通过提出一种分布式自适应事件触发控制协议,有效避免了对网络拓扑全局信息的依赖,降低了通信频率。在给出的事件触发条件中,为每个智能体引入一个辅助函数来动态调节其阈值。与传统的静态阈值相比,动态阈值能够保证更少的事件触发时刻。首先,通过模型变换,将多智能体系统的一致性问题转化为相应误差系统的稳定性问题。进一步,利用代数图论和Lyapunov稳定性理论,得到了多智能体系统达到一致的充分性判据。同时,证明了在所提出的事件触发条件下,能够有效地排除Zeno行为。最后,通过仿真实例验证了理论结果的有效性。
关键词
多智能体系统,一致性,自适应控制,动态事件触发控制,一般线性动态

Adaptive Dynamic Event-Triggered Consensus of Linear Multi-Agent Systems
Changyuan Tian, Kai’en Liu, Dandan Cui
School of Mathematics and Statistics, Qingdao University, Qingdao Shandong
Received: Sep. 24th, 2021; accepted: Oct. 27th, 2021; published: Nov. 3rd, 2021

ABSTRACT
This paper is concerned with adaptive dynamic event-triggered consensus of general linear multi-agent systems. The dependence on the global information of network topology can be avoided and the communication frequency can be reduced by proposing a distributed adaptive event-triggered control protocol. In the event-triggered condition, an auxiliary function is introduced for each agent to regulate its threshold dynamically. Compared with the traditional static threshold, the dynamic one can ensure less event-triggered instants. First, by model transformation, the consensus problem of multi-agent systems is transformed into the stability problem of the corresponding error systems. Furthermore, by using algebraic graph theory and Lyapunov stability theory, some sufficient criteria for multi-agent systems to achieve consensus are obtained. Meanwhile, it is proved that the proposed event-triggered conditions can exclude Zeno behavior effectively. One simulation example is provided to validate the effectiveness of obtained theoretical results.
Keywords:Multi-Agent Systems, Consensus, Adaptive Control, Dynamic Event-Triggered Control, General Linear Dynamics

Copyright © 2021 by author(s) and Hans Publishers Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY 4.0).
http://creativecommons.org/licenses/by/4.0/


1. 引言
近年来,多智能体系统的协同控制问题受到了不同领域研究学者的广泛关注 [1] [2]。一致性问题是多智能体系统研究中最重要和最基本的一个问题,即通过设计恰当的分布式控制协议,使系统中的智能体就某些关键量趋于相同。目前,关于一致性问题的研究已经取得了许多成果 [3] [4]。然而,许多工作都假设智能体可以连续地获取其邻居的状态信息,并进行连续地控制更新,这往往需要较高的通信成本,因此这种方式与实际应用相距甚远。一些改进的控制机制已在最近的一些工作中得到应用,如周期采样控制和事件触发控制 [5] [6]。与周期采样控制相比,事件触发控制在采样时刻的选择上更加灵活。在事件触发控制中,只有在特定事件发生时才需要进行系统通信和控制更新,可以有效地降低系统能耗。因此,该控制机制已成为一个热门的研究课题 [7] [8] [9]。在 [10] [11] 中,针对一阶多智能体系统,提出了基于时滞的周期事件触发控制协议。文献 [12] 通过采用Artstein-Kwon-Pearson方法将具有时滞的多智能体系统转化为无时滞的多智能体系统,针对无时滞的一般线性多智能体系统,基于事件触发控制协议,分别研究了无领导者和领导–跟随一致性问题。
在一些关于高阶多智能体系统一致性问题的研究中,都需要用到Laplacian矩阵特征值的信息。然而,Laplacian矩阵依赖于整个通信拓扑,这意味着所提出的一致性协议不是完全分布式的。文献 [13] 针对一般线性和Lipschitz非线性两种情况,提出了自适应分布式一致性协议,用于调整相邻智能体之间的耦合权重。文献 [14] 通过提出自适应滑模协议,研究了具有外部干扰的多智能体系统的一致性问题。在实际应用中,将事件触发控制与自适应控制相结合,不仅可以节省通信资源,而且还能消除对全局信息的依赖。文献 [15] 和 [16] 分别研究了基于分布式自适应事件触发机制的二阶和一般线性多智能体系统的一致性。文献 [17] 通过设计自适应事件触发控制协议,系统地研究了线性多智能体系统在有向图上的一致性。
另一方面,上述所提到文献 [10] - [17] 中的事件触发阈值均是状态依赖的。随着时间的推移,由于阈值越来越小,则会出现事件频繁触发的情形,导致产生一些不必要的触发。在 [18] 中,通过在事件触发条件中引入动态参数,研究了一阶多智能体系统的分布式事件触发和自触发的一致性问题。文献 [19] 研究了动态事件触发机制下一般线性多智能体系统的领导–跟随一致性问题。文献 [20] 研究了一般线性多智能体系统在固定拓扑和切换拓扑下的动态事件触发一致性问题。以上所给结果表明,动态参数在排除Zeno行为方面起着至关重要的作用。文献 [21] 基于动态事件触发机制,研究了一般线性多智能体系统自适应一致性,既进一步降低通信成本和控制更新次数,又消除了对全局信息的依赖。
基于上述工作,本文进一步研究一般线性多智能体系统的自适应动态事件触发一致性。设计了完全分布式的自适应动态事件触发一致性协议,避免了对全局信息的依赖,进一步降低了通信成本和控制更新次数。理论分析表明,动态事件触发阈值可以有效排除Zeno行为。
本文的其余部分如下。第1节给出了预备知识并对所研究问题进行了陈述。第2节讨论了自适应动态事件触发机制下的一般线性多智能体系统一致性问题,在给定的事件触发条件下,得出系统实现一致的充分条件。在第3节中,通过数值仿真验证了理论分析的有效性。最后,第4节对本文进行了总结并对未来拟进一步研究的方向进行了展望。
2. 预备知识和问题陈述
2.1. 预备知识
多智能体系统之间的通信拓扑可以描述为一个无向图 ,其中 表示顶点集, 表示边集。当 时,节点i和节点j称作邻居,这意味着智能体i和j能够互相通信。从节点 到节点 的路径是 ,,形式的相邻边序列。如果任意一对不同的节点之间存在一条路径,则称无向图是连通的。 为 邻接矩阵,其中 当且仅当 ,否则 。在本文中,我们假定 ,因此 。 表示节点i的邻居集。 表示度矩阵,其中 是节点i的入度。图 的Laplacian矩阵定义为 。本文将对称矩阵的特征值按递增的顺序排列,即对于矩阵L, 。由L的定义可得 。进一步,如果 是连通的,则 。
符号说明: 和 分别表示n维实向量和 维实矩阵的集合, 表示N维单位矩阵,0表示具有适当维数的所有元素为0的列向量。 表示Kronecker积。 表示向量的欧几里德范数或矩阵的向量诱导2-范数。对于实对称矩阵A, 意味着A是正定的。
2.2. 问题陈述
考虑一个由N个智能体组成的多智能体系统,第i个智能体的动态描述为
(1)
其中 和 是常值矩阵, 和 分别是智能体i的状态和控制输入。如果 ,其中 ,则称系统(1)可以达到一致。
在进一步分析之前我们提出以下假设。
假设1 是可镇定的。
在假设1下,对于任意正定矩阵Q,存在一个正定矩阵P,满足以下代数Riccati方程
(2)
3. 主要结果
在本节中,针对系统(1),提出一种分布式自适应动态事件触发控制协议,研究给出保证(1)一致性成立的条件。定义 为 的状态估计,其中 , 表示智能体i的第k个事件触发时刻且 。智能体i的状态误差定义为 ,。对于每个智能体,提出了以下分布式自适应事件触发一致性协议
(3)
(4)
其中 和 是反馈增益矩阵, 表示边 的时变耦合权值且 ,。注意,如果节点j不是节点i的邻居,那么(4)中 相关的项不出现。
对每一个智能体,设计如下动态事件触发函数,
(5)
(6)
其中 ,, 是一个辅助变量且 ,,。事件触发时刻定义为 。
注1 在(5)中, 是时变的,其更新规律与状态误差和相邻状态估计误差有关。与静态事件触发策略相比,在(5)中 是动态调节阈值的关键因素。如果将 设为零,则(5)中的事件触发函数变为静态函数,可视为其特例。
注2 在事件触发机制下,连续两个触发时刻 和 之间不触发任何事件。因此我们有 ,即 。通过递推,我们有 ,。因此,对于 ,可得 。相比静态情况,动态事件触发机制(5)中的触发条件更灵活。
将分布式控制协议(3)和(4)代入(1)中,可得
(7)
定义 ,其中 。根据(1)和(3),可以得到 满足以下动态
(8)
因此,系统的(1)的一致性问题能够等价地转换为误差系统(8)的稳定性问题。
定理1 假设图 是连通的。令 和 ,其中 是(2)的解。在协议(3)和(4)作用下,如果(6)中 满足 ,那么多智能体系统(1)能够达到一致。此外,每个耦合权值 均收敛到某个有限的稳态值。
证明选取以下Lyapunov函数
其中 ,,, 是稍后设计的常数。
计算 关于时间的导数为
(9)
根据Young不等式 [22] 可得
(10)
和
(11)
将(10)和(11)代入(9)中可得
(12)
计算 关于时间的导数为
(13)
结合(12)和(13)以及 的定义可得
(14)
注意到
(15)
类似于(10),不难得出
(16)
类似于(11)可得
(17)
将(15)~(17)代入(14)中,结合 的定义可得
(18)
设 ,结合事件触发条件,由(18)可得
(19)
其中 为Q的最小特征值。由Riccati方程(2)可得 ,因此 。设 ,其中 。易见 意味着 ,。因此,根据LaSalle不变原理,当 时, ,这意味着多智能体系统(1)可以达到一致。
因为 ,所以 和 是有界的。结合 是不减的,可得每一个耦合权值 都收敛到某个有限的稳态值。
定理2 在定理1的条件下,多智能体系统(1)不存在Zeno行为。
证明通过(1)和(3)可得
计算 在 区间上的导数
(20)
假设多智能体系统(1)存在Zeno行为,则至少存在一个智能体i满足 ,其中 是一个正数并且 。由定理1可得 是有界的。不失一般性,假设 ,其中 是一个正数。由(20)可知
其中 。考虑一个非负函数 满足
(21)
根据比较原理 [23] 可得 ,其中 是(21)的解。由(5)可知如果 ,则有 。通过求解 从0到 所演化的时间,不难看出两个事件触发时刻 和 之间间隔 的下界 可以通过解以下方程得到
上述方程等价于
(22)
设 ,由(22)可得 。根据数列极限的定义,存在正整数 ,对于任意的 ,使得 成立。因此 ,这与事实 相矛盾。因此Zeno行为被排除。
4. 数值仿真
在本节中,通过仿真实例来验证所得结果的有效性。考虑由(1)描述的一般线性多智能体系统,其中
设(2)中 ,求解(2)给出(4)和(5)中的反馈增益矩阵为
假设智能体之间的通信拓扑如图1所示且所有智能体的初始值都是随机的,显然图1是连通的。在(4)中,设 ,对于任意的 , 随机抽取。设(5)和(6)中的参数 ,,,,且 随机选取,其中 。
Figure 1. Communication topology among agents
图1. 智能体间的通信拓扑
所有智能体的事件触发时刻如图2所示。状态误差 如图3所示,其中 ,由此可以得出多智能体系统(1)渐近实现一致性。耦合权值 的演变状态如图4所示,它们分别收敛于有限稳态值。
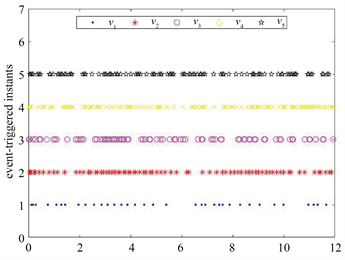
Figure 2. Event-triggered instants
图2. 事件触发时刻

Figure 3. State errors
图3. 状态误差
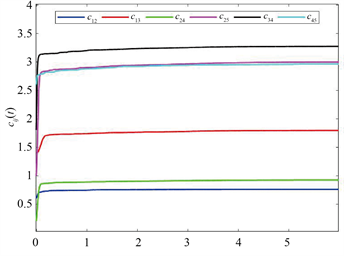
Figure 4. Adaptive coupling weights
图4. 自适应耦合权值
5. 结论
本文研究了基于动态事件触发的一般线性多智能体系统的自适应一致性问题。通过设计自适应动态事件触发协议,避免了在事件触发机制下对系统拓扑全局信息的依赖,并且有效降低了通信负担。通过理论分析表明,所提动态事件触发条件可以有效排除Zeno行为。数值仿真也验证了所得结果的有效性。今后的工作将研究具有切换拓扑的一般线性多智能体系统的一致性问题。
基金项目
这项工作由山东省自然科学基金项目(No. ZR202102180402)资助。
文章引用
田昌源,刘开恩,崔丹丹. 线性多智能体系统的自适应动态事件触发一致性
Adaptive Dynamic Event-Triggered Consensus of Linear Multi-Agent Systems[J]. 理论数学, 2021, 11(11): 1770-1780. https://doi.org/10.12677/PM.2021.1111200
参考文献
- 1. Savkin, A.V., Wang, C., Baranzadeh, A., Xi, Z.Y. and Nguyen, H. (2016) Distributed Formation Building Algorithms for Groups of Wheeled Mobile Robots. Robotics and Autonomous Systems, 75, 463-474. https://doi.org/10.1016/j.robot.2015.08.006
- 2. Ji, Z.J., Lin, H., Cao, S.B., Qi, Q.Y. and Ma, H. (2021) The Complexity in Complete Graphic Characterizations of Multi-Agent Controllability. IEEE Transactions on Cybernetics, 51, 64-76. https://doi.org/10.1109/TCYB.2020.2972403
- 3. Wang, D.D., Zhou, Q.H. and Zhu, W. (2018) Adaptive Event-Based Consensus of Multi-Agent Systems with General Linear Dynamics. Journal of Systems Science & Com-plexity, 31, 120-129. https://doi.org/10.1007/s11424-018-7360-0
- 4. 叶丹, 张天予, 李奎. 全局信息未知的多智能体自适应容错包容控制[J]. 山东大学学报(工学版), 2017, 47(5): 1-6.
- 5. Cheng, L., Wang, Y.P., Hou, Z.G., Tan, M. and Cao, Z.Q. (2013) Sampled-Data Based Average Consensus of Second-Order Integral Multi-Agent Systems: Switching Topologies and Communication Noises. Automatica, 49, 1458-1464. https://doi.org/10.1016/j.automatica.2013.02.004
- 6. Dimrogonas, D.V., Frazzoli, E. and Johansson, K.H. (2012) Distributed Event-Triggered Control for Multi-Agent Systems. IEEE Transactions on Automatic Control, 57, 1291-1297. https://doi.org/10.1109/TAC.2011.2174666
- 7. Fan, Y., Feng, G., Wang, Y. and Song C. (2013) Distributed Event-Triggered Control of Multi-Agent Systems with Combinational Measurements. Automatica, 49, 671-675. https://doi.org/10.1016/j.automatica.2012.11.010
- 8. Shang, Y.L. (2018) Fixed-Time Group Consensus for Multi-Agent Systems with Non-Linear Dynamics and Uncertainties. IET Control Theory & Applications, 12, 395-404. https://doi.org/10.1049/iet-cta.2017.1021
- 9. Li, X.D, Peng, D.X. and Cao, J.D. (2020) Lyapunov Stability for Impulsive Systems via Event-Triggered Impulsive Control. IEEE Transactions on Automatic Control, 65, 4908-4913. https://doi.org/10.1109/TAC.2020.2964558
- 10. Liu, K.E. and Ji, Z.J. (2017) Consensus of Multi-Agent Systems with Time Delay Based on Periodic Sample and Event Hybrid Control. Neurocomputing, 270, 11-17. https://doi.org/10.1016/j.neucom.2016.12.106
- 11. Liu, K.E., Ji, Z.J. and Zhang, X.F. (2020) Periodic Event-Triggered Consensus of Multi-Agent Systems Under Directed Topology. Neurocomputing, 385, 33-41. https://doi.org/10.1016/j.neucom.2019.12.081
- 12. Ai, X.L. and Wang, L. (2021) Distributed Fixed-Time Event-Triggered Consensus of Linear Multi-Agent Systems with Input Delay. International Journal of Robust and Nonlinear Control, 31, 2526-2545. https://doi.org/10.1002/rnc.5404
- 13. Li, Z.K., Ren, W., Liu, X.D. and Fu, M.Y. (2013) Consensus of Multi-Agent Systems with General Linear and Lipschitz Non-Linear Dynamics Using Distributed Adaptive Protocols. IEEE Transactions on Automatic Control, 58, 1786-1791. https://doi.org/10.1109/TAC.2012.2235715
- 14. Yu, Z.Y., Yu, S.Z., Jiang, H.J. and Hu, C. (2021) Distributed Consensus for Multi-Agent Systems via Adaptive Sliding Mode Control. International Journal of Robust and Nonlinear Control, 31, 7125-7151. https://doi.org/10.1002/rnc.5670
- 15. Zhu, W., Zhou, Q.H., Wang, D.D. and Feng, G. (2018) Fully Distributed Consensus of Second-Order Multi-Agent Systems Using Adaptive Event-Based Control. Science China Information Sciences, 61, Article No. 129201. https://doi.org/10.1007/s11432-018-9510-2
- 16. Cheng, B. and Li, Z.K. (2019) Fully Distributed Event-Triggered Protocols for Linear Multi-Agent Networks. IEEE Transactions on Automatic Control, 64, 1655-1662. https://doi.org/10.1109/TAC.2018.2857723
- 17. Li, X.W., Sun, Z.Y., Tang, Y. and Karimi, H.R. (2021) Adaptive Event-Triggered Consensus of Multi-Agent Systems on Directed Graphs. IEEE Transactions on Automatic Control, 66, 1670-1685. https://doi.org/10.1109/TAC.2020.3000819
- 18. Yi, X.L., Liu, K., Dimarogonas, D.V. and Johansson, K.H. (2019) Dynamic Event-Triggered and Self-Triggered Control for Multi-Agent Systems. IEEE Transactions on Automatic Control, 64, 3300-3307. https://doi.org/10.1109/TAC.2018.2874703
- 19. Du, S.L., Liu, T. and Ho, D.W.C. (2020) Dynamic Event-Triggered Control for Leader-Following Consensus of Multi-Agent Systems. IEEE Transactions on Systems, Man, and Cybernetics: Systems, 50, 3243-3251. https://doi.org/10.1109/TSMC.2018.2866853
- 20. Li, Y.F., Liu, X.D., Liu, H.K., Du, C.K. and Lu, P.L. (2021) Distributed Dynamic Event-Triggered Consensus Control for Multi-Agent Systems under Fixed and Switching Topol-ogies. Journal of the Franklin Institute, 358, 4348-4372. https://doi.org/10.1016/j.jfranklin.2021.03.027
- 21. He, W.L., Xu, B., Han Q.L. and Qian F. (2020) Adaptive Consensus Control of Linear Multi-Agent Systems with Dynamic Event-Triggered Strategies. IEEE Transactions on Cybernetics, 50, 2996-3008. https://doi.org/10.1109/TCYB.2019.2920093
- 22. Bernstein, D.S. (2009) Matrix Mathematics: Theory, Facts, and Formulas. Princeton University Press, Princeton. https://doi.org/10.1515/9781400833344
- 23. Li, Z.K. and Duan Z. (2017) Cooperative Control of Multi-Agent Systems: A Consensus Region Approach. CRC Press, Boca Raton. https://doi.org/10.1201/b17571