Advances in Applied Mathematics
Vol.
08
No.
04
(
2019
), Article ID:
29992
,
11
pages
10.12677/AAM.2019.84092
Oscillation Criteria for a Class of Second-Order Mixed Nonlinear Differential Equations
Jinying Lin, Tengjie Chen
School of Mathematics and Big Data, Huizhou University, Huizhou Guangdong
Received: Apr. 6th, 2019; accepted: Apr. 21st, 2019; published: Apr. 28th, 2019

ABSTRACT
New oscillation criteria for a class of second-order mixed nonlinear damping equations are obtained by means of the integral averaging technique and a new kernel function combined with the Elure integral. The new results have a higher generality than some of previous results. The zero distribution information of the solution is also obtained.
Keywords:Oscillation, Second-Order, Differential Equations, Mixed Nonlinearities, Riccati Transform
一类二阶混合非线性微分方程的 振动准则
林锦滢,陈腾杰
惠州学院,数学与大数据学院,广东 惠州
收稿日期:2019年4月6日;录用日期:2019年4月21日;发布日期:2019年4月28日

摘 要
运用积分平均技巧和新型的核函数,结合Elure积分,得到一类二阶混合非线性阻尼方程的新振动准则。新的结果具有更高的一般性,并能得到解的零点分布信息。
关键词 :振动性,二阶,微分方程,混合非线性,Riccati变换

Copyright © 2019 by authors and Hans Publishers Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/


1. 引言
本文考虑如下二阶含阻尼项的混合型非线性微分方程
(1)
的振动性,其中
,
是一个满足
的n元数组,其中
。
一般地,若函数
满足方程(1),则称
是方程(1)的一个解。我们总假设方程(1)在区间
满足解的存在唯一性。如果方程(1)的一个解
在
存在任意大的零点,则称
为振动的,否则称为非振动的。
已有大量文献研究了方程(1)的特殊情形,如(见 [1] - [6] 及其中的参考文献)
(2)
(3)
大多数著名的振动准则都关系到f和q在区间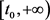 上的积分,这使得这些结果很难被应用到更加一般的情形。
上的积分,这使得这些结果很难被应用到更加一般的情形。
1999年,Wong [6] 和Kong [7] 分别指出,Ei-Sayed [4] 为方程(2)建立的区间振动准则中,由于其证明过程需要与一个常系数方程做比较而使得其结果的应用受到限制。于是,Wong [6] 和Kong [7] 分别建立方程(2)和方程(3)在
的情形下的区间振动准则。2003年,Yang [8] 应用Philos [9] 和Kong [7] 在方程(3)中使用的技巧,给出了几个区间振动准则。其中一个Kamenevs类型的振动准则如下:
定理1:假设
,
,
,
。则方程(3)当
时是振动的,如果
,
,使得下列结论成立:
(A1) 对
,
使得
(A2) 存在
,使得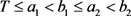 下列不等式成立
下列不等式成立
(4)
(5)
在2004年,Sun [10] 用一个新的核函数得出了以下定理。
定理2:方程(1)当
且
时是振动,如果存在一个常数
使得下式成立
(6)
另外一些类似的结果可以在Dube和Mingare [11] 中找到。受 [8] [10] [11] [12] 工作的启发,本文利用一个由Yang [13] [14] 首次介绍的核函数
,建立方程(1)的有关Elure积分的新的振动准则。我们的结果将改进和推广Sun [10] 和Yang [8] 的结果。最后,我们给出一个例子说明新的振动准则的应用。
作为准备,我们先给出函数类
的定义和需要用到的引理。
定义1:令
,
,
,称一个实值函数H属于
,表示为
。如果存在一个函数
满足以下条件:
(H1) 在E上
,
,且在
上
。
(H2)
在
上对第二个变量存在连续的偏导函数,且存在
,满足
引理1:设
是一个满足
的n元数组,其中
。则存在一个n元数组
,使得
i)
,
ii)
。
2. 主要结论
定理2.1:假设
使得
(7)
若存在一个函数
,使得
(8)
其中
,
或
。则方程(1)是振动的。
证明:假设方程(1)存在非振动解
,无妨设
。我们定义如下函数:
(9)
由方程(1)和(9)式,对于
我们有
(10)
由算术几何平均不等式:
(11)
其中,
,
,我们令
,记
(12)
(13)
我们有
(14)
因此
(15)
根据假设,选择
,
使得在区间
上,
,得到
。 (16)
方程(16)两边同时乘
,将t用s替代,并将它们从
到
积分,通过简单计算我们可以得到
(17)
这就意味着
(18)
这与(8)式矛盾,从而
至少有一个零解
。
当
时,我们可以选择
,使得在区间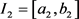 上
,那么就得到类似上述的矛盾。所以
至少有一个零解
。
上
,那么就得到类似上述的矛盾。所以
至少有一个零解
。
注意到T是任意的,于是可得方程的每个解都有任意大的零点。因此方程(1)是振动的。定理2.1证毕。
令
,其中
,根据定理2.1,我们有以下的振动结论。
定理2.2:假设任意
,存在
,使得(7)式成立。如果存在两个常数
,使得
(19)
其中
,
或
,则方程(1)是振动的。
定义
令
,其中
,则有
根据定理2.1,我们有以下的振动结论。
定理2.3:假设任意
,存在
,使得(7)式成立。如果存在两个常数
,使得
(20)
其中
,
或
,则方程(1)是振动的。
定理2.4:设
。且对任意的
,存在
使得(7)式成立。如果存在两个常数
,使得
(21)
其中
,
或
,则方程(1)是振动的。
证明:
(22)
其中,设
。使用Euler公式
且
设
我们可以得到,
(23)
(24)
(25)
因此
(26)
将(26)式代入(22),我们有
(27)
于是
(28)
由(21)和(28)式,得到
因此,依据定理2.2方程(1)是振动的。定理2.4证毕。
推论2.1:设
。且对任意的
,存在
使得(7)式成立。如果存在一个常数
使得
(29)
其中
,
或
,则方程(1)是振动的。
证明:在(21)中分别用
和2替代
和
,可以得到
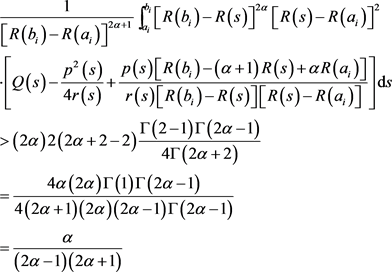 (30)
(30)
推论2.2:设
。且对任意的
,存在
使得(7)式成立。如果存在一个常数
有
(31)
其中
,
或
,则方程式(1)是振动的。
如果假定
,注意
根据定理2.1~2.4。我们可以得到以下定理。
定理2.5:设
成立。且对任意
,存在
使得(7)式成立。如果存在函数
,使得
(32)
其中
,
或
,则方程式(1)振动的。
定理2.6:设
且对任意的
,存在
使得(7)式成立。如果存在两个常数
,使得
 (33)
(33)
其中
,
或
,则方程式(1)是振动的。
定理2.7:设
,
,且对任意的
,存在
使得(7)是成立。如果存在两个常数
,使得
(34)
其中
,
或
,则方程式(1)是振动的。
推论2.3:设
,
。且对任意的
,存在
使得(7)式成立。如果存在常数
使得
(35)
其中
,
或
,则方程式(1)是振动的。
推论2.4:设
,
。且对任意的
,存在
使得(7)式成立。如果存在常数
,使得
(36)
其中
,
或
,则方程式(1)是振动的。
3. 例子
考虑二阶非线性方程
(37)
易证明方程(37)在
上是振动的。事实上,让
,我们选择
则
再令
,很容易得到
于是有
由定理2.6知方程(31)是振动的。
致谢
本文工作受到了惠州学院重点重大研究培育项目(hzu201705)及广东省大学生科技创新培育专项项目的资助。
文章引用
林锦滢,陈腾杰. 一类二阶混合非线性微分方程的振动准则
Oscillation Criteria for a Class of Se-cond-Order Mixed Nonlinear Differential Equations[J]. 应用数学进展, 2019, 08(04): 815-825. https://doi.org/10.12677/AAM.2019.84092
参考文献
- 1. Kartsatos, A.G. (1972) Maintenance of Oscillations under the Effect of a Periodic Forcing Term. Proceedings of the American Mathematical Society, 33, 377-383. https://doi.org/10.1090/S0002-9939-1972-0330622-0
- 2. Wong, J.S.W. (1988) Second Order Nonlinear Forced Oscillations. SIAM Journal on Mathematical Analysis, 19, 667-675. https://doi.org/10.1137/0519047
- 3. Elbert, A. (1998) Oscillation/Nonoscillation for Linear Second Order Dif-ferential Equation. Journal of Mathematical Analysis and Applications, 226, 207-219. https://doi.org/10.1006/jmaa.1998.6059
- 4. Ei-Sayed, M.A. (1993) An Oscillation Criteria for a Forced Se-cond-Order Linear Differential Equation. Proceedings of the American Mathematical Society, 118, 813-817. https://doi.org/10.2307/2160125
- 5. Nasr, A.H. (1998) Sufficient Conditions for the Oscillation of Forced Su-per-Linear Second Order Differential Equation with Oscillatory Potential. Proceedings of the American Mathematical Society, 126, 123-125.
https://doi.org/10.1090/S0002-9939-98-04354-8
- 6. Wong, J.S.W. (1999) Oscillation Criteria for a Forced Se-cond Order Linear Differential Equation. Journal of Mathematical Analysis and Applications, 231, 235-340. https://doi.org/10.1006/jmaa.1998.6259
- 7. Kong, Q. (1999) Interval Criteria for Oscillation of Second Order Linear Differential Equations. Journal of Mathematical Analysis and Applications, 229, 258-270. https://doi.org/10.1006/jmaa.1998.6159
- 8. Yang, Q. (2003) Interval Oscillation Criteria for a Forced Second Order Nonlinear Ordinary Differential Equations with Oscillatory Potential. Applied Mathematics and Computation, 135, 49-64.
https://doi.org/10.1016/S0096-3003(01)00307-1
- 9. Philos, Ch.G. (1989) Oscillation Theorems for Linear Dif-ferential Equations of Second Order. Archiv der Mathematik (Basel), 53, 483-492. https://doi.org/10.1007/BF01324723
- 10. Sun, Y.G. (2004) New Kamenev-Type Oscillation Criteria for Se-cond-Order Nonlinear Differential Equations with Damping. Journal of Mathematical Analysis and Applications, 291, 341-351.
https://doi.org/10.1016/j.jmaa.2003.11.008
- 11. Dube, S.G.A. and Mingarelli, A.B. (2005) Nonlinear Functions and a Theorem of Sun. Journal of Mathematical Analysis and Applications, 308, 208-220. https://doi.org/10.1016/j.jmaa.2004.11.060
- 12. Zhuang, R.K. and Wu, H.W. (2011) New Oscillation Criteria Related to Euler’s Integral for Certain Nonlinear Differential Equation. Annals of Differential Equations, 27, 111-118.
- 13. Yang, Q.G. (2004) Oscillation of Self-Adjoint Matrix Hamiltonian Systems. Journal of Mathematical Analysis and Applications, 296, 110-130. https://doi.org/10.1016/j.jmaa.2004.03.052
- 14. Yang, Q.G. (2007) On the Oscillation of Certain Nonlinear Neutral Partial Differential Equations. Applied Mathematics Letters, 20, 900-907. https://doi.org/10.1016/j.aml.2006.11.001





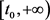 上的积分,这使得这些结果很难被应用到更加一般的情形。
上的积分,这使得这些结果很难被应用到更加一般的情形。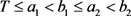 下列不等式成立
下列不等式成立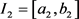 上
,那么就得到类似上述的矛盾。所以
至少有一个零解
。
上
,那么就得到类似上述的矛盾。所以
至少有一个零解
。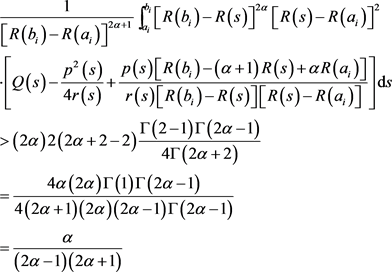 (30)
(30) (33)
(33)