Pure Mathematics
Vol.
13
No.
12
(
2023
), Article ID:
78611
,
10
pages
10.12677/PM.2023.1312379
度量与三角比度量的精确比较及其应用
宋星晨,王根娣
浙江理工大学理学院,浙江 杭州
收稿日期:2023年11月14日;录用日期:2023年12月15日;发布日期:2023年12月29日

摘要
本文研究了一个与边界相关的双曲型度量 和三角比度量的精确比较关系。应用该关系,得到了 度量与Cassini度量、双曲度量和距离比度量之间的比较不等式。特别地,证明了这些不等式的精确性。此外,还通过 度量与三角比度量之间的比较关系得到了在 度量下bi-Lipschitz映射的拟共形性。
关键词
度量,三角比度量,度量比较,拟共形性

Sharp Comparison between the Metric and the Triangular Ratio Metric and Its Applications
Xingchen Song, Gendi Wang
School of Science, Zhejiang Sci-Tech University, Hangzhou Zhejiang
Received: Nov. 14th, 2023; accepted: Dec. 15th, 2023; published: Dec. 29th, 2023

ABSTRACT
This article investigates the sharp comparison between a boundary-related hyperbolic type metric and the triangular ratio metric. By applying this relationship, comparisons between the metric and the Cassinian metric, the hyperbolic metric, and the distance ratio metric are obtained. In particular, we show the accuracy of these inequalities. Additionally, the quasi-conformality of the bi-Lipschitz mapping under the metric is also obtained by comparing it with the triangular ratio metric.
Keywords: Metric, Triangular Ratio Metric, Metric Comparison, Quasi-Conformality

Copyright © 2023 by author(s) and Hans Publishers Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY 4.0).
http://creativecommons.org/licenses/by/4.0/


1. 引言
双曲度量对于推动几何函数论的发展有很大的意义,然而在高维空间中双曲度量存在着局限性,因此引入了双曲型度量。常见的双曲型度量有三角比度量、Cassini度量、距离比度量等。在双曲型度量研究中,关于双曲度量与双曲型度量之间的比较是很有意义的。此外,双曲型度量在共形映射、拟共形映射下的不变性和偏差性在双曲型度量几何学中也有着重要作用。
近期,Song和Wang引入了一个与边界相关的双曲型度量 (即度量的大小与区域边界上的点有关),并研究了在 子域上它和双曲度量、双曲型度量之间的球包含关系;单位球内Möbius变换在 度量下的偏差性等几何性质 [1] 。
本文,我们将研究 度量与三角比度量的精确比较及其应用。首先给出了在单位圆盘内特殊点处 度量的具体公式。此外,得到了 度量与三角比度量的精确比较,应用该比较关系获得了 度量与Cassini度量、双曲度量、距离比度量之间的比较关系,并证明了这些比较关系的精确性。特别地,由 度量与三角比度量之间的比较关系,得到了在 度量下bi-Lipschitz映射的拟共形性。
2. 预备知识
本文采用的符号如下: 表示实数集, 表示n维欧氏空间, 和 分别表示上半空间 和单位球 。开集 且 ,对任意 , 表示点x和点y之间的欧氏距离, 表示点x与D的边界 之间的距离。记 , 。
2.1. 度量
对任意 , 度量定义如下 [1] :
。
由 的定义可得它在单位圆盘内特殊点处的具体表达式,即下面的引理1。
引理1 对任意 ,且 , ,
a) 若 ,则
;
b) 若 ,则
。
证明 a) 若 ,则定义中的上确界可在 的边界上点p满足 处取得(见图1)。此时
。
b) 若 ,则定义中的上确界可在 的边界上点p满足 处取得(见图2)。此时
。
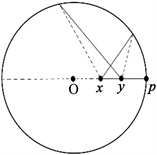
Figure 1. x and y are on the same side of O
图1. x,y在O的同侧

Figure 2. x and y are on the opposite sides of O
图2. x,y在O的同侧
引理1 在证明 度量和其它度量比较关系的精确性时起着重要作用。
下面我们介绍三角比度量和Cassini度量的定义,它们与 度量的定义具有一定的相似性。
2.2. 三角比度量
三角比度量由Hästö提出 [2] 。对任意 ,三角比度量定义如下:
。
Parisa等研究了关于三角比度量满足bi-Lipschitz条件映射的拟共形性 [3] 。Rainio等考虑了角形域内在拟共形映射下三角比度量的几何性质 [4] 。Chen等研究了三角比度量与双曲度量、双曲型度量之间的比较关系 [5] 。Jia等研究了三角比度量的上下界 [6] 。
2.3. Cassini度量
Ibragimov引入了Cassini度量 [7] 。对任意 ,Cassini度量定义如下:
。
Ibragimov研究了Cassini度量的测地性和等距性等几何性质 [7] 。Ibragimov等还考虑了在单位球内Cassini度量与双曲度量之间的比较关系 [8] 。Wu等研究了Cassini度量的上下界、Cassini度量和双曲度量之间的比较关系 [9] 。
2.4. 双曲度量
在上半空间 和单位球 上的双曲度量 和 的定义分别如下 [10] :
,对任意 ,
,对任意 。 (1)
由式(1)易得
, (2)
。 (3)
经典的双曲度量推动了几何函数论的发展,由于双曲度量在高维空间中的局限性,Gehring引入了距离比度量,并研究了它在高维拟共形映射下的偏差性质。
2.5. 距离比度量
Gehring等首先引入了距离比度量的原始定义 [11] ,下述形式则由Vuorinen提出 [12] 。对任意 ,距离比度量定义如下:
。
由 的定义,Parisa等研究了 [3] :
。
3. 与相关度量的比较
在本节,通过观察 度量与三角比度量的定义,我们首先得到了 度量与三角比度量之间的比较关系,并证明了比较关系的精确性。这为研究 度量与其它度量之间的比较关系以及 度量下bi-Lipschitz映射的拟共形性做了准备。
定理1 对任意 ,有
,
且不等式都是精确的。
证明 对任意 , ,显然有
,
。
由p的任意性,得
。
于是
。
为证明不等式的精确性,令 。
首先证明右边不等式的精确性。令 ,则
。
此时,有
。
其次证明左边不等式的精确性。令 , , ,则
。
此时,有
。
应用 度量与三角比度量之间的比较关系,我们得到了 度量与Cassini度量、双曲度量和距离比度量之间的比较不等式,并证明了比较不等式的精确性。
引理2 ( [13] ,定理1.3)对任意 ,有
。
当 时,不等式是精确的。
定理2 对任意 ,有
。
当 时,不等式是精确的。
证明 该不等式由定理1和引理2易得。为证明不等式的精确性,令 , , ,则由引理1和文献( [9] ,引理2(2))得
。
此时,有
。
引理3 ( [6] ,定理3) ( [11] ,引理2.6)对任意 ,有
,
且不等式都是精确的。
定理3 对任意 ,有
,
且不等式都是精确的。
证明 该不等式由定理1和引理3易得。下面证明不等式的精确性。
首先证明右边不等式的精确性。令 , , ,则由引理1和式(2)得
。
此时,有
。
其次证明左边不等式的精确性。令 , , ,则由引理1和式(2)得
, 。 (4)
由式(2)和式(4)得
。
此时,有
。
引理4 ( [3] ,引理2.1~2.2)对任意 ,有
,
且不等式是精确的。
定理4 对任意 ,有
,
且不等式是精确的。
证明 该不等式由定理1和引理4易得。为证明不等式的精确性,令 。
首先证明右边不等式的精确性。令 ,则
。
此时,有
。
其次证明左边不等式的精确性。令 , , ,则
。
此时,有
。
下面我们考虑在一个小球形域内的任意两点在大球形域内 度量和距离比度量之间的比较关系。
引理5 设 , ,对任意 ,有
。
证明 因为 ,所以 。
首先证明右边不等式。对任意 ,有
,
,
。
由p的任意性,得
。
其次证明左边不等式。对任意 ,有
由p的任意性,得
。
于是
。
引理6 设 , ,对任意 ,有
。
证明 因为 ,所以 。
首先证明右边不等式。因为当 时, ,所以
。
其次证明左边不等式。因为当 时, ,所以
。
于是
。
定理5 设 , ,对任意 ,有
。
证明 对任意 ,由引理5和引理6得
。
。
于是
。
4. 度量下bi-Lipschitz映射的拟共形性
Parisa等发现了关于三角比度量满足bi-Lipschitz条件的保向同胚映射是拟共形的 [3] 。在本节,我们利用 度量和三角比度量之的比较关系,获得了关于 度量满足bi-Lipschitz条件的保向同胚映射的拟共形性。
引理7 ( [3] ,定理4.4)设 , 是一个保向同胚映射,对任意 ,关于 满足L-bi-Lipschitz条件,即
,
则f是拟共形的,它的线性伸张系数 。
定理6 设开集 , 是一个保向同胚映射,对任意 ,关于 满足L-bi-Lipschitz条件,即
,
则f是拟共形的,它的线性伸张系数 。
证明 对任意 ,由定理1得
,
。
则f也是一个关于三角比度量满足2L-bi-Lipschitz条件的保向同胚映射,即
。
由引理7得f是拟共形的,它的线性伸张系数
。
5. 总结
度量是一个新引入的双曲型度量,目前关于它的研究结果很少,本文对 度量进行了以下两方面研究:
a) 研究了 度量与经典的三角比度量之间的精确比较关系,应用该比较关系得到 度量与其它相关度量之间的比较不等式,并证明了这些不等式的精确性。
b) 由 度量与三角比度量之间的比较关系,得到了在 度量下bi-Lipschitz映射的拟共形性。
文章引用
宋星晨,王根娣. C~度量与三角比度量的精确比较及其应用
Sharp Comparison between the C~ Metric and the Triangular Ratio Metric and Its Applications[J]. 理论数学, 2023, 13(12): 3653-3662. https://doi.org/10.12677/PM.2023.1312379
参考文献
- 1. Song, X.C. and Wang, G.D. (2023) A New Metric Associated with the Domain Boundary.
- 2. Hästö, P.A. (2002) A New Weighted Metric: The Relative Metric I. Journal of Mathematical Analysis and Applications, 274, 38-58. https://doi.org/10.1016/S0022-247X(02)00219-6
- 3. Hariri, P., Vuorinen, M. and Zhang, X.H. (2017) Inequali-ties and Bi-Lipschitz Conditions for Triangular Ratio Metric. The Rocky Mountain Journal of Mathematics, 47, 1211-1148. https://doi.org/10.1216/RMJ-2017-47-4-1121
- 4. Rainio, O. and Vuorinen, M. (2023) Triangular Ratio Metric under Quasiconformal Mappings in Sector Domains. Computational Methods and Function Theory, 23, 269-293. https://doi.org/10.1007/s40315-022-00447-3
- 5. Chen, J.L., Hariri, P., Klén, R., et al. (2015) Lipschitz Conditions, Triangular Ratio Metric, and Quasiconformal Maps. Annales Academiae Scientiarum Fennicae Mathematica, 40, 683-709. https://doi.org/10.5186/aasfm.2015.4039
- 6. Jia, G.L. and Zhang, X.H. (2020) Bounds and Inequalities for the Triangular Ratio Metric. Journal of Zhejiang Sci-Tech University, 43, 858-864.
- 7. Ibragimov, Z. (2009) The Cassinian Metric of a Domain in . Uzbek Mathematical Journal, 1, 53-67.
- 8. Ibragimov, Z., Mohapatra, M.R., Sahoo, S.K., et al. (2017) Geometry of the Cassinian Metric and Its Inner Metric. Bulletin of the Malaysian Mathematical Sciences Society, 40, 361-372. https://doi.org/10.1007/s40840-015-0246-6
- 9. Wu, Y.P. and Wang, G.D. (2020) Some Properties of the Cassinian Metric. Journal of Zhejiang Sci-Tech University, 43, 865-870.
- 10. Beardon, A.F. (1983) The Geometry of Discrete Groups. Springer, New York. https://doi.org/10.1007/978-1-4612-1146-4
- 11. Gehring, F.W. and Osgood, B.G. (1979) Uniform Domains and the Quasi-Hyperbolic Metric. Journal d’Analysen Mathématique, 36, 50-74. https://doi.org/10.1007/BF02798768
- 12. Vuorinen, M. (2006) Conformal Geometry and Quasiregular Mappings. Springer, Berlin.
- 13. Hariri, P., Klén, R., Vuorinen, M., et al. (2017) Some Remarks on the Cassinian Metric. Publicationes Mathematicae-Debrecen, 90, 269-285. https://doi.org/10.5486/PMD.2017.7386