Pure Mathematics
Vol.04 No.06(2014), Article ID:14392,6
pages
10.12677/PM.2014.46038
Asymptotic Properties for the Parameter Estimator in the Near-Explosive Autoregressive Process
Mingming Yu, Jiao Meng
Nanjing University of Aeronautics and Astronautics, Nanjing
Email: mengyilianmeng@163.com, zbmengjiao@sina.com
Received: Oct. 13th, 2014; revised: Nov. 11th, 2014; accepted: Nov. 20th, 2014
Copyright © 2014 by authors and Hans Publishers Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



ABSTRACT
In this paper, we focus our attention on the following near-explosive autoregressive process: . When
. When  and
and  in the near-explosive case, the asymptotic distributions for the least squares estimator of
in the near-explosive case, the asymptotic distributions for the least squares estimator of 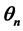 can be obtained.
can be obtained.
Keywords:Autoregressive Process, Least Squares Estimator, Near-Explosive

近爆炸性自回归序列中参数估计量的渐近性质
于明明,孟娇
南京航空航天大学,南京
Email: mengyilianmeng@163.com, zbmengjiao@sina.com
收稿日期:2014年10月13日;修回日期:2014年11月11日;录用日期:2014年11月20日

摘 要
本论文的目的是研究近爆炸性自回归序列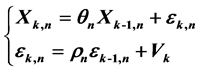 中, 当
中, 当 ,
, 时参数
时参数 最小二乘估计量的渐近分布。
最小二乘估计量的渐近分布。
关键词 :自回归序列,最小二乘法估计量,近爆炸

1. 介绍
本文我们讨论下面自回归序列:
 . (1)
. (1)
并且满足下列假设:
(1) ,
, 是未知参数,并且满足
是未知参数,并且满足 ,
, ,
,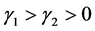 ,其中
,其中 且
且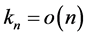 ;
;
(2) 是独立同分布
是独立同分布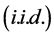 随机变量,且
随机变量,且 ,
, ;
;
(3)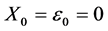 。
。
为了估计未知参数 ,我们通过使
,我们通过使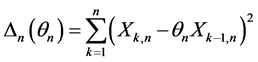 的值达到最小得到
的值达到最小得到 的最小二乘估计为
的最小二乘估计为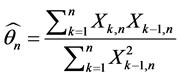 ,
, 。
。
首先,当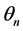 是一个固定的常数
是一个固定的常数 且
且 时,这种情况下
时,这种情况下 的渐近分布已经被很多科研者证明出来,我们可以参考文献 [1] [2] ,情况再复杂一点,当
的渐近分布已经被很多科研者证明出来,我们可以参考文献 [1] [2] ,情况再复杂一点,当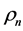 也是一个固定的常数
也是一个固定的常数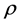 时,在 [3] 中,作者证出了
时,在 [3] 中,作者证出了 的渐近正态性。其次,科研者们不再满足于研究
的渐近正态性。其次,科研者们不再满足于研究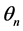 固定时的情况,假设
固定时的情况,假设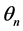 是一个可变的序列,
是一个可变的序列,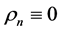 ,Chan
,Chan
和Wei在 [4] 中证出了在 时,
时, 渐近于一个布朗运动,当
渐近于一个布朗运动,当 ,在 [5] 中,作者给出
,在 [5] 中,作者给出
了 的渐近分布。
的渐近分布。
最后,在这篇文章中我们来考虑 ,
,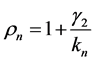 ,
, 时
时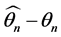 的渐近性质。我们有如下主要的结论:
的渐近性质。我们有如下主要的结论:
定理1:当 充分大时
充分大时
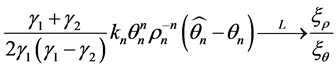 ,
,
这里 表示依分布收敛,
表示依分布收敛, ,其中
,其中 。
。
2. 定理的证明
下面为了计算方便,对所有的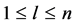 ,我们令
,我们令
 ,
, ,
,
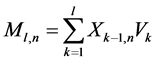 ,
,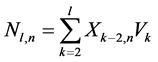 ,
,
计 ,
,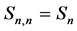 ,
, ,
, ,这样我们得到
,这样我们得到
 。
。
另外,定义一些新的序列
 ,
, ,
,
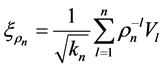 ,
,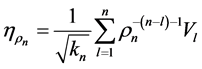 。
。
为了证明定理内容,我们引入下面引理
引理2.1:对于模型(1),我们得到
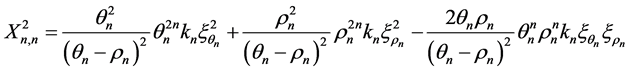
以及 ,
, 。
。
证明:由Phillips和Magdalinos [6] ,我们知 ,故对所有的
,故对所有的 ,有
,有
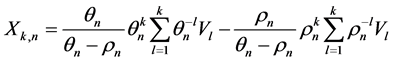 , (2)
, (2)
通过简单的计算,我们可以得到

另外,通过公式(2)和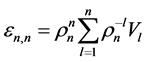 ,我们可以得到
,我们可以得到
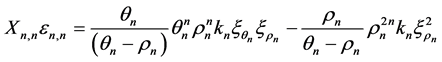 以及
以及 。
。
引理2.2:当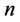 无穷大时,我们得到
无穷大时,我们得到
 ,其中
,其中 ,
,
并且

证明:通过 [7] 中的推论5.5,我们只需要证明:对任意的非零向量 ,有
,有
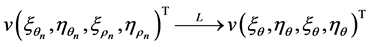 ,对任意的
,对任意的 ,令
,令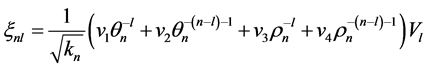 ,有
,有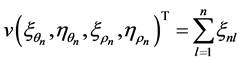 。
。
一方面,因为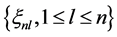 是一个独立非同分布随机变量列,通过一些简单的计算可得到
是一个独立非同分布随机变量列,通过一些简单的计算可得到

其中

另一方面,因为当 无穷大时,存在一个足够大的数
无穷大时,存在一个足够大的数 ,有
,有

并且
对任意的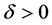 ,我们有
,我们有

因为 ,
,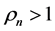 故有
故有 ,所以我们可以得到
,所以我们可以得到
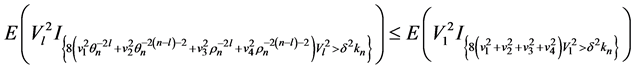
即有 ,又因为
,又因为 的可积性及
的可积性及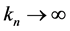 ,所以有
,所以有
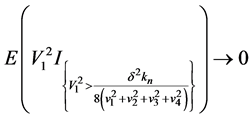 ,因此我们可以得到Lindeberg条件
,因此我们可以得到Lindeberg条件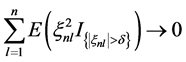 ;
;
基于上面两方面的原因,我们可以得到这个引理的证明。
引理2.3:当 无穷大时,我们可以得到
无穷大时,我们可以得到
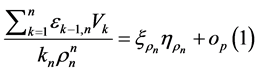 ,
, ,
, ,以及
,以及
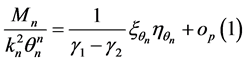 ,
,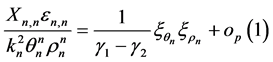 。
。
证明:首先,我们通过Phillip和Magdalions在 [6] 中对公式(10)的证明以及引理2.1可以得到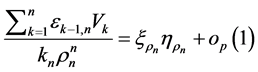 以及
以及 。
。
其次,通过引理2.1,引理2.2以及 ,我们可以得到
,我们可以得到

另外

易知:
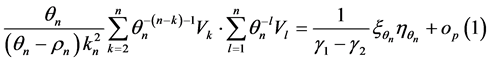 (3)
(3)
 (4)
(4)
又因为
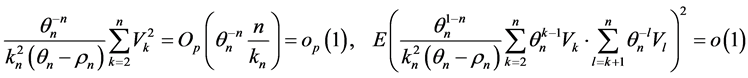
故
 (5)
(5)
同理可知:
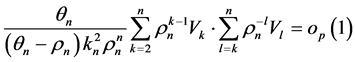 (6)
(6)
结合(3) (4) (5) (6)得:
 。
。
最后,易知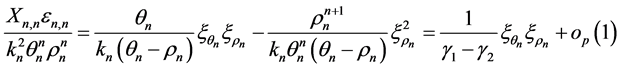 。
。
引理2.4:当 无穷大时,我们有
无穷大时,我们有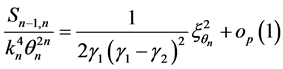 。
。
证明:由[3] 中(A.14)和(A.23)式,令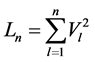 ,得到
,得到
 ; (7)
; (7)
又
 , (8)
, (8)
并且
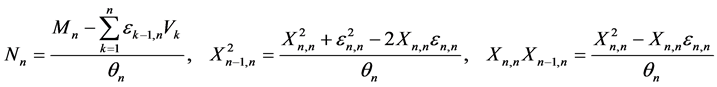 ,
,
因此根据(7)式我们可以得到 ,其中
,其中

由引理2.3得 及
及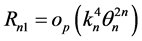 ,引理得证。
,引理得证。
定理1的证明
由(7) (8)式得,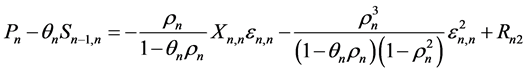 ,
,
其中 。
。
由引理2.1和引理2.3得
 (9)
(9)
又经过简单的计算得
 , (10)
, (10)
结合(9)(10),定理得证。
致谢
本论文是在我与同学孟娇的合作中完成的。感谢导师给予我们的支持,感谢南京航空航天大学数学系的各位老师给予我们的指导和帮助,感谢各位文献作者的成果给予我们的借鉴。
文章引用
于明明,孟 娇, (2014) 近爆炸性自回归序列中参数估计量的渐近性质
Asymptotic Properties for the Parameter Estimator in the Near-Explosive Autoregressive Process. 理论数学,06,261-267. doi: 10.12677/PM.2014.46038
参考文献 (References)
- 1. Anderson, T.W. (1959) On asymptotic distributions of estimators of parameters of stochastic difference equations. Annals of Mathematical Statistics, 32, 676-687.
- 2. Zhou, Z.Z. and Lin, Z.Y. (1958) Asymptotic theory for LAD es-timation of moderate deviations from a unit root. Statistics and Probability Letters, 29, 1188-1197.
- 3. Bercu, B. and Proia, F. (2013) A sharp analysis on the asymptotic behavior of the Durbin-Watson statistic for the first-order autore-gressive process. ESAIM: Probability and Statistics, 17, 500-530.
- 4. Chan, N.H. and Wei, C.Z. (1987) Asymptotic inference for nearly nonstationary AR(1) processes. Annals of Statistics, 15, 1050-1063.
- 5. Nabeya, S. and Perron, P. (1994) Local asymptotic distributions related to the AR(1) model with dependent errors. Journal of Econometrics, 62, 229-264.
- 6. Phillips, P.C.B. and Magdalinos, T. (2007) Limit theory for moderate deviations from a unit root. Journal of Econometrics, 136, 115-130.
- 7. Kallenberg, O. (2002) Foundations of modern probability. Springer, Berlin.