Pure Mathematics
Vol.06 No.03(2016), Article ID:17522,6
pages
10.12677/PM.2016.63025
Induced Maps Preserving Multiplicative Matrices over Fields
Jun Zhang, Chongguang Cao
Heilongjiang University, Harbin Heilongjiang

Received: Apr. 25th, 2016; accepted: May 9th, 2016; published: May 12th, 2016
Copyright © 2016 by authors and Hans Publishers Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



ABSTRACT
Let F be a field,  be the set of all
be the set of all 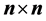 matrices over F. If a map
matrices over F. If a map 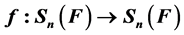 is defined by
is defined by
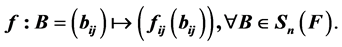
where  is the set of functions on F, then f is called a map induced by
is the set of functions on F, then f is called a map induced by  on
on . If
. If  implies
implies , then f is called preserving multiplicative matrices. In this paper, we characterize induced maps preserving multiplicative matrices over fields.
, then f is called preserving multiplicative matrices. In this paper, we characterize induced maps preserving multiplicative matrices over fields.
Keywords:Field, Preserving Multiplicative, Induced Map

域上矩阵保乘积的诱导映射
张隽,曹重光
黑龙江大学,黑龙江 哈尔滨

收稿日期:2016年4月25日;录用日期:2016年5月9日;发布日期:2016年5月12日

摘 要
令F是一个域, 是F上所有
是F上所有 对称矩阵的集合。如果一个映射
对称矩阵的集合。如果一个映射 被定义如下,
被定义如下,
 。
。
其中, 是关于F的函数集,则称f是
是关于F的函数集,则称f是 的由
的由 诱导的映射。如果对于
诱导的映射。如果对于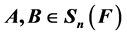 有
有 ,则f被称为保矩阵乘积。本文我们刻画域上矩阵保乘积的诱导映射。
,则f被称为保矩阵乘积。本文我们刻画域上矩阵保乘积的诱导映射。
关键词 :域,保乘积,诱导映射

1. 引言
刻画矩阵集合保持某些性质的映射称为矩阵保持问题研究。近年来,这种研究更感兴趣于映射没有线性和加法假定的情形,例如 [1] - [5] 。本文研究的诱导映射,其实也是这类问题的一种,又如 [6] 及 [7] 。
设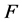 是一个域,
是一个域, 记
记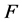 上所有
上所有 阶对称矩阵的集合。设
阶对称矩阵的集合。设 是
是 到自身的映射,
到自身的映射,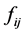 是
是 上的函数,其中
上的函数,其中 。如果定义
。如果定义
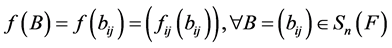
则称 是由
是由 诱导的映射。简称
诱导的映射。简称 的诱导映射。
的诱导映射。
在本文中令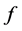 是
是 到自身的诱导映射,如果
到自身的诱导映射,如果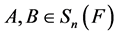 意味着
意味着 则称f保乘积。本文目的是刻画
则称f保乘积。本文目的是刻画 的保乘积的诱导映射。
的保乘积的诱导映射。
在本文中记 为单位矩阵,用
为单位矩阵,用 记
记 中所有非0元的集合,
中所有非0元的集合, 表示
表示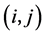 位置是1,其余位置是零的矩阵。记
位置是1,其余位置是零的矩阵。记 。
。
容易证明,若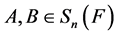 ,则
,则 当且仅当
当且仅当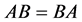 。
。
2. 主要结果
在 的情况下,对于
的情况下,对于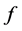 为
为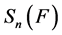 的保乘积的诱导映射的充要条件,我们有如下的重要结果。
的保乘积的诱导映射的充要条件,我们有如下的重要结果。
定理1.1. 设 为一个域,
为一个域, 为整数且
为整数且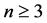 。
。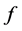 是
是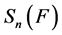 的诱导映射,则
的诱导映射,则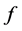 保乘积当且仅当存在一个可逆对角阵
保乘积当且仅当存在一个可逆对角阵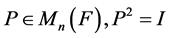 及
及 上的单自同态
上的单自同态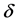 使得
使得
 (1)
(1)
其中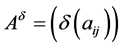 。
。
证明:充分性显然,下证必要性。假设 且互不相等,令
且互不相等,令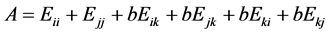 ,
,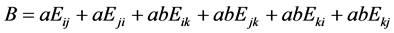
易见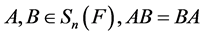 ,由
,由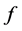 保乘积知
保乘积知 ,看
,看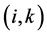 位置得
位置得
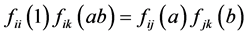 。 (2)
。 (2)
下面分两种情况讨论。
1) 对于任意不同的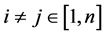 及任意
及任意 均有
均有 。由此情形条件可知
。由此情形条件可知
 , (3)
, (3)
 。 (4)
。 (4)
令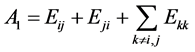 ,
, ,易见
,易见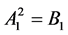 得
得 ,
,
看 位置且由(4)得
位置且由(4)得
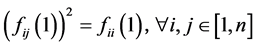 , (5)
, (5)
易推出 。由
。由 得到
得到 得
得 或1,易得
或1,易得
 , (6)
, (6)
故由(2)得
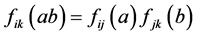 , (7)
, (7)
令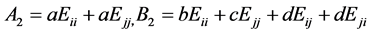 ,易见
,易见
由 保乘积知
保乘积知 ,看
,看 位置得
位置得 ,又由
,又由
可得
 (8)
(8)
由 易见
易见 看
看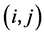
位置得
 , (9)
, (9)
令 ,由(7)可知,当
,由(7)可知,当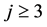 时,有
时,有
 ,
,
又对 有
有
 ,
,
因此有
 , (10)
, (10)
由 得
得 ,又由(10)得
,又由(10)得
 ,
,
满足(10)。当 时,由
时,由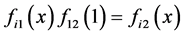 可推出
可推出
 ,
,
仍然满足(10)。总之(10)对所有情形成立。
令 ,则由(7)计算可得
,则由(7)计算可得 ,则有
,则有
 , (11)
, (11)
再由 ,易见
,易见
 看
看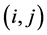 位置得
位置得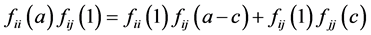 ,由
,由
和(9)可得
 , (12)
, (12)
从而有
 , (13)
, (13)
式意味着 ,故
,故 为
为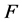 的单自同态。
的单自同态。
令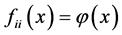 ,则
,则 中,
中,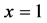 时,有
时,有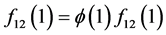 ,则
,则
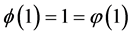 , (14)
, (14)
由(9)得 ,可推出
,可推出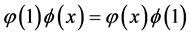 ,
,
又由(14)可得 。
。
由(4)和(10),设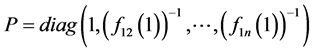 ,易见
,易见
 ,
,
注意到由(5)和(6)易得 ,显然有
,显然有 ,这样结论1)被证明。
,这样结论1)被证明。
2) 若存在某 及某
及某 使
使 ,
,
令 ,由
,由 ,看
,看
 和
和 位置得
位置得
 , (15)
, (15)
 , (16)
, (16)
令 ,
,
由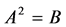 ,看
,看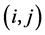 位置,得
位置,得
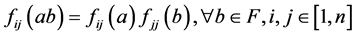 , (17)
, (17)
由(17)可得 即得
即得 ,又由(15)和(16)可得
,又由(15)和(16)可得 。由(15)可得
。由(15)可得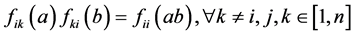 ,
,
由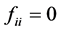 可得
可得 。由(16)可得,
。由(16)可得, ,由
,由 ,得到
,得到 。同理,
。同理,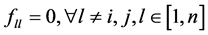 。由(17)易得
。由(17)易得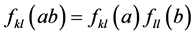 ,由
,由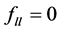 ,可得
,可得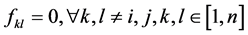 因此,推出
因此,推出 ,证毕。
,证毕。
定理1.2. 令F为一个域,设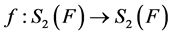 为一个由函数
为一个由函数 导出的映射,满足
导出的映射,满足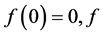 保乘积当且仅当存在一个可逆对角阵
保乘积当且仅当存在一个可逆对角阵 的且
的且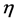 为
为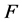 上的单自同态,那么有
上的单自同态,那么有
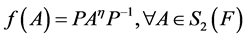 。 (18)
。 (18)
证明:下面分两种情况讨论。
1) 对于任意不同的 及任意
及任意 均有
均有 。
。
充分性显然,下证必要性。令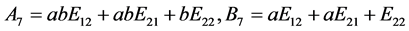 ,易得
,易得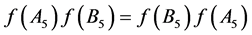 ,看(1,2)位置且由(6)得到
,看(1,2)位置且由(6)得到
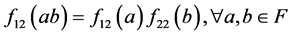 , (19)
, (19)
由(4)~(6)可得
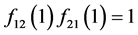 , (20)
, (20)
由(8),令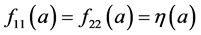 ,在(21)中,令
,在(21)中,令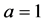 ,得到
,得到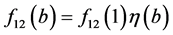 ,
,
又由(20)得到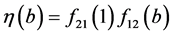 ,故
,故
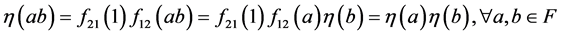 , (21)
, (21)
又由(12)可得
 , (22)
, (22)
又由(4),因此得到
 ,令
,令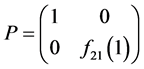 ,
,
显然有 且
且
 。 (23)
。 (23)
2) 对于某 有
有 。
。
令 由
由 看(1,1)位置得
看(1,1)位置得
 , (24)
, (24)
看其(2,2)位置得
 , (25)
, (25)
由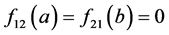 可得
可得 即
即
 , (26)
, (26)
令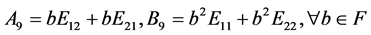 ,由
,由 ,看(1,1)位置,又由(4)得
,看(1,1)位置,又由(4)得
 , (27)
, (27)
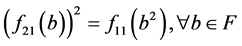 , (28)
, (28)
又由(26)得
 , (29)
, (29)
综上所述,由(26)和(29)得到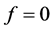 。
。
文章引用
张 隽,曹重光. 域上矩阵保乘积的诱导映射
Induced Maps Preserving Multiplicative Matrices over Fields[J]. 理论数学, 2016, 06(03): 166-171. http://dx.doi.org/10.12677/PM.2016.63025
参考文献 (References)
- 1. Li, C.K., Plevnik, L. and Semrl, P. (2012) Preservers of Matrix Pairs with a Fixed Inner Product Value. Operators and Matrices, 6, 433-464.
- 2. Cao, C.G., Ge, Y.L. and Yao, H.M. (2013) Maps Preserving Classical Adjoint of Products of Two Matrices. Linear and Multilinear Algebra, 61, 1593-1604. http://dx.doi.org/10.1080/03081087.2012.753592
- 3. Huang, L.P. (2006) Geometry of Matrices over Ring. Science Press.
- 4. You, H. and Wang, Z.Y. (2007) k-Potence Preserving Maps without the Linearty and Surjectivity Assumptions. Linear Algebra and Its Applications, 426, 238-254. http://dx.doi.org/10.1016/j.laa.2007.04.024
- 5. Chooi, W.L. and Ng, W.S. (2010) On Classical Ad-joint-Commuting Mappings between Matrix Algebras. Linear Algebra and Its Applications, 432, 2589-2599. http://dx.doi.org/10.1016/j.laa.2009.12.001
- 6. Liu, S.W. and Zhang, G.D. (2006) Maps Preserving Rank $1$ Matrices over Fields. Journal of Natural Science of Heilongjiang University, 23, 138-140.
- 7. Yang, L., Ben, X.Z., Zhang, M. and Cao, C.G. (2014) Induced Maps on Matrices over Fields. Abstract and Applied Analysis, 2014, Article ID: 596796.