Advances in Applied Mathematics
Vol.06 No.03(2017), Article ID:20782,10
pages
10.12677/AAM.2017.63037
Exact Multiplicity of Positive Solutions for Convex-Concave-Convex Nonlinearities
Zirao Huang, Dingyong Bai
School of Mathematics and Information Science, Guangzhou University, Guangzhou Guangdong

Received: May 7th, 2017; accepted: May 22nd, 2017; published: May 27th, 2017

ABSTRACT
In this paper we study the bifurcation curve of the Dirichlet boundary value problem with convex- concave-convex nonlinearities. By using Time-map techniques, we prove that the bifurcation curve of the boundary value problem is S-shaped when the nonlinearities are asymptotic sublinear. Consequently, the exact multiplicity of positive solutions is determined.
Keywords:Exact Multiplicity of Positive Solutions, Dirichlet Boundary Value Problem, Bifurcation Curve, Time-Map
具有凸-凹-凸非线性项的边值问题正解的确切个数
黄子饶,白定勇
广州大学数学与信息科学学院,广东 广州
收稿日期:2017年5月7日;录用日期:2017年5月22日;发布日期:2017年5月27日

摘 要
本文研究了具有凸-凹-凸非线性项的狄利克雷边值问题正解的分支曲线。通过时间映射分析法,证明了在非线性项为渐近次线性时,边值问题的正解分支曲线为S-型曲线,从而确定了边值问题的正解的确切个数。
关键词 :确切正解个数,狄利克雷边值问题,分支曲线,时间映射

Copyright © 2017 by authors and Hans Publishers Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/


1. 引言
本文考虑以下边值问题:
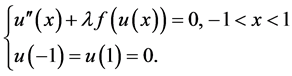 (1.1)
(1.1)
其中 为分支参数,
为分支参数,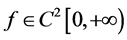 。
。
边值问题正解的分支的研究一直是微分方程领域的重要部分。关于边值问题正解研究,目前比较常用的方法有上下解方法、积分法、分支理论、时间映射分析法等。对于非线性项为凹函数、凸函数、凹-凸函数以及凸-凹函数的研究已有大量的成果。在1970年,T. Laetsch [1] 就利用积分法研究了边值问题
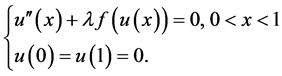 (1.2)
(1.2)
在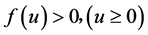 ,
,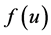 在
在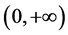 为凸函数时,得出了(1.2)的正解的确切个数。2001年,S.-H. Wang和D.-M. Long [2] 利用时间映射分析法研究了边值问题
为凸函数时,得出了(1.2)的正解的确切个数。2001年,S.-H. Wang和D.-M. Long [2] 利用时间映射分析法研究了边值问题
 (1.3)
(1.3)
在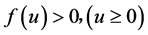 ,
,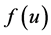 在
在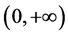 为凹-凸函数且满足一定条件时,得出了(1.3)的正解的确切个数。2011年,K.-C. Hung和S.-H. Wang [3] 利用时间映射分析法证明了当
为凹-凸函数且满足一定条件时,得出了(1.3)的正解的确切个数。2011年,K.-C. Hung和S.-H. Wang [3] 利用时间映射分析法证明了当 ,
, 在
在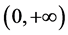 为凸-凹函数且为渐近次线性时,(1.1)正解的分支曲线在
为凸-凹函数且为渐近次线性时,(1.1)正解的分支曲线在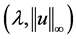 -平面上为S-型曲线。2014年,P. Korman和Y. Li [4] 利用分支理论证明了当
-平面上为S-型曲线。2014年,P. Korman和Y. Li [4] 利用分支理论证明了当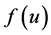 分别在
分别在 为凸-凹函数或凹-凸函数且满足一定条件时,(1.1)正解的分支曲线在
为凸-凹函数或凹-凸函数且满足一定条件时,(1.1)正解的分支曲线在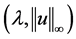 -平面上为S-型曲线或反S-型曲线。
-平面上为S-型曲线或反S-型曲线。
当(1.1)的非线性项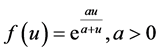 时,即为扰动的Gelfand问题。对于此问题,也有大量的成果。通过 [5] 的证明,存在一个分支值
时,即为扰动的Gelfand问题。对于此问题,也有大量的成果。通过 [5] 的证明,存在一个分支值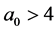 ,使得当
,使得当 ,(1.1)正解的分支曲线在
,(1.1)正解的分支曲线在 -平面上单调递增;当
-平面上单调递增;当 ,(1.1)正解的分支曲线在
,(1.1)正解的分支曲线在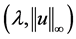 -平面上为S-型曲线。在 [3] [6] [7] [8] 中都对
-平面上为S-型曲线。在 [3] [6] [7] [8] 中都对 取近似值,最新的结果为 [8] 中的
取近似值,最新的结果为 [8] 中的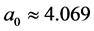 。对于此问题的研究,还可参考 [9] [10] [11] [12] [13] 。
。对于此问题的研究,还可参考 [9] [10] [11] [12] [13] 。
不难注意到,很多文献都是对非线性项为凸函数或凹-凸函数或凸-凹函数时进行研究,而很少有对非线性项为凹-凸-凹函数或凸-凹-凸函数时的研究,这是因为在这两类情况下,时间映射的图形十分复杂,讨论其单调性及极值点将变得十分麻烦。本文对非线性项为凸-凹-凸函数进行研究,证明了当非线性项为渐近次线性时,(1.1)正解的分支曲线在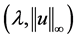 -平面上为S-型曲线。
-平面上为S-型曲线。
2. 准备工作
定义(1.1)正解的分支曲线
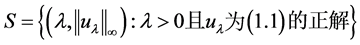 .
.
则分支曲线在 -平面上为S-型曲线即为S恰好存在2个转向点
-平面上为S-型曲线即为S恰好存在2个转向点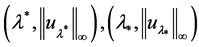 ,其中
,其中 ,使得:
,使得:
1)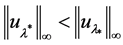 。
。
2) 分支曲线S在 向左转向。
向左转向。
3) 分支曲线S在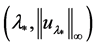 向右转向。
向右转向。
首先,设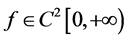 ,且有以下假设条件:
,且有以下假设条件:
(H1)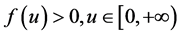 。
。
(H2)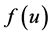 在
在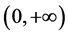 上是凸-凹-凸函数,即存在
上是凸-凹-凸函数,即存在 ,使得
,使得

(H3)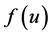 为渐近次线性,即:
为渐近次线性,即: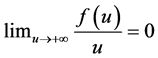 。
。
为了提出下面的假设条件,先定义以下函数:
 . (2.1)
. (2.1)
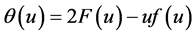 . (2.2)
. (2.2)
因此
 . (2.3)
. (2.3)
 .
.
由(H2)知, 在
在 上是凹-凸-凹函数,即
上是凹-凸-凹函数,即
 (2.4)
(2.4)
若 ≤0,则由(2.4),(H3)以及
≤0,则由(2.4),(H3)以及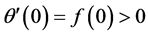 ,易得:存在
,易得:存在 ,使得:
,使得:
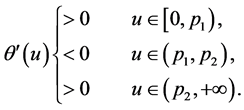 (2.5)
(2.5)
其中 均为
均为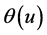 的极值点。
的极值点。
再定义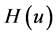 :
:
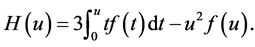 (2.6)
(2.6)
则:
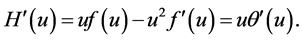 (2.7)
(2.7)
因此在 时,
时, 与
与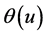 有着相同的单调性以及极值点。
有着相同的单调性以及极值点。
有了以上的定义,再提出以下的假设:
(H4)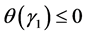 。
。
(H5)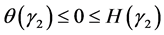 或
或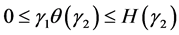 ,即
,即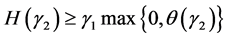 。
。
以下为本文的主要结果:
定理2.1 设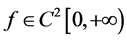 ,若
,若 满足(H1)-(H5),则(1.1)正解的分支曲线在
满足(H1)-(H5),则(1.1)正解的分支曲线在 -平面上为S-型曲线,即存在两个正数
-平面上为S-型曲线,即存在两个正数 ,当
,当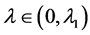 或
或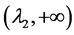 时,(1.1)存在唯一正解,当
时,(1.1)存在唯一正解,当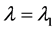 或
或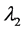 时,(1.1)恰好存在两个正解,当
时,(1.1)恰好存在两个正解,当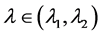 时,(1.1)恰好存在三个正解;且
时,(1.1)恰好存在三个正解;且 。
。
3. 主要结果的证明
本文使用J. Smoller和A. Wasserman [14] 以及T. Laetsch [1] 使用过的时间映射分析法对(1.1)进行分析,时间映射等式为:
 . (3.1)
. (3.1)
 为(1.1)的正解当且仅当
为(1.1)的正解当且仅当
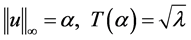 . (3.2)
. (3.2)
因此,研究(1.1)正解的个数等同于研究时间映射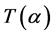 在
在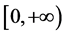 上的图像。
上的图像。
为了证明定理2.1,给出以下引理:
引理3.1 [1] 设 ,若
,若 满足(H1)-(H3),则
满足(H1)-(H3),则
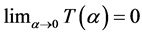 ,
,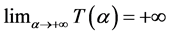 . (3.3)
. (3.3)
且 在
在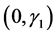 最多只有一个极值点,且为极大值点。
最多只有一个极值点,且为极大值点。
引理3.2设 ,若
,若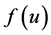 满足(H1)-(H5),则
满足(H1)-(H5),则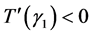 ,且
,且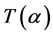 在
在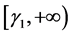 上恰好有一个极值点,且为极小值点,此极值点在
上恰好有一个极值点,且为极小值点,此极值点在 上。
上。
证明:分几步进行证明,首先证明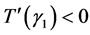 。
。
由(3.1)计算得:
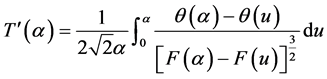 . (3.4)
. (3.4)
由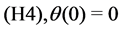 及(2.5)可知:对任意的
及(2.5)可知:对任意的 ,有
,有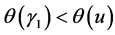 ,且
,且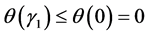 ,所以
,所以
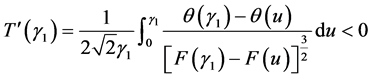 . (3.5)
. (3.5)
接下来证明 在
在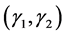 上存在唯一的极值点,且为极小值点。把区间
上存在唯一的极值点,且为极小值点。把区间 分为
分为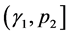 以及
以及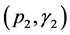 ,分几步进行证明:
,分几步进行证明:
1) 由上面证明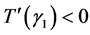 的过程以及(2.5),易得:对任意的
的过程以及(2.5),易得:对任意的 ,有
,有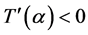 。因此
。因此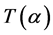 在
在 上单调递减,从而不存在任何极值点。
上单调递减,从而不存在任何极值点。
2) 接下来证明 。
。
a) 若 ,因为
,因为 ,所以由(2.5)得:存在
,所以由(2.5)得:存在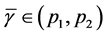 ,使得:
,使得:

以及
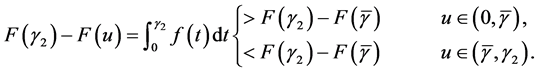
因此
 . (3.6)
. (3.6)
不难注意到等号不能取,所以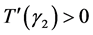 。
。
b) 若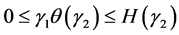 ,则存在
,则存在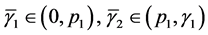 ,使得:
,使得:
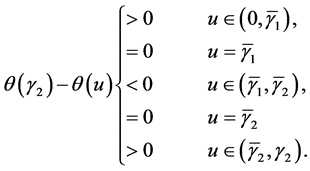
以及
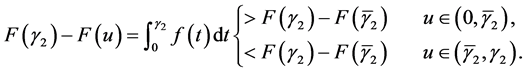
因此
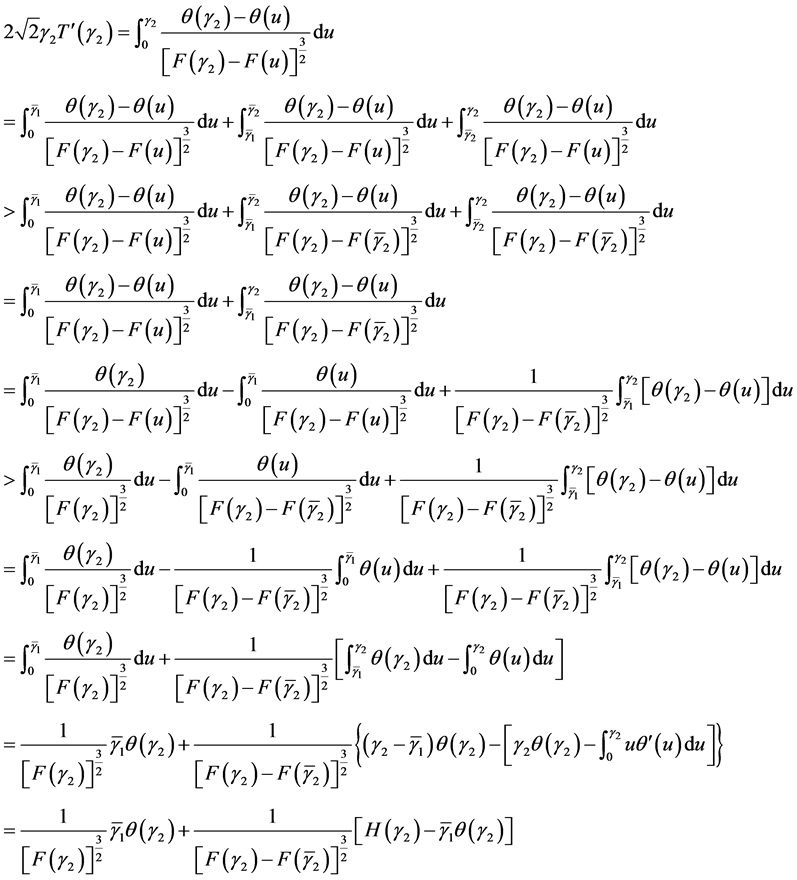 .
.
因为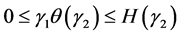 ,所以
,所以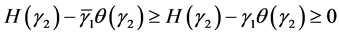 。因此
。因此
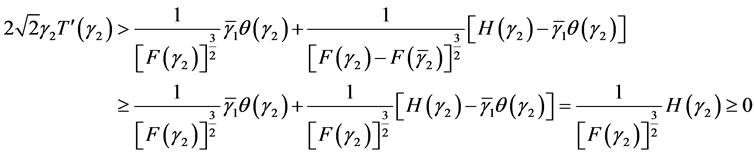 . (3.7)
. (3.7)
不难注意到等号不能取,所以 。
。
因此,无论是 还是
还是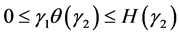 ,都有
,都有 。
。
3) 下面证明在 内,
内,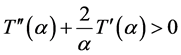 。
。
由(3.4)可计算得:
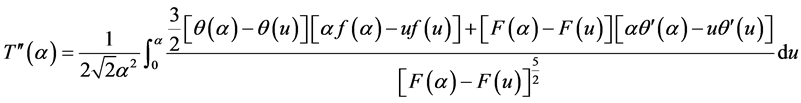 (3.8)
(3.8)
所以
 . (3.9)
. (3.9)
其中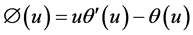 ,所以
,所以 ,且:
,且:
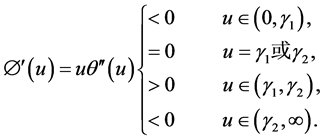 (3.10)
(3.10)
下面分两种情况讨论:
a) 若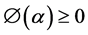 ,则对任意的
,则对任意的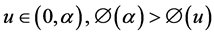 且
且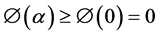 ,所以易得
,所以易得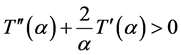 。
。
b) 若 ,因为
,因为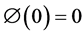 ,所以由(3.10)得:存在
,所以由(3.10)得:存在 ,使得:
,使得:
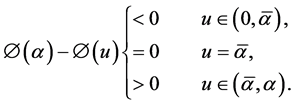
以及

所以
 . (3.11)
. (3.11)
注意到 ,且
,且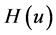 与
与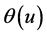 有相同的单调性及极值点
有相同的单调性及极值点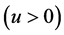 ,所以由(2.5)及(3.10),可得:
,所以由(2.5)及(3.10),可得:
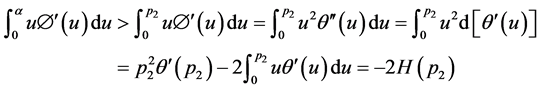 .
.
下面简单地证明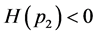 。在
。在 ,有
,有 ,
, ,所以
,所以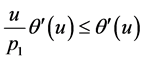 ;而在
;而在 ,有
,有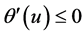 ,
,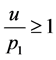 ,所以
,所以 。因此在
。因此在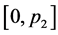 上,都有
上,都有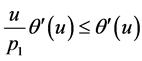 ,即
,即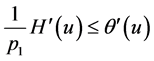 ,对其从0到p2积分,可得:
,对其从0到p2积分,可得: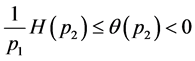 。所以
。所以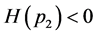 。
。
因此由(3.11)得:对所有在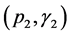 上且满足
上且满足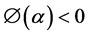 的
的 ,都有:
,都有:
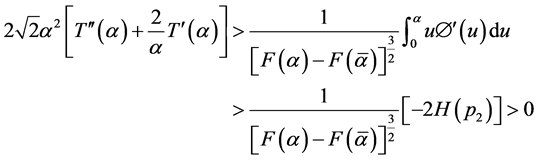 . (3.12)
. (3.12)
所以对所有的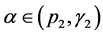 ,都有
,都有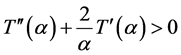 。
。
由前面的证明可知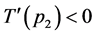 ,
,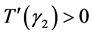 ,所以
,所以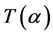 在
在 上至少有一个极值点,且为极小值点。又因为对所有的
上至少有一个极值点,且为极小值点。又因为对所有的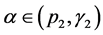 ,都有
,都有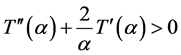 。设
。设 为
为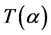 在
在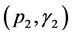 内的任意一个极值点,则由(3.12),当
内的任意一个极值点,则由(3.12),当 时必有
时必有 ,因此
,因此 必为极小值,从而
必为极小值,从而 是唯一的。所以
是唯一的。所以 在
在 上存在唯一的极值点,且为极小值点。
上存在唯一的极值点,且为极小值点。
最后证明 在
在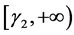 上不存在任何极值点。
上不存在任何极值点。
对任意的 ,由(2.5)易得
,由(2.5)易得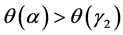 ,因此
,因此
 .
.
按照证明(3.7)的过程,同样可得 (只是被积函数的分母不同,对证明结果没有影响),就不再加以证明。所以对任意的
(只是被积函数的分母不同,对证明结果没有影响),就不再加以证明。所以对任意的 ,都有
,都有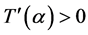 。因此
。因此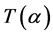 在
在 上单调递增,从而不存在任何极值点。
上单调递增,从而不存在任何极值点。
定理2.1的证明 由(2.5)知: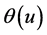 在
在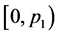 单调递增,又由前面的讨论,易得:对任意的
单调递增,又由前面的讨论,易得:对任意的 ,
,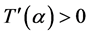 ,因此
,因此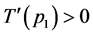 ,又
,又 ,所以
,所以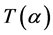 在
在 上至少有一个极值点,且为极大值点。又由引理3.1知,
上至少有一个极值点,且为极大值点。又由引理3.1知,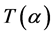 在
在 上最多只有一个极值点,且为极大值点。因此
上最多只有一个极值点,且为极大值点。因此 在
在 上存在唯一的极值点,且为极大值点,此极值点在
上存在唯一的极值点,且为极大值点,此极值点在 上。
上。
因此,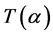 在
在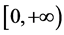 一共有两个极值点,一个在
一共有两个极值点,一个在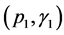 中,为极大值点;另一个在
中,为极大值点;另一个在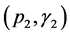 中,为极小值点;在其他区间没有极值点。设这两个极值点分别为
中,为极小值点;在其他区间没有极值点。设这两个极值点分别为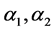 ,且
,且 ,则
,则 为极大值点,
为极大值点,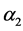 为极小值点,因此
为极小值点,因此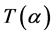 在
在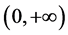 上的单调性为:
上的单调性为:
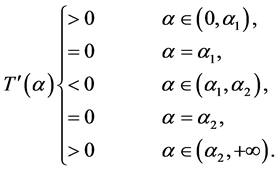 (3.14)
(3.14)
又由(3.3)有:
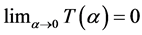 ,
,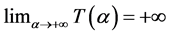 .
.
因此(1.1)正解的分支曲线在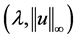 -平面上的轨迹为一条S-型曲线,它从
-平面上的轨迹为一条S-型曲线,它从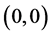 点出发向右移动,到达
点出发向右移动,到达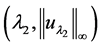 点后向左转向,到达
点后向左转向,到达 点后向右转向,此后不再发生转向且趋于无穷。即存在两个正数
点后向右转向,此后不再发生转向且趋于无穷。即存在两个正数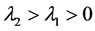 ,当
,当 或
或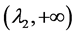 时,(1.1) 存在唯一正解,当
时,(1.1) 存在唯一正解,当 或
或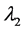 时,(1.1)恰好存在两个正解,当
时,(1.1)恰好存在两个正解,当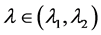 时,(1.1)恰好存在三个正解;且
时,(1.1)恰好存在三个正解;且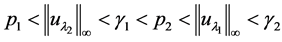 。
。
文章引用
黄子饶,白定勇. 具有凸-凹-凸非线性项的边值问题正解的确切个数
Exact Multiplicity of Positive Solutions for Convex-Concave-Convex Nonlinearities[J]. 应用数学进展, 2017, 06(03): 317-326. http://dx.doi.org/10.12677/AAM.2017.63037
参考文献 (References)
- 1. Laetsch, T. (1970) The Number of Solution of a Nonlinear Two Point Boundary Value Problem. Indiana University Mathematics Journal, 20, 1-13. https://doi.org/10.1512/iumj.1971.20.20001
- 2. Wang, S.-H. and Long, D.-M. (2001) An Exact Multiplicity Theorem Involving Concave-Convex Nonlinearities and Its Application to Stationary Solutions of a Singular Diffusion Problem. Nonlinear Analysis, 44, 469-486. https://doi.org/10.1016/S0362-546X(99)00272-2
- 3. Hung, K.-C. and Wang, S.-H. (2011) A Theorem on S-Shaped Bifurcation Curve for a Positone Problem with Convex-Concave Nonlinearity and its Applications to the Perturbed Gelfand Problem. Journal of Differential Equations, 251, 223-237. https://doi.org/10.1016/j.jde.2011.03.017
- 4. Korman, P. and Li, Y. (2014) Exact Multiplicity of Positive Solutions for Concave-Convex and Convex-Concave Nonlinearities. Journal of Differential Equations, 257, 3730-3737. https://doi.org/10.1016/j.jde.2014.07.007
- 5. Brown, K.J., Ibrahim, M.M.A. and Shivaji, R. (1981) S-Shaped Bifurcation Curves. Nonlinear Analysis, 5, 475-486. https://doi.org/10.1016/0362-546X(81)90096-1
- 6. Korman, P. and Li, Y. (1999) On the Exactness of an S-Shaped Bifurcation Curve. Proceedings of the American Mathematical Society, 127, 1011-1020. https://doi.org/10.1090/S0002-9939-99-04928-X
- 7. Huang, S.-Y. and Wang, S.-H. (2015) On S-Shaped Bifurcation Curves for a Two-Point Boundary Value Problem Arising in Theory of Thermal Explosion. Discrete and Continuous Dynamical Systems, 35, 4839-4858. https://doi.org/10.3934/dcds.2015.35.4839
- 8. Huang, S.-Y. and Wang, S.-H. (2016) Proof of a Conjecture for the One-Dimensional Perturbed Gelf and Problem from Combustion Theory. Archive for Rational Mechanics and Analysis, 222, 769-825. https://doi.org/10.1007/s00205-016-1011-1
- 9. Wang, S.-H. (1994) On S-Shaped Bifurcation Curves. Nonlinear Analysis Theory Methods & Applications, 22, 1475-1485. ttps://doi.org/10.1016/0362-546X(94)90183-X
- 10. Du, Y. (2005) Bifurcation and Related Topics in Elliptic Problems. Handbook of Differential Equations Stationary Partial Differential Equations, 2, 127-209. https://doi.org/10.1016/S1874-5733(05)80011-7
- 11. Y.Du, Y.Lou (2001) Proof of a Conjecture for the Perturbed Gelfand Equation from Combustion Theory, Journal of Differential Equations, 173, 213-230. https://doi.org/10.1006/jdeq.2000.3932
- 12. Korman, P. (2006) Global Solution Branches and Exact Multiplicity of Solutions for Two Point Boundary Value Problems. Handbook of Differential Equations Ordinary Differential Equations, 3, 547-606. https://doi.org/10.1016/S1874-5725(06)80010-6
- 13. Korman, P., Li, Y. and Ouyang, T. (2005) Computing the Location and the Direction of Bifurcation. Mathematical Research Letters, 12, 933-944. https://doi.org/10.4310/MRL.2005.v12.n6.a13
- 14. Smoller, J. and Wasserman, A. (1981) Global Bifurcation of Steady-State Solutions. Journal of Differential Equations, 39, 269-290. https://doi.org/10.1016/0022-0396(81)90077-2