Advances in Condensed Matter Physics
Vol.
13
No.
01
(
2024
), Article ID:
81578
,
8
pages
10.12677/CMP.2024.131002
一维交替在位作用(t-U-X)模型的基态相图
王李月,丁汉芹*
新疆大学物理科学与技术学院,新疆 乌鲁木齐
收稿日期:2024年1月22日;录用日期:2024年2月22日;发布日期:2024年2月29日

摘要
在弱耦合条件下,我们研究了奇(Uo)、偶(Ue)晶格上不相等Hubbard相互作用的一维非常规t-U-X模型的量子特质。在四分之一填充时,在 的情况下,不相等的在位排斥相互作用导致umklapp过程的发生。玻色化和重整化群分析有助于获得基态相图,包括一个单相超导序的金属相和分别具有自旋密度波和电荷密度波的二个绝缘相。
关键词
一维t-U-X模型,不相等Hubbard相互作用,相图,四分之一填充,弱耦合

Phase Diagram of the One-Dimensional t-U-X Model with Different Hubbard Interactions
Liyue Wang, Hanqin Ding*
School of Physical Science and Technology, Xinjiang University, Urumqi Xinjiang
Received: Jan. 22nd, 2024; accepted: Feb. 22nd, 2024; published: Feb. 29th, 2024

ABSTRACT
At weak coupling, we examine quantum properties of the one-dimensional unconventional t-U-X model with unequal Hubbard interaction at even (Ue) and odd (Uo) lattices. At quarter filling, the unequal on-site repulsive interactions have a key effect that leads to the occurrence of umklapp processes in the case of . The bosonization and renormalization-group analysis helps get the ground-state phase diagram, which includes a metallic phase with the singlet superconductivity instability and two insulating with the spin- and charge-density-wave instabilities.
Keywords:1D t-U-X Model, Different Hubbard Interaction, Phase Diagram, Quarter Filling, Weak Coupling

Copyright © 2024 by author(s) and Hans Publishers Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY 4.0).
http://creativecommons.org/licenses/by/4.0/


1. 引言
作为一种开创性的量子模型,一维(1D) Hubbard模型 [1] 已被广泛用于描述低维多体系统中的电子关联。Hubbard模型构成了我们理解高度相关材料丰富物理的基础,然而,它的标准哈密顿量仅包括与占据无关的跳跃(t)和在所有位置的等位相互作用(U)。通常,从一个位点到另一个位点的跳跃与这两个位点的电子占有数有关,这导致了密度相关的跳跃 [2] [3] [4] 的发生,它描述了位于位点和键上的电荷之间的相互作用,也称为键–电荷相互作用(X) [5] [6] [7] [8] 。U项和X项是通过关注两体势的矩阵元素的不同选择,从相同的紧束缚模型哈密顿量 [1] 中获得的。通过利用Wannier态 , , 。在位置表示中,U项和X项分别对应于势的对角矩阵元素和非对角矩阵元素。一开始,键–电荷相互作用被引导来解释混合价态材料的低能物理 [9] 。随后,X相互作用在不同的背景下得到了广泛的研究 [10] - [16] 。特别是,一维t-U-X模型 [17] [18] [19] [20] [21] 的相图研究受到了相当大的关注,其哈密顿量的形式为 [17]
(1)
通过产生( )和湮灭算符( ),可得到数密度算符 。U项描述局域电子,X项延伸到涉及最近电子的非局域轨道。此外,在一些络合物中,X的值可能更大 [22] 。系统(1)的基态性质引起了人们的极大兴趣。在半填充时,Aligia等人展示了一种非常规的绝缘体–超导体转变 [17] 。Buzatu展示了在半填充时,系统不受X项的影响,其特征是自旋密度波(SDW)相,而在非半填充时X和U相互作用相互竞争,导致基态变成具有几种类型不稳定性的金属相 [18] 。
Hubbard模型被视为相关系统的原型,并解释了许多材料的性质,而这些性质并没有用平均场理论来解释。相比之下,t-U-X模型可以描述一系列更多的现象。此外,具有格点间扩展的对角相互作用, ,也被广泛研究 [23] - [30] 。最普遍的扩展是由BKS哈密顿量给出的,它考虑了所有可能的最近邻耦合 [31] 。尽管如此,这些模型还是假设任何晶格位点都具有相同的在位相互作用。我们不得不承认,这个方案既简化了复杂的相互作用,又突出了物理性质。然而,在一些材料中确实存在不同的Hubbard相互作用,如high-Tc La2CuO4,其中Cu和O位点具有不相等的在位排斥作用 [32] 。Emery进一步将Cu-O平面转化为1D模型 [33] 。Japarized等人通过使用Cu-O链的1D哈密顿量研究了Hubbard相互作用的不等价性 [34] 。在本文中,我们扩展了模型(1),在释放等Hubbard能量假说的基础上,选取了奇数和偶数晶格位点上不同的现场相互作用,分别为Uo和Ue。考虑到这一点,该模型可以用哈密顿描述如下:
(2)
在位排斥影响umkalpp散射过程中,人们普遍认为,在1D标准情况下( ),这种散射仅发生在半填充时。 ( )的作用是有利于(阻碍)带有非零电荷的位点的跳跃 [8] 。在此,我们重点讨论四分之一填充,且所有参数均为正值( )。我们只考虑弱耦合的情况,在这种情况下,玻色化和重正化群分析方法可以有效地应用。结果表明,当Uo和Ue都大于 时,就会出现umklapp过程。这一特征在一维传统t-U-X模型(1)中是没有的。量子相图包含一个具有单线超导(SS)不稳定性的金属相和两个具有自旋密度波(SDW)和电荷密度波(CDW)不稳定性的绝缘相。
2. 玻色化和重整化群分析
费米系统的低能特性主要取决于费米表面附近的态。在一维情况下,我们只需考虑费米点附近的激发。在这种情况下,晶格场算符可以用左移( )和右移( )分量展开为 [35] ,
(3)
其中,j位点的平均电子填充度定义为 ,N电子平均占据N0个位点。一维电子系统具有电荷–自旋分离的特性。umklapp和后向过程分别影响电荷和自旋激发。相关的umklapp散射会产生能隙,从而导致绝缘态。相反,不相关的过程不能产生间隙,系统就是导体。同样,后向散射的相关性也决定了自旋间隙的存在。为了突出模型(2)不同于模型(1)的量子特性,我们分析了交替Hubbard相互作用对umklapp过程的影响。为此,我们将哈密顿方程(2)中的Uo和Ue项重写为另一种等价形式
(4)
通过表达式(3),上述均匀项和交替在位作用项被转化为手性场的组合
(5)
(6)
这里 和 。(5)和(6)中携带振荡因子的最后一项描述了我们最关心的umklapp过程。显然,只有在 或1/4时,这两个项才会有区别,这表明umklapp过程要么发生在半填充时,要么发生在四分之一填充时。在连续极限中,晶格场算符可以由连续场代替: , 。这些手性费米子算子又可以用一对对偶玻色算子 和 来表示 [35]
(7)
(8)
其中 对应于1 (−1)的值。hermitian因子 保持了不同费米子的反对易关系, 。玻色场 和 遵循 。玻色化方法 [35] [36] [37] 已被广泛应用于一维量子系统,其中的物理现象分别由自旋( )和电荷( )通道描述。
按照惯例,电荷–自旋耦合项在弱耦合机制中作为一个强无关算子可以省略 [38] [39] [40] [41] [42] 。在这一方法中,哈密顿(2)被转换为用独立电荷场和自旋场来表示:
(9)
和 项分别对应后向过程和umklapp过程。参数 和 的关系是 ,费米速度 。自旋和电荷Luttinger参数 主导着关联函数的衰变行为。g和K的振幅由相互作用和填充决定,计算公式为
(10)
(11)
(12)
(13)
除了谐振项之外,哈密顿方程(9)中还有余弦项,它们对电荷隙和自旋隙的存在与否有重要影响。这对应于已知正弦–戈登(SG)模型的重整化群(RG)分析 [35] 。根据初始g和K之间的关系,该模型的低能激发有两种不同类型的区域:
1) 对于 ,RG流被限制在强耦合区域。表征后向过程或umklapp过程的余弦项变得相关,自旋或电荷扇区出现了间隙。为了最大限度地减少基态能量,当 时, 场被固定在期望值 附近;而当 时, 场固定在0周围 [39] [40] 。
2) 对于 ,系统处于弱耦合区域。随着长度尺度的增大,余弦项被重正化为无关项。激发时没有间隙,并且 场不会被固定。
3. 基态相图
在本文中,我们只考虑四分之一填充。从方程(10)和(12)可以看出,存在 的关系。这在物理上来自自旋扇区中的SU(2)对称性,并且流沿着RG流图的分界线的方向进行。自旋间隙在 时打开,在 时关闭。因此,自旋间隙相变发生在:
(14)
在无自旋带隙的情况下,定点取 的值。
在具有较低U(1)对称性的电荷通道中,情况变得有些复杂。电荷流偏离了分界线,有了更多的选择。根据RG分析,电荷激发在以下条件下是大质量的:
(15)
同样地,它对应于不等式关系 或 。电荷场的相应期望值为 或 。无论哪种情况,都会出现umklapp散射。
与不等式(15)相反的是
(16)
描述了弱耦合区域,在该区域内,电荷激发是无质量的。重正化流最终归零,umklapp过程消失。由此可见,电荷隙过渡的边界有两个分支。对于 ,为
(17)
而在 ,为
(18)
在无电荷间隙区,除了边界(17)和(18)外,不动点的特征是 。
通过了解每个扇区中的转变,现在可以确定相位区域。首先,我们排除了键位不稳定性的存在,因为它们只出现在半填充的情况下 [41] 。因此,我们只需要考虑在位密度波和超导不稳定性,相应的序参量由玻色子场定义为:
(19)
(20)
(21)
(22)
与标准情况一样,前两个参数与奇数和偶数位置无关,描述的是SS和TS相关性。而后两者描述的是SDW和CDW相关性,依赖于位置的奇偶性:在奇数位点,它们的计算公式为:
(23)
(24)
在偶数位置,它们的形式为:
(25)
(26)
通过上述分析得出了相图(图1),该相图分为五个区域,包括三个不同的相。
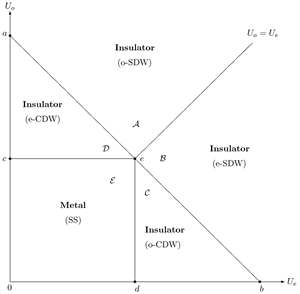
Figure 1. Phase diagram of the model (2) at quarter filling and at weak coupling
图1. 模型(2)在弱耦合情况下,考虑四分之一填充时的基态量子相图
在A和B的半间隙扇区中,电荷通道有间隙,而自旋通道没有间隙。这样就无法产生无电荷间隙的 和SS不稳定性,系统只能是绝缘体。在另一方面, 场是波动的,而 场是固定的,在A中赋予 ,在B中赋予 。很容易发现,在A区域中, 和 取非零值,而在B区域中,非零序参量为 和 。为了确定主导序参量,我们借助于相应的关联函数( ) [23] : ,而 。显然,SDW的不稳定性抑制了CDW的不稳定性。基态处于SDW相关性占主导地位的绝缘相。我们分别称它们为A区的o-SDW和B区的e-SDW。
在C和D的全间隙扇区中,自旋和电荷激发都是间隙的。这与umklapp和后向散射有关。TS、SS和SDW不稳定性都被抑制。在C区域, ,因此只有 。在D区域, ,而 ,因此只有 。该系统是一个具有o-CDW或e-CDW不稳定性的绝缘体。
在另一个半间隙扇区E中,它的激发与区域A和B中的激发相反。自旋间隙处处打开,这导致抑制了TS和SDW的不稳定性。同时,电荷间隙总是关闭的,从而导致没有umklapp过程。这与Luther-Emery (LE)后向散射模型的结构相吻合 [42] 。另一方面,自旋场的 ,而 场是波动的。因此, 和 可以同时不为零。然而,它们的相关性表现出不同的衰减行为: ,而 。由于 ,基态表现出具有以SS相关性为主导的LE金属特征。
4. 总结
我们研究了一维扩展Hubbard模型,除了额外的键荷排斥相互作用外,在位排斥作用考虑了在奇数和偶数位置不相等的情况。相比之下,本项研究可以提供对在位电子的更一般的描述。此外,所涉及的模型还与一些实际材料有关。因此,无论是理论还是实验,我们的考虑都是必要的。通过玻色化方法,将费米子哈密顿量转化为玻色子形式,通过两个独立的自旋场和电荷场可以方便地阐明基态性质。在自旋–电荷分离的假设下,该模型的红外行为可以用Sine-Gordon模型来说明,并且自旋隙和电荷隙可以独立存在。与此相关的后向和umklapp过程的出现可以通过重整化群分析来实现。结果表明,在四分之一填充的情况下,不相等的哈伯德斥力对umklapp散射有关键影响,在 的情况下会发生umklapp散射。这不仅存在电荷隙转变,还存在自旋隙转变。如上图1所示:完整的相图由五个区域组成,分别包括三个不同的相。在以Uo为纵坐标,Ue为横坐标的平面上,相图相对于 线是对称的,这从物理角度看是一个自然的结果。由于所考虑的模型满足晶格平移不变性,当交换Ue和Uo参数时,哈密顿(2)保持不变。因此,无论是 还是 ,基态结构必须是相同的。电荷–间隙相变线 和 ,交点所围成的区域被划分为金属相和绝缘相,而自旋–间隙相变线 则把绝缘项分别划分为以CDW为主导的全隙相和以SDW为主导的半隙相。这种现象不同于传统的t-U-X模型的特点。该项研究有望增加对低维多体问题的理解。
文章引用
王李月,丁汉芹. 一维交替在位作用(t-U-X)模型的基态相图
Phase Diagram of the One-Dimensional t-U-X Model with Different Hubbard Interactions[J]. 凝聚态物理学进展, 2024, 13(01): 7-14. https://doi.org/10.12677/CMP.2024.131002
参考文献
- 1. Hubbard, J. (1963) Electron Correlations in Narrow Energy Bands. Proceedings of the Royal Society of London, Series A, 276, 238-257. https://doi.org/10.1098/rspa.1963.0204
- 2. Kivelson, S., Su, W.P., Schrieffer, J.R. and Heeger, A.J. (1987) Missing Bond-Charge Repulsion in the Extended Hubbard Model: Effects in Polyacetylene. Physical Review Letters, 58, 1899-1902. https://doi.org/10.1103/PhysRevLett.58.1899
- 3. Strack, R. and Vollhardt, D. (1994) Rigorous Criteria for Ferromagnetism in Itinerant Electron Systems. Physical Review Letters, 72, 3425-3428. https://doi.org/10.1103/PhysRevLett.72.3425
- 4. Campbell, D.K., Gammel, J.T. and Loh, E.Y. (1990) Mod-eling Electron-Electron Interactions in Reduced-Dimensional Materials: Bond-Charge Coulomb Repulsion and Dimerization in Peierls-Hubbard Models. Physical Review B, 42, 475-492. https://doi.org/10.1103/PhysRevB.42.475
- 5. Painelli, A. and Girlando (1989) Electron Correlations in One Dimension: The Hubbard Model. Physical Review B, 39, 2830. https://doi.org/10.1103/PhysRevB.39.2830
- 6. Strack, R. and Vollhardt, D. (1993) Hubbard Model with Nearest-Neighbor and Bondcharge Interaction: Exact Ground-State Solution in a Wide Range of Parameters. Physical Review Letters, 70, 2637-2640. https://doi.org/10.1103/PhysRevLett.70.2637
- 7. Airoldi, M. and Parola, A. (1995) Superconducting Ground State in a Model with Bendcharge Interaction. Physical Review B, 51, 16327-16335. https://doi.org/10.1103/PhysRevB.51.16327
- 8. Luz, D.M. and Santos, R.R. (1996) Bond-Charge Interaction in the Extended Hubbard Chain. Physical Review B, 54, 1302-1307. https://doi.org/10.1103/PhysRevB.54.1302
- 9. Foglio, M.E. and Falicov, L.M. (1979) New Approach to the Theory of Intermediate Valence. I. General Formation. Physical Review B, 20, 4554. https://doi.org/10.1103/PhysRevB.20.4554
- 10. Marsiglio, F.M. and Hirsch, J.E. (1990) Hole Superconduc-tivity and the High-Tc Oxides. Physical Review B, 41, 6435-6456. https://doi.org/10.1103/PhysRevB.41.6435
- 11. FHL, Korepin, V.E. and Schoutens, K. (1993) Electronic Model for Superconductivity. Physical Review Letters, 70, 73-76. https://doi.org/10.1103/PhysRevLett.70.73
- 12. Dolcini, F. and Montorsi, A. (2002) Finite-Temperature Properties of the Hubbard Chain with Bond-Charge Interaction. Physical Review B, 66, Article ID: 075112. https://doi.org/10.1103/PhysRevB.66.075112
- 13. Anfossi, A., Giorda, P., Montorsi, A. and Traversa, F. (2005) Two-Point versus Multipartite Entanglement in Quantum Phase Transitions. Physical Review Letters, 95, Article ID: 056402. https://doi.org/10.1103/PhysRevLett.95.056402
- 14. Dobry, A.O. and Aligia, A.A. (2011) Quantum Phase Diagram of the Half Filled Hubbard Model with Bond-Charge Interaction. Nuclear Physics B, 843, 767-783. https://doi.org/10.1016/j.nuclphysb.2010.10.017
- 15. Dolcini, F. and Montorsi, A. (2013) Quantum Phases of One-Dimensional Hubbard Models with Three- and Four-Body Couplings. Physical Review B, 88, Article ID: 115115. https://doi.org/10.1103/PhysRevB.88.115115
- 16. Chen, W.T., Zhang, J. and Ding, H.Q. (2023) Ground-State Instabilities in a Hubbardtype Chain with Particular Correlated Hopping at Non-Half Filling. Results in Physics, 49, Article ID: 106472. https://doi.org/10.1016/j.rinp.2023.106472
- 17. Aligia, A.A., Anfossi, A., Arrachea, I., Boschi, C.D.L., Dobry, A.O. and Gazza, C. (2007) Incommmensurability and Unconventional Superconductor to Insulator Transition in the Hubbard Model with Bond-Charge Interaction. Physical Review Letters, 99, Article ID: 206401. https://doi.org/10.1103/PhysRevLett.99.206401
- 18. Buzatu, F.D. (1994) Phase Diagram (T, U, X) Model: Ground-State Phase Diagram in a Mean-Field-Type Approximation. Physical Review B, 49, 10176. https://doi.org/10.1103/PhysRevB.49.10176
- 19. Schadschneider, A. (1995) Superconductivity in an Exactly Solvable Hubbard Model with Bond-Charge Interaction. Physical Review B, 51, 10386. https://doi.org/10.1103/PhysRevB.51.10386
- 20. Bu Lka, B.R. (1998) Superconductivity in the Hubbard Model with Correlated Hopping: Slave-Boson Study. Physical Review B, 57, 10303-10306. https://doi.org/10.1103/PhysRevB.57.10303
- 21. Anfossi, A., Boschi, C.D.E., Montorsi, A. and Ortolani, F. (2006) Single-Site Entanglement at the Superconductor-Insulator Transition in the Hirsch Model. Physical Review B, 73, Article ID: 085113. https://doi.org/10.1103/PhysRevB.73.085113
- 22. Hubsch, A., Lin, J.C., Pan, J. and Cox, D.L. (2006) Cor-related Hybridization in Transition-Metal Complexes. Physical Review Letters, 96, Article ID: 196401. https://doi.org/10.1103/PhysRevLett.96.196401
- 23. Voit, J. (1992) Phase Diagram and Correlation Functons of the Half-Filled Hubbard Model in One Dimension. Physical Review B, 45, 4027-4042. https://doi.org/10.1103/PhysRevB.45.4027
- 24. Nakamura, M. (2000) Tricritical Behavior in the Extended Hubbard Chains. Physical Review B, 61, 16377-16392. https://doi.org/10.1103/PhysRevB.61.16377
- 25. Jeckelmann, E. (2002) Ground State Phases of the Half-Filled One-Dimensional Extended Hubbard Model. Physical Review Letters, 89, Article ID: 236401. https://doi.org/10.1103/PhysRevLett.89.236401
- 26. Tsuchiizu, M. and Furusaki, A. (2002) Phase Diagram of the One-Dimensional Extended Hubbard Model at Half Fiiling. Physical Review Letters, 88, Article ID: 056402. https://doi.org/10.1103/PhysRevLett.88.056402
- 27. Sandvik, A.W., Balents, L. and Campbell, D.K. (2004) Ground State Phases of the Half-Filled One-Dimensional Extended Hubbard Model. Physical Review Letters, 92, Article ID: 236401. https://doi.org/10.1103/PhysRevLett.92.236401
- 28. Tam, K.M., Tsai, S.W. and Campbell, D.K. (2006) Functional Renormalization Group Analysis of the Half-Filled One-Dimensional Extended Hubbard Model. Physical Review Letters, 96, Article ID: 036408. https://doi.org/10.1103/PhysRevLett.96.036408
- 29. Ejima, S. and Nishimoto, S. (2007) Phase Diagram of the One-Dimensional Half-Filled Extended Hubbard Model. Physical Review Letters, 99, Article ID: 216403. https://doi.org/10.1103/PhysRevLett.99.216403
- 30. Menard, M. and Bourbonnais, C. (2011) Renormalization Group Analysis of the Onedimensional Extended Hubbard Model. Physical Review B, 83, Article ID: 075211. https://doi.org/10.1103/PhysRevB.83.075111
- 31. De Boer, J., Korepin, V.E. and Schdaschneider, A. (1995) η Pairing as a Mechanism of Superconductivity in Models of Strongly Correlated Electrons. Physical Review Letters, 74, 789-792. https://doi.org/10.1103/PhysRevLett.74.789
- 32. Emery, VJ. (1987) Theory of High-Tc Supercon-ductivity in Oxides. Physical Review Letters, 58, 2794-2797. https://doi.org/10.1103/PhysRevLett.58.2794
- 33. Emery, V.J. (1990) Strong-Coupling Field Theory and Sol-iton Doping in a One-Dimensional Copper-Oxide Model. Physical Review Letters, 65, 1076-1079. https://doi.org/10.1103/PhysRevLett.65.1076
- 34. Japaridze, G., Khomskili, D. and Muller-Hartmann, E. (1993) The One-Dimensional 1/4-Filled Hubbard Model with Alternating Interactions. Annalen der Physik, 2, 38-50. https://doi.org/10.1002/andp.19935050105
- 35. Gogolin, A.O., Nersesyan, A.A. and Tsvelik, A.M. (1998) Bosonization and Strongly Correlated Systems. Cambridge University Press, New York.
- 36. Emery, V.J. (1979) Highly Conducting One-Dimensional Solids. Plenum, New York.
- 37. Solyom, J. (1979) The Fermi Gas Model of One-Dimensional Conductors. Advances in Physics, 28, 201-303. https://doi.org/10.1080/00018737900101375
- 38. Montorsi, A., Dolcini, F., Iotti, R.C. and Rossi, F. (2017) Symmetry-Protected Topological Phases of One-Dimensional Interacting Fermions with Spin-Charge Separation. Physical Review B, 95, Article ID: 245108. https://doi.org/10.1103/PhysRevB.95.245108
- 39. Giamarch, T. (2003) Quantum Physics in One Dimension. Oxford University Press, Oxford. https://doi.org/10.1093/acprof:oso/9780198525004.001.0001
- 40. Tsvelik, A.M. (1995) Quantum Field Theory in Condensed Matter Physics. Cambridge University Press, New York.
- 41. Japaridze, G.I. and Mul-ler-Hartmann, E. (1997) Bond-Located Ordering in the One-Dimensional Penson-Kolb-Hubbard Model. Journal of Physics C, 9, 10509-10519. https://doi.org/10.1088/0953-8984/9/47/018
- 42. Luther, A. and Emery, V.J. (1974) Backward Scattering in the One-Dimensional Electron Gas. Physical Review Letters, 33, 589-592. https://doi.org/10.1103/PhysRevLett.33.589