Pure Mathematics
Vol.4 No.06(2014), Article ID:14364,7 pages
DOI:10.12677/PM.2014.46034
The C1 Solution of Perturbation Feigenbaum Functional Equation on High-Dimensional Space
School of Mathematics and Computational Science, Lingnan Normal University, Zhanjiang
Email: 327284846@qq.com
Copyright © 2014 by authors and Hans Publishers Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received: Sep. 28th, 2014; revised: Oct. 23rd, 2014; accepted: Oct. 30th, 2014
ABSTRACT
In this paper, by using the related theory of matrix analysis, Schauder fixed point theorem and Banach fixed point theorem, also the related properties of the homeomorphism, the existence, uniqueness and stability of the continuously differentiable solution of perturbation Feigenbaum functional equation on high-dimensional space are researched.
Keywords:Feigenbaum Functional Equation, Schauder Fixed Point Theorem, Banach Fixed Point Theorem, Perturbation, Existence, Uniqueness, Stability

高维空间上扰动型Feigenbaum泛函方程的C1解
李 华,林日新,冷 薇,王 静,成嘉玲,张纾语
岭南师范学院数学与计算科学学院,湛江
Email: 327284846@qq.com
收稿日期:2014年9月28日;修回日期:2014年10月23日;录用日期:2014年10月30日

摘 要
本文利用矩阵分析的相关理论及Schauder不动点定理、Banach不动点定理及自同胚的相关性质研究了高维空间上扰动型Feigenbaum泛函方程的连续可微解的存在性、唯一性及稳定性。
关键词
Feigenbaum泛函方程,Schauder不动点定理,Banach不动点定理,扰动,存在性,唯一性,稳定性

1. 引言
在文献[1] -[7] 中,诸多学者研究了第二类型的 泛函方程
泛函方程
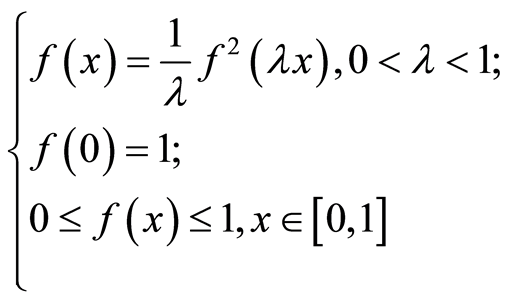 (1.1)
(1.1)
在区间![]() 上具有特定初值条件的渐近奇异解及单峰连续解,同时也讨论了
上具有特定初值条件的渐近奇异解及单峰连续解,同时也讨论了 在连续且逐段单调情形下的精确解。从动力系统的研究领域来看,对于这个方程的定性研究也是非常有意义的。考虑到这是个包含迭代的泛函方程,人们也更关注高维空间和抽象空间上它的非单调连续可微解。
在连续且逐段单调情形下的精确解。从动力系统的研究领域来看,对于这个方程的定性研究也是非常有意义的。考虑到这是个包含迭代的泛函方程,人们也更关注高维空间和抽象空间上它的非单调连续可微解。
文献[8] 研究了一维空间 上的
上的 型泛函方程的
型泛函方程的 解及
解及![]() 解,但所研究的方程,在迭代方程的形式上具有明显的规范性条件,诸多迭代方程的讨论也都具有此条件,如文献[9]
-[13] ,本文将去掉规范性条件,进而借用求解一阶线性微分方程的Cauchy积分思想,将带有边值问题的第二类型的
解,但所研究的方程,在迭代方程的形式上具有明显的规范性条件,诸多迭代方程的讨论也都具有此条件,如文献[9]
-[13] ,本文将去掉规范性条件,进而借用求解一阶线性微分方程的Cauchy积分思想,将带有边值问题的第二类型的 泛函方程转化为定性研究一个带有小扰动的
泛函方程转化为定性研究一个带有小扰动的 泛函方程,易见方程(1.1)在有关条件
泛函方程,易见方程(1.1)在有关条件
下与方程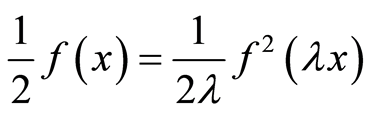 同解。
同解。
本文利用矩阵分析的相关理论,通过构造与文献[1] -[7] 不同的结构算子,利用![]() 不动点定理、自同胚和紧凸子集的相关性质,研究高维空间
不动点定理、自同胚和紧凸子集的相关性质,研究高维空间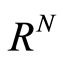 上一个紧凸子集
上一个紧凸子集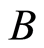 上无规范性系数条件的扰动型
上无规范性系数条件的扰动型 泛函方程
泛函方程
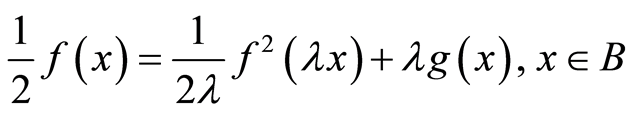 (1.2)
(1.2)
的连续非单调解的相关性质,其中![]() 和
和![]() 在紧凸子集
在紧凸子集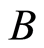 上是一个
上是一个![]() 类函数,
类函数, ,获得了方程(1.2)的非单调连续可微解的存在性、唯一性及稳定性。
,获得了方程(1.2)的非单调连续可微解的存在性、唯一性及稳定性。
2. 预备知识
假设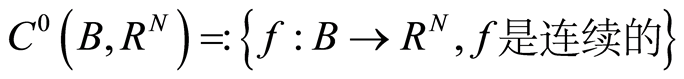 ,其中
,其中![]() 为
为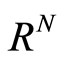 上的范数,
上的范数,![]() 为
为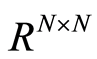 上的范数,那么明显的,
上的范数,那么明显的,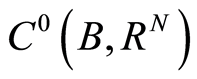 是关于范数
是关于范数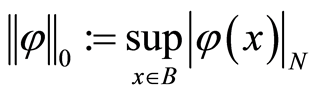 ,
, 构成一个
构成一个 空间。
空间。
假设 ,易证
,易证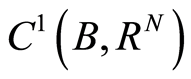 是一个关于范数
是一个关于范数![]() 是一个
是一个![]() 空间,其中
空间,其中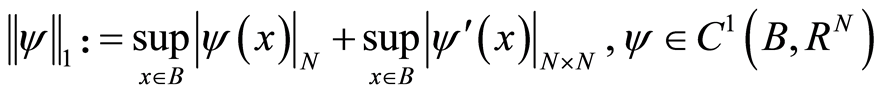 。其中
。其中![]() 是映射
是映射![]() 在
在 处的一个
处的一个![]() 矩阵。
矩阵。
作为 一个闭子集,
一个闭子集,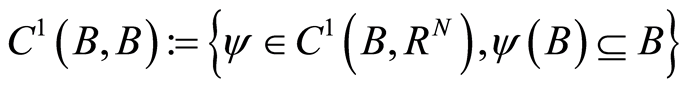 是完备的距离空间。因
是完备的距离空间。因 ,易见,若
,易见,若![]() ,则
,则![]() 。下面讨论中的
。下面讨论中的![]() 均为
均为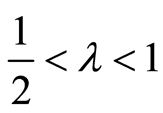 ,不再重述。
,不再重述。
下面的诸多引理是必需的,其证明方法与文献[11] 中的相类似,但是条件比文献[11] 中的要弱得多。
引理2.1:假设![]() 且
且
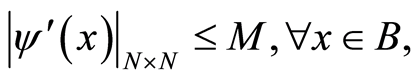 (2.1)
(2.1)
 (2.2)
(2.2)
其中 和
和![]() 是正数,则对于
是正数,则对于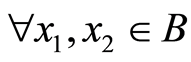 ,有
,有
 (2.3)
(2.3)
证明:
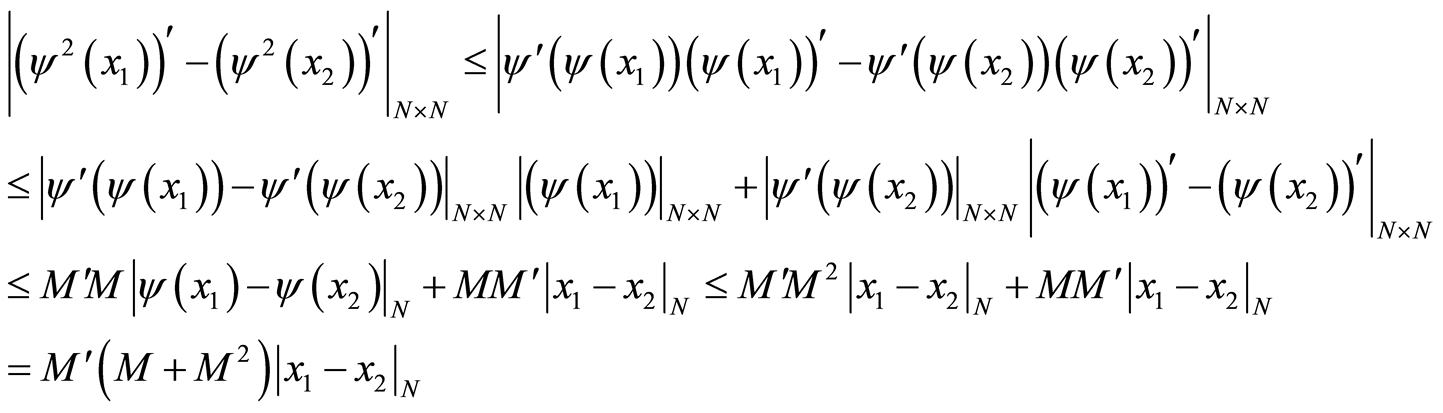
推论2.1:假设![]() 并且
并且
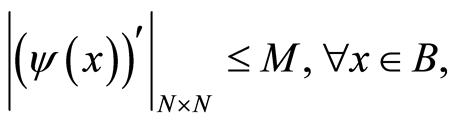 (2.4)
(2.4)
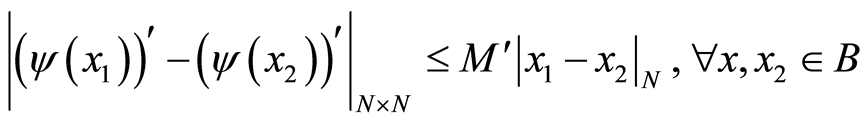 (2.5)
(2.5)
其中 和
和![]() 是正数。则对于
是正数。则对于 ,有
,有
 (2.6)
(2.6)
证明:对![]() ,由条件,我们有
,由条件,我们有

证毕。
引理2.2:假设![]() 并且
并且![]() 满足(2.1)。则
满足(2.1)。则
 (2.7)
(2.7)
证明:
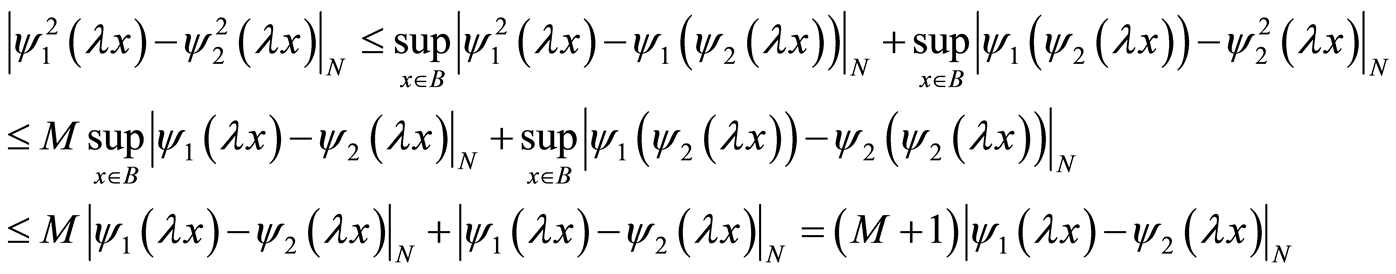
于是,(2.7)成立,证毕。
引理2.3:假设![]() 并且都满足(2.4)和(2.5)。则
并且都满足(2.4)和(2.5)。则
 (2.8)
(2.8)
证明:

所以(2.8)成立,证毕。
3. 主要结果
给定常数 ,假设
,假设
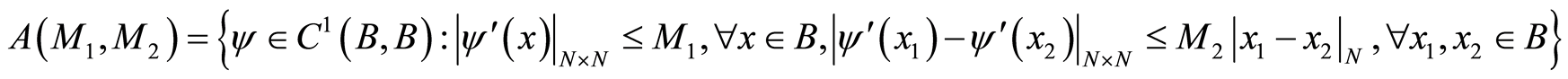
定理3.1(存在性) 给定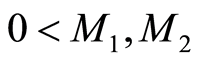 且
且![]() ,
, ,若存在正常数
,若存在正常数 满足
满足

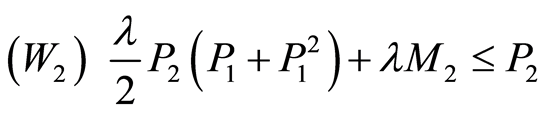
则方程(1.2)有一解![]() 。
。
证明:令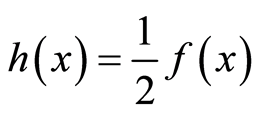 。构造如下的算子
。构造如下的算子![]() :
:

其中![]() ,由于对任意的
,由于对任意的![]() ,
,![]() 是连续可微的,则对任意的
是连续可微的,则对任意的![]() ,
,![]() 也是连续可微的,且
也是连续可微的,且![]() 。由条件
。由条件![]() 和
和![]() ,对于任意的
,对于任意的 ,则有
,则有
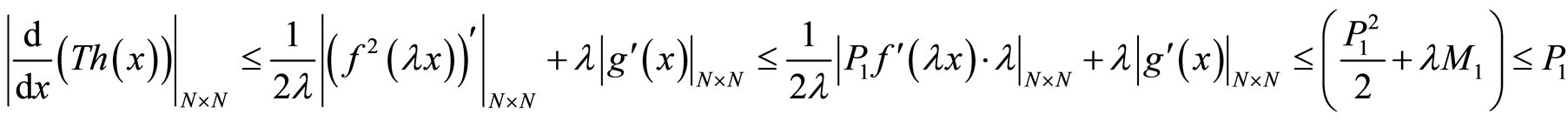
进一步,
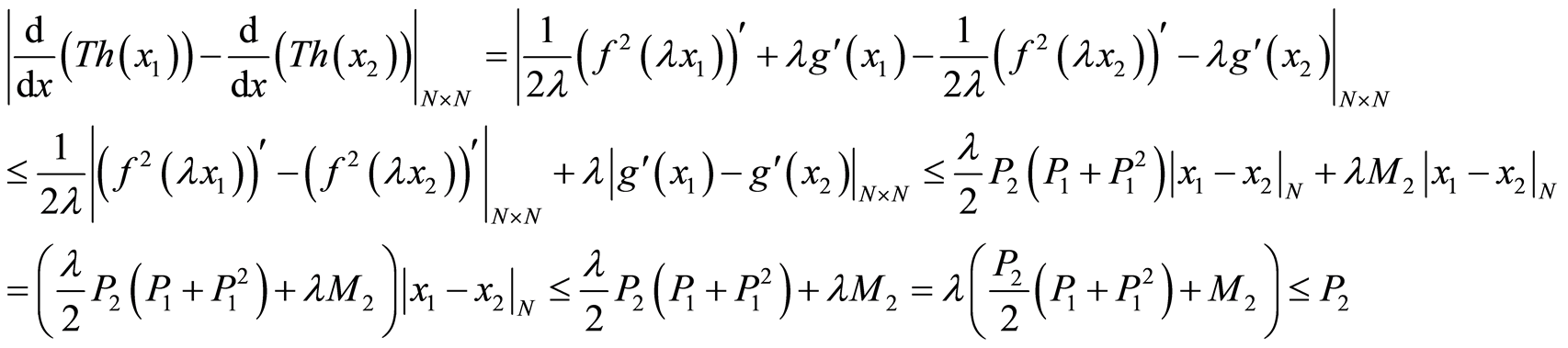
于是![]() ,即算子
,即算子 是
是![]() 上的一个自同胚映射。
上的一个自同胚映射。
下证算子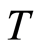 在范数
在范数![]() 下是连续的。对于任意的
下是连续的。对于任意的![]() ,则
,则![]() ,
,

其中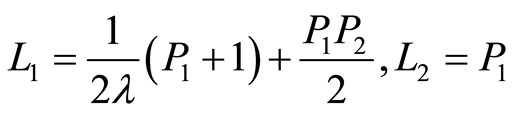 只要令
只要令![]() ,那么就有
,那么就有
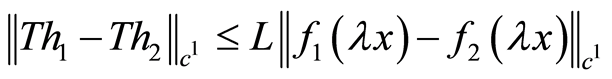 (3.1)
(3.1)
所以算子 是连续的。
是连续的。
下面证明![]() 是
是![]() 的一个紧凸子集。
的一个紧凸子集。
对任意的![]() 令
令![]() ,那么就有
,那么就有

对于任意的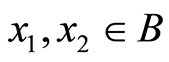 ,有
,有

所以,![]() 也就是
也就是![]() 是凸的并且也是紧的,因此对于任意的
是凸的并且也是紧的,因此对于任意的![]() ,故
,故![]() 是一致有界的。
是一致有界的。
对任给的![]() ,只要取
,只要取![]()
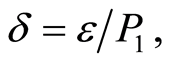 和
和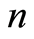 无关的,显然,对任意的整数
无关的,显然,对任意的整数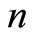 和
和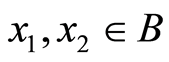
若![]() 时,则有
时,则有
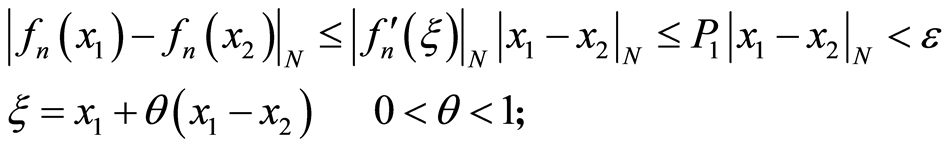
所以知道,![]() 是等度连续的。那么由
是等度连续的。那么由![]() 引理知道
引理知道![]() 是
是![]() 的紧凸子集;
的紧凸子集;
又由![]() 不动点定理,则存在一个函数
不动点定理,则存在一个函数![]() ,使得
,使得

因此方程(1.2)有一解![]() 。证毕。
。证毕。
定理3.2(唯一性) 假设条件![]() 和
和![]() 成立,同时假设条件
成立,同时假设条件![]() :
: ,则对任意的
,则对任意的![]() ,方程(1.2)有唯一解
,方程(1.2)有唯一解![]() 。
。
证明:由定理3.1可得到方程(1.2)解的存在性,并且![]() 是
是![]() 的一个闭集,由(3.1)和
的一个闭集,由(3.1)和![]() ,知
,知 ,我们得到算子
,我们得到算子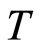 :
:![]() 是压缩的。因此由Banach不动点定理知算子T有唯一的不动点
是压缩的。因此由Banach不动点定理知算子T有唯一的不动点![]() ,即方程(1.2)有唯一解
,即方程(1.2)有唯一解![]() 。定理证毕。
。定理证毕。
定理3.3(稳定性) 设定理3.2中的条件成立,则在![]() 中方程(1.2)的解是连续依赖于给定的函数
中方程(1.2)的解是连续依赖于给定的函数![]() 。
。
证明:对于![]() ,由定理3.1及定理3.2我们可以得出分别存在唯一的函数
,由定理3.1及定理3.2我们可以得出分别存在唯一的函数![]() ,使得
,使得![]() 。
。
于是,由引理2.2和引理2.3,我们有

因为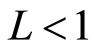 ,我们有
,我们有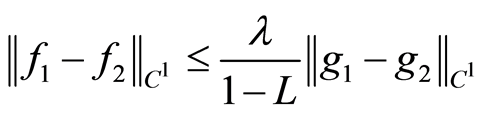 。故,方程(1.2)的解是连续依赖于给定的函数
。故,方程(1.2)的解是连续依赖于给定的函数![]() ,即方程的解是稳定的。证毕。
,即方程的解是稳定的。证毕。
4. 例子
令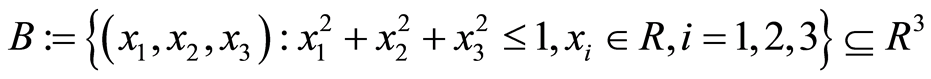 ,其中
,其中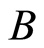 是
是 的一个紧凸子集,那么取定
的一个紧凸子集,那么取定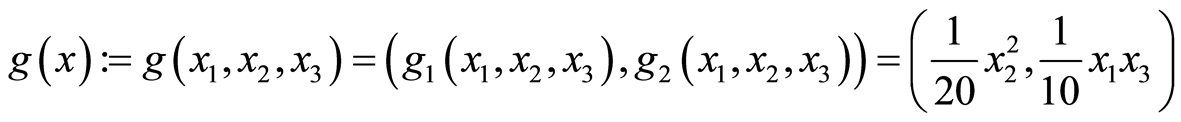 ,而
,而![]()
考虑方程
 (4.1)
(4.1)
取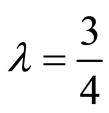 ,显然
,显然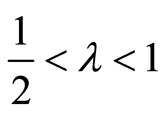 ,
,
因为:
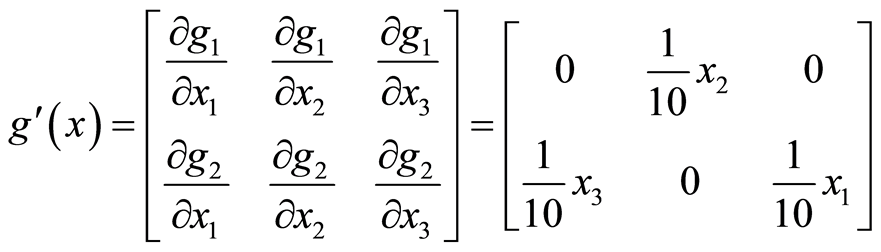
那么,
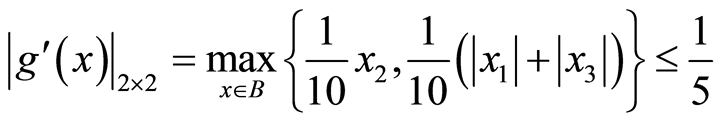
对于任意的 ,令
,令![]() ,有
,有
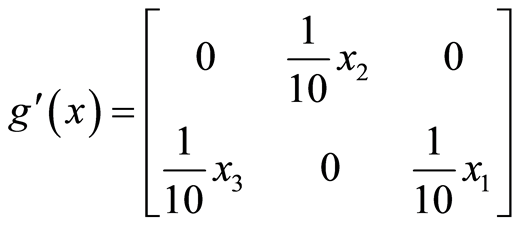
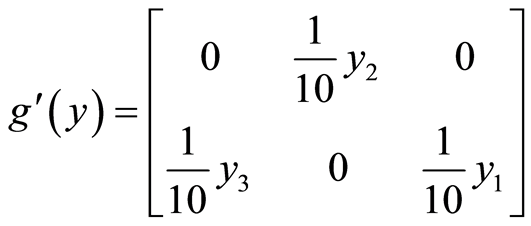
所以:

即: 。
。
由条件![]() 等价于不等式:
等价于不等式: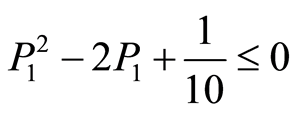 ,其中,那么可得,
,其中,那么可得, 。选取
。选取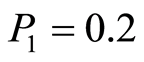 ,又由条件
,又由条件![]() 等价于不等式:
等价于不等式: 。
。
所以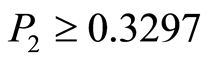 。取
。取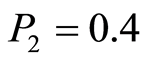 。由定理(3.1) (3.2) (3.3)知,方程(4.1)在
。由定理(3.1) (3.2) (3.3)知,方程(4.1)在![]() 中存在唯一个连续可微解,且连续依赖于
中存在唯一个连续可微解,且连续依赖于![]() 。
。
基金项目
广东省大学生科技创新重点培育项目;岭南师范学院2014年度大学生创新创业训练计划项目;全国大学生创新训练项目,编号:201410579006。
参考文献 (References)
- [1] Lanford, O.E. (1987) A computer assisted proof of the Feigenbaum conjectures. Bulletin of the American Mathematical Society, 6, 427-434.
- [2] Eokmann, J.-P. and Wittwer, P. (1987) A computer assisted proof of the Feigenbaum conjectures. Journal of Statistical Physics, 46, 455-477.
- [3] Epstein, H. (1986) New proofs of the existence of the Feigenbaum function. Communications in Mathematical Physics, 106, 395-426.
- [4] Thompson, C.J. and McGuire, J.B. (1982) Asymptotic and essentially singular solution of the Feigenbaum equation. Journal of Statistical Physics, 27, 183-200.
- [5] Mestel, B.D., Osbaldestin, A.H. and Tstgvintsev, A.V. (2002) Continued fractions and solutions of the FeigenbaumCvitanovie equation. C R Acad Sci Paris, 334, 683-688.
- [6] McCarthy, P.J. (1983) The general exact bijective continuous solution of Feigenbaum’s functional equation. Communications in Mathematical Physics, 91, 431-443.
- [7] Zhang, J.Z. and Yang, L. (1983) Discussion on iterative roots of continuous and piecewise monotone functions. Acta mathematica Sinica, Chinese, 26, 398-412.
- [8] Lin, X.M., Huang, S.M. and Huang, H.M. (2011) On the C1 solution of the Feigenbaum type functional equation. Journal of Zhanjiang Normal College, 3.
- [9] Zhang, W.N. (1987) Discussion on the iterated equation
 . Chinese Science Bulletin, 32, 1441-1451.
. Chinese Science Bulletin, 32, 1441-1451. - [10] Zhang, W.N. (1988) Stability of the solution of the iterated equation
 . Acta Mathematica Sinica, 18, 421-424.
. Acta Mathematica Sinica, 18, 421-424. - [11] Zhang, W.N. (1998) Discussion on the differentiable solutions of
the iterated equation
 . Nonlinear Analysis: Theory, Methods and Applications,
15, 387-398.
. Nonlinear Analysis: Theory, Methods and Applications,
15, 387-398. - [12] Zhang, W.N. (2000) Solutions of equivariance for a polynomial-like iterated equation. Proceedings of the Royal Society of Edinburgh, Section A, 130, 1153-1163.
- [13] Li, X.P. (2004) A class of iterative equation on a Banach space. Journal of Sichuan University (N. SCI. E.), 41, 505-510.