Pure Mathematics
Vol.05 No.04(2015), Article ID:15679,6
pages
10.12677/PM.2015.54022
Existence of Bounded Solutions to a Class of Nonlinear Elliptic Problems with General Growth in the Gradient
Yujuan Tian1, Chao Ma2
1School of Mathematical Sciences, Shandong Normal University, Jinan Shandong
2School of Mathematical Sciences, University of Jinan, Jinan Shandong
Email: tianyujuan0302@126.com
Received: Jun. 30th, 2015; accepted: Jul. 10th, 2015; published: Jul. 16th, 2015
Copyright © 2015 by authors and Hans Publishers Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



ABSTRACT
This paper studies a class of nonlinear elliptic equations with  growth in the gradient. By discussing the fixed point for a class of Volterra integral operator, we use symmetric technique to prove the existence of bounded solutions.
growth in the gradient. By discussing the fixed point for a class of Volterra integral operator, we use symmetric technique to prove the existence of bounded solutions.
Keywords:Nonlinear Elliptic Equation, Gradient Term, Bounded Solution, Symmetric Technique

关于梯度具有一般增长的非线性椭圆问题 有界解的存在性
田玉娟1,马超2
1山东师范大学数学科学学院,山东 济南
2济南大学数学科学学院,山东 济南
Email: tianyujuan0302@126.com
收稿日期:2015年6月30日;录用日期:2015年7月10日;发布日期:2015年7月16日

摘 要
本文研究了一类关于梯度具有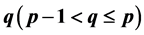 增长的非线性椭圆方程。通过对一类Volterra型积分算子不动点的讨论,我们应用对称技术证明了有界解的存在性。
增长的非线性椭圆方程。通过对一类Volterra型积分算子不动点的讨论,我们应用对称技术证明了有界解的存在性。
关键词 :非线性椭圆方程,梯度项,有界解,对称技术

1. 引言
本文研究如下非线性椭圆方程Dirichlet问题有界弱解的存在性:
 (1.1)
(1.1)
其中 是
是 中的有界区域,非线性项
中的有界区域,非线性项 关于梯度具有
关于梯度具有 增长,即
增长,即 。
。
对于 的情形,问题(1.1)解的存在性已被众多学者通过各种方法在附加结构条件的情况下进行了研究。例如,若
的情形,问题(1.1)解的存在性已被众多学者通过各种方法在附加结构条件的情况下进行了研究。例如,若 满足符号条件,Bensoussan等[1] 讨论了弱解的存在性;Boccardo等[2] 在方程存在上下解的前提下证明了有界解的存在性;而在具有正零阶项的结构条件下,文献[3] [4] 得到了类似结论。近些年,对于没有附加结构条件的问题(1.1),有界解的存在性也得到了广泛研究。这些研究基于对称技术进行讨论,利用Schwarz对称建立原方程与对称方程解之间的比较结果,进而获得解的先验估计,以此证明存在性。文献[5] -[8] 考虑了
满足符号条件,Bensoussan等[1] 讨论了弱解的存在性;Boccardo等[2] 在方程存在上下解的前提下证明了有界解的存在性;而在具有正零阶项的结构条件下,文献[3] [4] 得到了类似结论。近些年,对于没有附加结构条件的问题(1.1),有界解的存在性也得到了广泛研究。这些研究基于对称技术进行讨论,利用Schwarz对称建立原方程与对称方程解之间的比较结果,进而获得解的先验估计,以此证明存在性。文献[5] -[8] 考虑了 的情形。之后,Ferone和Messano在[9] 中结合特殊不等式将结论推广至
的情形。之后,Ferone和Messano在[9] 中结合特殊不等式将结论推广至 的情形,然而其证明需要
的情形,然而其证明需要 ,该条件对于存在有界解来说较强。
,该条件对于存在有界解来说较强。
因此,本文的工作主要是研究在一般的假设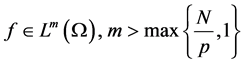 下,问题(1.1)有界解的存在
下,问题(1.1)有界解的存在
性。通过对一类Volterra型积分算子不动点的研究,获得了问题(1.1)与一类对称问题解之间的比较结果,进而证得结论。本文的安排如下:第二节将给出一些预备知识,第三节给出假设与主要结果,第四节给出主要结果的证明。
2. 预备知识
为方便起见,我们记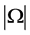 为区域
为区域 的Lebesgue测度,
的Lebesgue测度, ,
, ,以后不再说明。
,以后不再说明。
定义2.1:设 是
是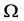 上的可测函数,
上的可测函数, 是
是 的分布函数,称
的分布函数,称 ,
, 为函数
为函数 的单调递减重排。
的单调递减重排。
令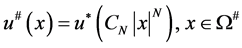 ,称
,称 为函数
为函数 的单调递减球对称重排,也称为Schwarz对称。这里
的单调递减球对称重排,也称为Schwarz对称。这里 表示单位球体积,
表示单位球体积, 表示球心位于原点且与
表示球心位于原点且与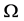 具有相同测度的球。
具有相同测度的球。
注2.1 [10] :Schwarz对称保持函数的 范数不变,即
范数不变,即 。
。
设 为Carathéodory函数,
为Carathéodory函数,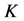 为如下Volterra型积分算子 [11] :
为如下Volterra型积分算子 [11] :
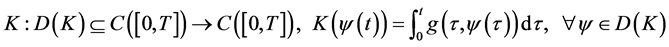 (2.1)
(2.1)
定义2.2:设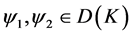 ,
, ,若存在三常数
,若存在三常数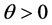 ,
, 和
和 使得对任意
使得对任意 ,都有
,都有

则称算子 具有性质(l)。
具有性质(l)。
引理2.1 (比较原理):设 为由(2.1)给出的Volterra型积分算子,且具有性质(l)。若对
为由(2.1)给出的Volterra型积分算子,且具有性质(l)。若对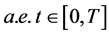 ,
, 为非减函数,则对任意
为非减函数,则对任意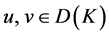 满足
满足 ,
, ,都有
,都有
 (2.2)
(2.2)
证明:采用反证法。若(2.2)不成立,则必存在 和
和 使得
使得 ,且
,且
 。记
。记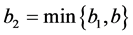 ,其中
,其中 是性质(l)中的常数。对
是性质(l)中的常数。对
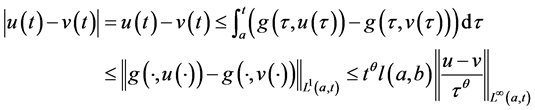
上式两端关于 取最大值且注意
取最大值且注意 ,得
,得
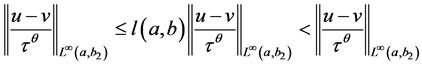
产生矛盾。所以假设不成立,(2.2)得证。
3. 假设与主要结果
本文研究非线性椭圆问题(1.1),其中 ,
, 为Carathéodory函数且对
为Carathéodory函数且对 及
及 满足以下假设条件:
满足以下假设条件:
(i) ,其中
,其中 ;
;
(ii) ,其中
,其中 为常数,
为常数, 且
且 ;
;
(iii) ,其中
,其中 为常数,
为常数, ,
,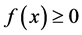 且
且 。
。
定义3.1:函数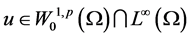 称为问题(1.1)的弱解,如果以下等式成立:
称为问题(1.1)的弱解,如果以下等式成立:

首先,考虑(1.1)的对称问题
 (3.1)
(3.1)
其中 为
为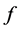 的Schwarz对称。讨论该对称问题对称解存在的条件,定理如下。
的Schwarz对称。讨论该对称问题对称解存在的条件,定理如下。
定理3.1设 ,其中
,其中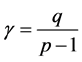 ,
, ,
,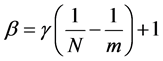 。
。
若
 (3.2)
(3.2)
则问题(3.1)必存在对称解 ,即
,即 。且有如下估计:
。且有如下估计:

这里 、
、 是仅与已知量
是仅与已知量 有关的常数。
有关的常数。
其次,给出问题(1.1)的解与问题(3.1)的对称解之间的比较结果。
定理3.2:假设(i)~(iii)成立。若 是问题(1.1)的解,
是问题(1.1)的解, 是问题(3.1)的对称解,则
是问题(3.1)的对称解,则
 (3.3)
(3.3)
 (3.4)
(3.4)
最后,得到问题(1.1)有界弱解的存在性。
定理3.3:假设(i)~(iii)和(3.2)成立。若

则问题(1.1)至少存在一个解。
4. 主要结果的证明
对于Volterra型积分算子(2.1),更具体地,我们令 ,
,

则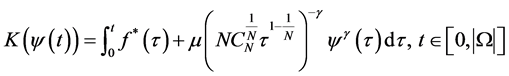 。记
。记 是
是 的值域。
的值域。
引理4.1:设(3.2)成立,在 中令
中令 ,则算子
,则算子 在
在 中必有不动点。
中必有不动点。
证明:首先可证 。事实上,对于
。事实上,对于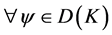 及
及 ,由Hölder不等式得
,由Hölder不等式得
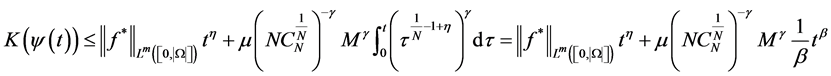
由 的具体形式以及
的具体形式以及 容易计算得
容易计算得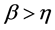 ,故
,故
 ,即
,即
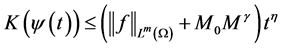 . (4.1)
. (4.1)
又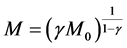 ,由(3.2)可知
,由(3.2)可知 。所以
。所以 ,说明
,说明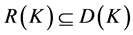 。
。
其次,因 ,故
,故 。从而,对任意
。从而,对任意
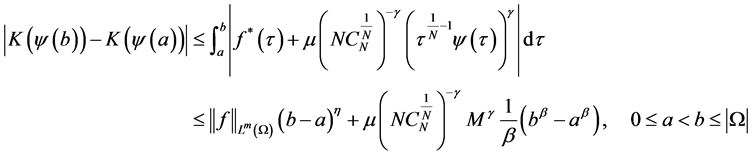
因此当 时,
时,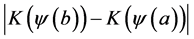 一致地收敛于0,故
一致地收敛于0,故 等度连续。此外,由(4.1)得
等度连续。此外,由(4.1)得
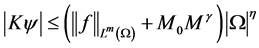 ,从而
,从而 是一致有界的。于是由Ascoli-Arzela定理知
是一致有界的。于是由Ascoli-Arzela定理知 是
是 中的列紧集,即
中的列紧集,即 是
是 中的紧算子。注意到
中的紧算子。注意到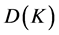 是有界闭凸集,
是有界闭凸集, 且
且 是紧算子。
是紧算子。
根据Schauder不动点定理得, 在
在 中必有一个不动点。
中必有一个不动点。
引理4.2 [9] 设 是问题(1.1)的解,
是问题(1.1)的解,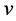 是问题(3.1)的对称解,则对
是问题(3.1)的对称解,则对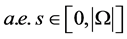 ,有
,有
 (4.2)
(4.2)
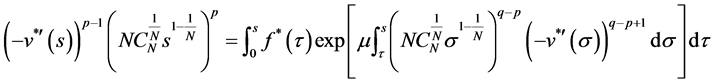 (4.3)
(4.3)
定理3.1的证明:令
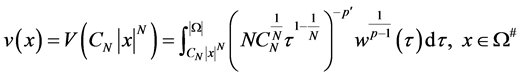 (4.4)
(4.4)
其中 为引理4.1中
为引理4.1中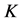 的不动点。显然
的不动点。显然 且
且 。
。
又
注意到 ,故
,故

而
于是, 。此外,易验证
。此外,易验证 满足问题(3.1)弱解的定义。从而(4.4)给出的正是问题(3.1)的一个对称解。
满足问题(3.1)弱解的定义。从而(4.4)给出的正是问题(3.1)的一个对称解。
定理3.2的证明:令
 (4.5)
(4.5)
等式两边关于s求导,得
 (4.6)
(4.6)
由(4.2)知 ,带入(4.6)中有
,带入(4.6)中有

上式在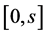 上积分,并注意
上积分,并注意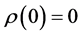 ,可得
,可得 。同理,若令
。同理,若令
 (4.7)
(4.7)
则有以下等式成立: 。
。
由(4.5)和(4.7)计算可得 。又对
。又对 ,任取
,任取 ,
,

注意到 ,所以
,所以

令 ,则
,则 ,从而算子
,从而算子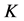 具有性质(l)。
具有性质(l)。
于是由引理2.1可知,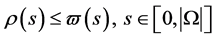 。
。
上式结合(4.2)和(4.3)可得 ,
, ,即
,即

注意到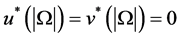 ,因此
,因此
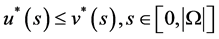 ,即
,即
此外,(3.4)的证明完全类似于文献[9] 中定理4.1的证明,这里不再赘述。
定理3.3的证明:
对于 ,考虑以下逼近问题
,考虑以下逼近问题
 (4.8)
(4.8)
其中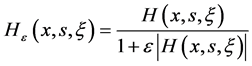 ,则
,则 满足条件(iii)且
满足条件(iii)且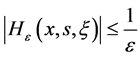 。
。
从而问题(4.8)存在解 。又由定理3.1知问题(3.1)存在对称解
。又由定理3.1知问题(3.1)存在对称解 且有估计
且有估计 和
和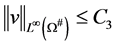 。
。
因此,根据定理3.2有: 且
且 。于是
。于是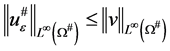 。注意到
。注意到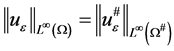 ,所以
,所以 。从而
。从而
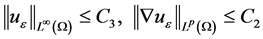
最后,利用文献[3] [4] 中经典的逼近理论,可证问题(1.1)至少存在一个解。
基金项目
山东省中青年科学家科研奖励基金(BS2012SF026);数学天元青年基金(11426146);济南大学博士基金(XBS1337)。
文章引用
田玉娟,马 超, (2015) 关于梯度具有一般增长的非线性椭圆问题有界解的存在性
Existence of Bounded Solutions to a Class of Nonlinear Elliptic Problems with General Growth in the Gradient. 理论数学,04,143-149. doi: 10.12677/PM.2015.54022
参考文献 (References)
- 1. Bensoussan, A., Baccordo, L. and Murat, F. (1988) On a nonlinear partial differential equation having natural growth terms and unbounded solution. Annales de l’institut Henri Poincaré (C) Analyse Non Linéaire, 5, 347-364.
- 2. Baccordo, L., Murat, F. and Puel, J.P. (1984) Résultats d'existence pour certains problèmes elliptiques quasilinéaires. Annali Della Scuola Normale Superiore Di Pisa-Classe Di Scienze, 11, 213-285.
- 3. Baccordo, L., Murat, F. and Puel, J.P. (1988) Existence of bounded solution for nonlinear elliptic unilateral problems, Annali di Ma-tematica Pura ed Applicata, 152, 183-196. http://dx.doi.org/10.1007/BF01766148
- 4. Baccordo, L., Murat, F. and Puel, J.P. (1992) estimate for some nonlinear elliptic partial differential equation and application to an existence result. SIAM Journal on Mathematical Analysis, 23, 326-333. http://dx.doi.org/10.1137/0523016
- 5. Ferone, V., Posteraro, M.R. and Rakotoson, J.M. (1999) -estimate for nonlinear elliptic problems with p-growth in the gradient. Journal of Inequalities and Applications, 3, 109-125. http://dx.doi.org/10.1155/s1025583499000077
- 6. Grenon, N. (2001) Existence and comparison results for qua-silinear elliptic equations with critical growth in the gradient. Journal of Differential Equations, 171, 1-23. http://dx.doi.org/10.1006/jdeq.2000.3833
- 7. Grenon, N. and Trombetti, C. (2003) Existence results for a class of nonlinear elliptic problems with p-growth in the gradient. Nonlinear Analysis: Theory, Methods & Applications, 52, 931-942. http://dx.doi.org/10.1016/S0362-546X(02)00143-8
- 8. Messano, B. (2006) Symmetrization results for classes of nonlinear elliptic equations with q-growth in the gradient. Nonlinear Analysis: Theory, Methods & Applications, 64, 2688-2703. http://dx.doi.org/10.1016/j.na.2005.07.042
- 9. Ferone, V. and Messano, B. (2007) Comparison and existence results for classes of nonlinear elliptic equations with general growth in the gradient. Advanced Nonlinear Studies, 7, 31-46.
- 10. Kawohl, B. (1985) Rearrangement and convexity of level sets in PDE. Lecture Notes in Math., 1150, Springer-Verlag, Berlin.
- 11. Pašić, M. (1996) Isoperimetric inequalities in quasilinear elliptic equations of Le-ray-Lions type. Journal de Mathématiques Pures et Appliqués, 75, 343-366.