Advances in Applied Mathematics
Vol.
11
No.
10
(
2022
), Article ID:
57082
,
9
pages
10.12677/AAM.2022.1110778
一类3-缠绕的Jones多项式
杨晓雨
辽宁师范大学,辽宁 大连
收稿日期:2022年9月21日;录用日期:2022年10月14日;发布日期:2022年10月25日

摘要
选定了3-缠绕的一种定向方式,结合Giller的房间理论给出任意两个3-缠绕的复合的Jones多项式。接着,通过研究计算得到了一类特殊3-缠绕的Jones多项式的递推公式以及由其闭包所形成的链环的Jones多项式。
关键词
Jones多项式,不变量,3-缠绕

The Jones Polynomials of a Class of 3-Tangles
Xiaoyu Yang
Liaoning Normal University, Dalian Liaoning
Received: Sep. 21st, 2022; accepted: Oct. 14th, 2022; published: Oct. 25th, 2022

ABSTRACT
An unusual orientation of 3-tangles is given, and the Jones polynomial of the concatenation of two 3-tangles is given by Giller’s room theory. Then, the recursion formula of the Jones polynomials of a special kind of 3-tangles is obtained by studying and calculating. In addition, the formula to obtain the Jones polynomial of the links obtained from their closure is given.
Keywords:Jones Polynomial, Invariant, 3-Tangle

Copyright © 2022 by author(s) and Hans Publishers Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY 4.0).
http://creativecommons.org/licenses/by/4.0/


1. 引言
纽结理论研究纽结和链环在连续形变下保持不变的特性,是拓扑学中引人入胜的一支。等价分类问题是纽结理论的中心,人们通过寻找纽结不变量来解决纽结的等价问题。纽结多项式 [1] [2] [3] [4] 是常见的纽结不变量之一,而Jones多项式是重要的纽结多项式,它为研究纽结与链环的手征性提供了有力的工具。
近几年,不同定向的3-缠绕的多项式成为了数学界的一个关注点且有了大量的研究成果 [5] - [10]。在文献 [6] 中,Cabrera给出了3-缠绕的一种定向方式,在此基础上研究了5种不同的方法闭合3-缠绕得到纽结或链环,给出了公式计算两个3-缠绕的复合的闭包的Conway多项式。文献 [9] 给出了3-缠绕的一种常规定向,也即3-辫子,并在此基础上研究了3-辫子的复合的六种不同闭包方法得到的链环的Conway多项式。文献 [10] 给出了3-缠绕不同于文献 [9] 的新的定向,并利用Giller的房间理论计算了它们的Conway多项式和Alexander多项式。
本文在前人的研究基础上,利用Giller的房间理论进一步研究了一类特殊3-缠绕 的Jones多项式。在预备知识我们将介绍一些有关3-缠绕和纽结Jones多项式的一些基本概念;在第二部分,我们将通过Giller的房间理论给出任意两个3-缠绕的复合的Jones多项式;第三部分,通过研究计算得到一类特殊的3-缠绕 的Jones多项式的递推公式,并进一步计算由其闭包所形成的链环的Jones多项式。
2. 预备知识
2.1. 3-缠绕的基本概念
定义2.1 [10] n-缠绕指一个偶对 ,其中 是一个三维实心球, 是一个具有非空边界的一维嵌入子流形,它包含n个弧(即n个同胚于 的子集),并满足 。
本文只讨论3-缠绕,且记为T而不是 。
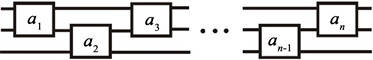
定义2.2 [11] n-辫子是由n条线组成的集合,这些线都连接在顶部和底部的水平线上(如图1),沿着任意一根线从顶部移动到底部时方向总是向下的,即每根线与两根水平线之间的任何水平面相交且只相交一次。n-辫子是n-缠绕的一种特殊情况。

Figure 1. A braid
图1. 辫子
 (a)
(a)
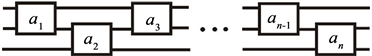 (b)
(b)
Figure 2. 3-braid with (a) n odd and (b) n even
图2. 3-辫子 其中(a) n为奇数(b) n为偶数
本文研究的是3-辫子,并使用符号 [10] 表示,其中 均为整数, 。如图2所示, 表示交叉点的类型和数量,规定:若 ,则3-辫子无交叉点,否则

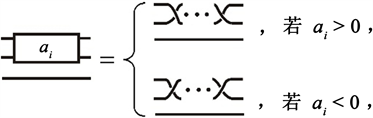
定义2.3 [10] 如图3所示3-辫子 是一个半扭转,记为 。

Figure 3. 3-braid
图3. 3-辫子
定义2.4 [12] 房间R是R2中的一个连通域,它具有相同数量的有向的进出绳。本文讨论的是如图4所示的3-房间。

Figure 4. The 3-room R
图4. 3-房间R
定义2.5 [12] 所有连通房间进出绳的集合,用 表示。 的一个元素称为R的居住者。(图5)
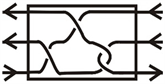
Figure 5. An inhabitant of the 3-room R
图5. 3-房间R的一个居住者
事实上,带有由进出绳诱导的方向的R的居住者即为一个有向3-缠绕。
定义2.6 [12] 在 中通过连接的方式定义一个记为“ ”的运算(如图6),将构造一些链环。

Figure 6. Inhabitants S and T in and their concatenation
图6. 中的居住者S和T以及它们的复合
2.2. 纽结的Jones多项式
有向链环定义的一个多项式不变量是Jones多项式 。这个多项式可以通过以下拆接关系 [11] 来计算:
1) ,
2) V( ) = 1,
) = 1,
其中是 平凡纽结,
是三个只在一个交叉点处不同的链环。(图7)
平凡纽结,
是三个只在一个交叉点处不同的链环。(图7)
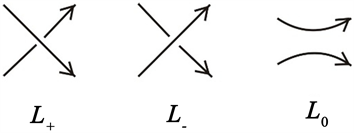
Figure 7. Skein triple
图7. 拆接关系
引理2.1 [13] 若L是具有c个分支的平凡链环,则 ,其中 。
引理2.2 [14] 设L为任意有向链环,则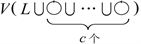 =
,其中
。
=
,其中
。
3. 3-缠绕的Jones多项式
在 中有6种不同的方式连接R的进绳和出绳。图8是连接R的进出绳后具有最少交叉点数的居住者,用 表示,其中 。这样的居住者的集合 称为 的基居住者集 [10]。

Figure 8. Basic inhabitants of
图8. 的基居住者
设F是 的分式域, 表示 在F上生成的自由向量空间, 是 在F上生成的子空间,其中 是一个拆接关系。记 是商向量空间 。特别地,图8所示的基居住者是 的基。因此, 中的每个元素都可以唯一地表示成基居住者的线性组合。
对任意的 ,通过对图T反复应用Jones多项式的拆接关系,直到只剩下基居住者为止,就得到了T的Jones多项式。因此
,其中 。
定理3.1设 是两个3-缠绕,且
,,
那么
证明:对 中的 应用Jones多项式的拆接公式,我们有
计算 ,并将多项式化简后即可得结果。下面我们计算 的情况,其他情况可类似计算。(表1)
Table1.
表1.
4. 3-缠绕 以及链环 的Jones多项式
4.1. 3-缠绕 的Jones多项式
定理4.1记 ,则对任意的 ,有 ,其中
证明:通过对k作归纳法证明该定理。 时, ,则有 。此时 ,。 时,经过计算可以得到
此时 ,,,,。
现在假设 时定理4.1成立。对于k时,应用定理3.1,我们有
将 的值代入后,有
此时
定理4.1得证。
4.2. 链环 的Jones多项式
利用3-缠绕可以构造一些链环。设T是一个3-缠绕,如图9所示方式闭合3-缠绕可以得到纽结或链环。记为 。
定理4.2设T是一个3-缠绕且 ,那么
。
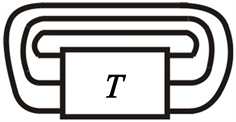
Figure 9. Link
图9. 链环
证明:由 ,有 。如图10,我们可以看到, 时, 是分支数为3的平凡链环; 时, 是分支数为2的平凡链环; 时, 为平凡结。由引理2.1有 ,,。此外,对 应用拆接关系有
( )
(
)
( )
(
)
( ),
),
得到 。因此,
。
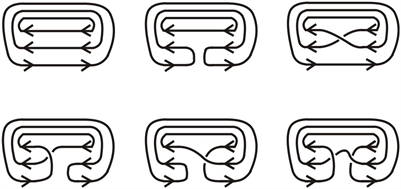
Figure 10. Link
图10. 链环
定理4.3链环 的Jones多项式为
。
证明:由定理4.1有 。再由定理4.2可得
。
5. 结语
本文主要讨论了一类3-缠绕的Jones多项式。通过Giller的房间理论给出两个3-缠绕的复合的Jones多项式。其次,给出一类特殊3-缠绕的Jones多项式计算公式,并在此基础上计算了3-缠绕闭包方法得到的链环的Jones多项式。
文章引用
杨晓雨. 一类3-缠绕的Jones多项式
The Jones Polynomials of a Class of 3-Tangles[J]. 应用数学进展, 2022, 11(10): 7325-7333. https://doi.org/10.12677/AAM.2022.1110778
参考文献
- 1. Alexander, J.W. (1928) Topological Invariants of Knots and Links. Transactions of the American Mathematical Society, 30, 275-306. https://doi.org/10.1090/S0002-9947-1928-1501429-1
- 2. Conway, J.H. (1970) An Enumeration of Knots and Links and Some of Their Algebraic Properties. Computational Problems in Abstract Algerbra, 22, 329-358. https://doi.org/10.1016/B978-0-08-012975-4.50034-5
- 3. Jones, V.F.R. (1985) A Polynomial Invariants for knots via von Neumann Algebras. Bulletin of the American Mathematical Society, 12, 103-111. https://doi.org/10.1090/S0273-0979-1985-15304-2
- 4. Freyd, P., Yetter, D., Hoste, J., et al. (1985) A New Pol-ynomial Invariant of Knots and Links. Bulletin of the American Mathematical Society, 12, 239-246. https://doi.org/10.1090/S0273-0979-1985-15361-3
- 5. Cabrera-Ibarra, H. (2003) On the Classification of Rational 3-Tangles. Journal of Knot Theory and Its Ramifications, 12, 921-946. https://doi.org/10.1142/S021821650300286X
- 6. Cabrera-Ibarra, H. (2004) Conway Polynomial of the Closures of Oriented 3-String Tangles. Boletín de la Sociedad Matemática Mexicana, 10, 55-62.
- 7. Cabrera-Ibarra, H. (2004) Results on the Classification of Rational 3-Tangles. Journal of Knot Theory and Its Ramifications, 13, 175-192. https://doi.org/10.1142/S021821650400307X
- 8. Cabrera-Ibarra, H. and Lizárraga, D.A.L. (2010) An Algorithm Based on 3-Braids to Solve Tangle Equations Arising in the Action of Gin DNA Invertase. Applied Mathematics and Computation, 216, 95-106. https://doi.org/10.1016/j.amc.2010.01.007
- 9. Lizárraga, D.A., Hernández, L.Y. and Cabrera-Ibarra, H. (2012) Computing the Conway Polynomial of Several Closures of Oriented 3-Braids. Topology and Its Applications, 159, 1195-1209. https://doi.org/10.1016/j.topol.2011.11.032
- 10. de los Angeles Guevara Hernández, M. and Cabre-ra-Ibarra, H. (2019) Infinite Families of Prime Knots with alt(K) = 1 and Their Alexander Polynomials. Journal of Knot Theory and Its Ramifications, 28, Article ID: 1950010. https://doi.org/10.1142/S021821651950010X
- 11. Adams, C. (1994) The Knot Book. W. H. Freeman and Com-pany, New York.
- 12. Giller, C.A. (1982) A Family of Links and the Conway Calculus. Transactions of the American Mathematical Society, 270, 75-109. https://doi.org/10.1090/S0002-9947-1982-0642331-X
- 13. 姜伯驹. 绳圈的数学[M]. 大连: 大连理工大学出版社, 2011.
- 14. Murasugi, K. (2007) Knot Theory and Its Applications. Springer Science & Business Media, Berlin. https://doi.org/10.1007/978-0-8176-4719-3